Hostname: page-component-586b7cd67f-2brh9 Total loading time: 0 Render date: 2024-12-02T22:02:49.431Z Has data issue: false hasContentIssue false
  • English
  • Français

Subcritical convection of liquid metals in a rotating sphere using a quasi-geostrophic model

Published online by Cambridge University Press:  26 October 2016

Céline Guervilly  and
Philippe Cardin
Céline Guervilly*
Affiliation:
School of Mathematics and Statistics, Newcastle University, Newcastle upon Tyne NE1 7RU, UK
Philippe Cardin
Affiliation:
Institut des Sciences de la Terre, Université Grenoble Alpes, CNRS, 38041 Grenoble, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

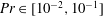We study nonlinear convection in a rapidly rotating sphere with internal heating for values of the Prandtl number relevant for liquid metals ($Pr\in [10^{-2},10^{-1}]$). We use a numerical model based on the quasi-geostrophic approximation, in which variations of the axial vorticity along the rotation axis are neglected, whereas the temperature field is fully three-dimensional. We identify two separate branches of convection close to onset: (i) a well-known weak branch for Ekman numbers greater than 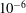$10^{-6}$, which is continuous at the onset (supercritical bifurcation) and consists of thermal Rossby waves and (ii) a novel strong branch at lower Ekman numbers, which is discontinuous at the onset. The strong branch becomes subcritical for Ekman numbers of the order of 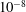$10^{-8}$. On the strong branch, the Reynolds number of the flow is greater than 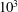$10^{3}$, and a strong zonal flow with multiple jets develops, even close to the nonlinear onset of convection. We find that the subcriticality is amplified by decreasing the Prandtl number. The two branches can co-exist for intermediate Ekman numbers, leading to hysteresis (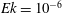$Ek=10^{-6}$, 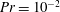$Pr=10^{-2}$). Nonlinear oscillations are observed near the onset of convection for $Ek=10^{-7}$ and $Pr=10^{-1}$.

JFM classification

Geophysical and Geological Flows: Quasi-geostrophic flows Geophysical and Geological Flows: Rotating flows Turbulent Flows: Turbulent convection
Type
Papers
Information
Journal of Fluid Mechanics , Volume 808 , 10 December 2016 , pp. 61 - 89
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ardes, M., Busse, F. H. & Wicht, J. 1997 Thermal convection in rotating spherical shells. Phys. Earth Planet. Inter. 99, 55–67.Google Scholar
Aubert, J.2001 Modèles expérimentaux et numériques de la convection dans le noyau terrestre. PhD thesis, Université Joseph Fourier Grenoble.Google Scholar
Aubert, J., Gillet, N. & Cardin, P. 2003 Quasigeostrophic models of convection in rotating spherical shells. Geochem. Geophys. Geosyst. 4 (7), 1052.Google Scholar
Aurnou, J. M., Calkins, M. A., Cheng, J. S., Julien, K., King, E. M., Nieves, D., Soderlund, K. M. & Stellmach, S. 2015 Rotating convective turbulence in Earth and planetary cores. Phys. Earth Planet. Inter. 246, 52–71.Google Scholar
Bajaj, K. M. S., Ahlers, G. & Pesch, W. 2002 Rayleigh–Bénard convection with rotation at small Prandtl numbers. Phys. Rev. E 65 (5), 056309.Google Scholar
Brummell, N. H. & Hart, J. E. 1993 High Rayleigh number-convection. Geophys. Astrophys. Fluid Dyn. 68, 85–114.Google Scholar
Busse, F. H. 1970 Thermal instabilities in rapidly rotating systems. J. Fluid Mech. 44, 441–460.Google Scholar
Busse, F. H. & Hood, L. L. 1982 Differential rotation driven by convection in a rapidly rotating annulus. Geophys. Astrophys. Fluid Dyn. 21 (1–2), 59–74.CrossRefGoogle Scholar
Busse, F. H. & Or, A. C. 1986 Convection in a rotating cylindrical annulus – thermal Rossby waves. J. Fluid Mech. 166, 173–187.CrossRefGoogle Scholar
Busse, F. H. & Simitev, R. 2004 Inertial convection in rotating fluid spheres. J. Fluid Mech. 498, 23–30.CrossRefGoogle Scholar
Calkins, M. A., Aurnou, J. M., Eldredge, J. D. & Julien, K. 2012 The influence of fluid properties on the morphology of core turbulence and the geomagnetic field. Earth Planet. Sci. Lett. 359, 55–60.Google Scholar
Cardin, P. & Olson, P. 1994 Chaotic thermal convection in a rapidly rotating spherical shell: consequences for flow in the outer core. Phys. Earth Planet. Inter. 82, 235–259.Google Scholar
Carrigan, C. R. & Busse, F. H. 1983 An experimental and theoretical investigation of the onset of convection in rotating spherical shells. J. Fluid Mech. 126, 287–305.CrossRefGoogle Scholar
Chandrasekhar, S. 1961 Hydrodynamic and Hydromagnetic Stability. Clarendon.Google Scholar
Christensen, U. R. 2002 Zonal flow driven by strongly supercritical convection in rotating spherical shells. J. Fluid Mech. 470, 115–133.Google Scholar
Clever, R. M. & Busse, F. H. 2000 Convection in a low Prandtl number fluid layer rotating about a vertical axis. Eur. J. Mech. (B/Fluids) 19 (2), 213–227.Google Scholar
Cordero, S. & Busse, F. H. 1992 Experiments on convection in rotating hemispherical shells: Transition to a quasi-periodic state. Geophys. Res. Lett. 19 (8), 733–736.Google Scholar
Costa, L. D., Knobloch, E. & Weiss, N. O. 1981 Oscillations in double-diffusive convection. J. Fluid Mech. 109 (257), 25–43.Google Scholar
Dormy, E. 2016 Strong-field spherical dynamos. J. Fluid Mech. 789, 500–513.Google Scholar
Dormy, E., Soward, A. M., Jones, C. A., Jault, D. & Cardin, P. 2004 The onset of thermal convection in rotating spherical shells. J. Fluid Mech. 501, 43–70.CrossRefGoogle Scholar
Eltayeb, I. A. 1972 Hydromagnetic convection in a rapidly rotating fluid layer. Proc. R. Soc. Lond. A 326 (1565), 229–254.Google Scholar
Fauve, S. 1998 Pattern forming instabilities. In Hydrodynamics and Non Linear Instabilities (ed. Godrèche, C. & Manneville, P.), pp. 387–489. Cambridge University Press.Google Scholar
Fearn, D. R. 1979 Thermal and magnetic instabilities in a rapidly rotating fluid sphere. Geophys. Astrophys. Fluid Dyn. 14 (1), 103–126.Google Scholar
Gillet, N., Brito, D., Jault, D. & Nataf, H.-C. 2007 Experimental and numerical study of convection in a rapidly rotating spherical shell. J. Fluid Mech. 580, 83–121.Google Scholar
Gillet, N. & Jones, C. A. 2006 The quasi-geostrophic model for rapidly rotating spherical convection outside the tangent cylinder. J. Fluid Mech. 554, 343–369.Google Scholar
Goluskin, D. 2016 Internally Heated Convection and Rayleigh–Bénard Convection. Springer.CrossRefGoogle Scholar
Greenspan, H. P. 1968 The Theory of Rotating Fluids. University Press.Google Scholar
Grote, E. & Busse, F. H. 2001 Dynamics of convection and dynamos in rotating spherical fluid shells. Fluid Dyn. Res. 28, 349–368.Google Scholar
Guervilly, C., Cardin, P. & Schaeffer, N. 2012 A dynamo driven by zonal jets at the upper surface: Applications to giant planets. Icarus 218, 100–114.CrossRefGoogle Scholar
Hori, K. & Wicht, J. 2013 Subcritical dynamos in the early Mars’ core: implications for cessation of the past Martian dynamo. Phys. Earth Planet. Inter. 219, 21–33.Google Scholar
Ingersoll, A. P. & Pollard, D. 1982 Motion in the interiors and atmospheres of Jupiter and Saturn – scale analysis, anelastic equations, barotropic stability criterion. Icarus 52, 62–80.Google Scholar
Jones, C. A. 2007 Thermal and compositional convection in the outer core. In Treatise on Geophysics (ed. Schubert, G.), pp. 131–185. Elsevier.Google Scholar
Jones, C. A., Rotvig, J. & Abdulrahman, A. 2003 Multiple jets and zonal flow on Jupiter. Geophys. Res. Lett. 30 (14), 140000.CrossRefGoogle Scholar
Jones, C. A., Soward, A. M. & Mussa, A. I. 2000 The onset of thermal convection in a rapidly rotating sphere. J. Fluid Mech. 405, 157–179.CrossRefGoogle Scholar
King, E. M. & Aurnou, J. M. 2013 Turbulent convection in liquid metal with and without rotation. Proc. Natl Acad. Sci. 110 (17), 6688–6693.CrossRefGoogle ScholarPubMed
Kuang, W., Jiang, W. & Wang, T. 2008 Sudden termination of Martian dynamo? Implications from subcritical dynamo simulations. Geophys. Res. Lett. 35, L14204.Google Scholar
Kuo, H.-L. 1949 Dynamic instability of two-dimensional nondivergent flow in a barotropic atmosphere. J. Atmos. Sci. 6, 105–122.Google Scholar
Labbé, F., Jault, D. & Gillet, N. 2015 On magnetostrophic inertia-less waves in quasi-geostrophic models of planetary cores. Geophys. Astrophys. Fluid Dyn. 109 (6), 587–610.Google Scholar
Manneville, P. 2004 Instabilities, Chaos and Turbulence. Imperial College Press.Google Scholar
Matsui, H., Heien, E., Aubert, J., Aurnou, J. M., Avery, M., Brown, B., Buffett, B. A., Busse, F., Christensen, U. R., Davies, C. J. et al. 2016 Performance benchmarks for a next generation numerical dynamo model. Geochem. Geophys. Geosyst. 17 (5), 1586–1607.Google Scholar
Miyagoshi, T., Kageyama, A. & Sato, T. 2010 Zonal flow formation in the Earth’s core. Nature 463, 793–796.CrossRefGoogle ScholarPubMed
Morin, V. & Dormy, E. 2004 Time dependent beta-convection in rapidly rotating spherical shells. Phys. Fluids 16 (5), 1603–1609.Google Scholar
Morin, V. & Dormy, E. 2009 The dynamo bifurcation in rotating spherical shells. Intl J. Mod. Phys. B 23, 5467–5482.Google Scholar
Nataf, H.-C. & Schaeffer, N. 2015 Turbulence in the core. In Treatise on Geophysics, 2nd edn. (ed. Schubert, G.), pp. 161–181. Elsevier.Google Scholar
Olson, P. L. 2013 The new core paradox. Science 342 (6157), 431–432.CrossRefGoogle ScholarPubMed
Or, A. C. & Busse, F. H. 1987 Convection in a rotating cylindrical annulus. II. Transitions to asymmetric and vacillating flow. J. Fluid Mech. 174, 313–326.CrossRefGoogle Scholar
Plaut, E. & Busse, F. H. 2002 Low-Prandtl-number convection in a rotating cylindrical annulus. J. Fluid Mech. 464, 345–363.Google Scholar
Plaut, E. & Busse, F. H. 2005 Multicellular convection in rotating annuli. J. Fluid Mech. 528, 119–133.CrossRefGoogle Scholar
Plaut, E., Lebranchu, Y., Simitev, R. & Busse, F. H. 2008 On the Reynolds stresses and mean fields generated by pure waves – applications to shear flows and convection in a rotating shell. J. Fluid Mech. 602, 303–326.Google Scholar
Pozzo, M., Davies, C., Gubbins, D. & Alfe, D. 2012 Thermal and electrical conductivity of iron at Earth/’s core conditions. Nature 485 (7398), 355–358.Google Scholar
Proctor, M. R. E. 1994 Convection and magnetoconvection in a rapidly rotating sphere. In Lectures on Solar and Planetary Dynamos, p. 97.CrossRefGoogle Scholar
Roberts, P. H. 1968 On the thermal instability of a rotating-fluid sphere containing heat sources. Phil. Trans. R. Soc. Lond. A 263, 93–117.Google Scholar
Roberts, P. H. 1988 Future of geodynamo theory. Geophys. Astrophys. Fluid Dyn. 44 (1–4), 3–31.CrossRefGoogle Scholar
Sánchez, J., Garcia, F. & Net, M. 2016 Critical torsional modes of convection in rotating fluid spheres at high Taylor numbers. J. Fluid Mech. 791, R1.Google Scholar
Scanlon, J. W. & Segel, L. A. 1967 Finite amplitude cellular convection induced by surface tension. J. Fluid Mech. 30 (01), 149–162.Google Scholar
Schaeffer, N. & Cardin, P. 2005 Quasigeostrophic model of the instabilities of the Stewartson layer in flat and depth-varying containers. Phys. Fluids 17 (10), 104111.CrossRefGoogle Scholar
Schaeffer, N. & Cardin, P. 2006 Quasi-geostrophic kinematic dynamos at low magnetic Prandtl number. Earth Planet. Sci. Lett. 245, 595–604.Google Scholar
Schnaubelt, M. & Busse, F. H. 1992 Convection in a rotating cylindrical annulus part 3. Vacillating and spatially modulated flows. J. Fluid Mech. 245, 155–173.Google Scholar
Simitev, R. & Busse, F. H. 2003 Patterns of convection in rotating spherical shells. New J. Phys. 5 (1), 97.Google Scholar
Soward, A. M. 1977 On the finite amplitude thermal instability of a rapidly rotating fluid sphere. Geophys. Astrophys. Fluid Dyn. 9 (1), 19–74.Google Scholar
Sreenivasan, B. & Jones, C. A. 2011 Helicity generation and subcritical behaviour in rapidly rotating dynamos. J. Fluid Mech. 688, 5.Google Scholar
Stellmach, S. & Hansen, U. 2004 Cartesian convection driven dynamos at low Ekman number. Phys. Rev. E 70, 056312.Google Scholar
Sumita, I. & Olson, P. 2000 Laboratory experiments on high Rayleigh number thermal convection in a rapidly rotating hemispherical shell. Phys. Earth Planet. Inter. 117, 153–170.Google Scholar
Sun, Z.-P., Schubert, G. & Glatzmaier, G. A. 1993 Transitions to chaotic thermal convection in a rapidly rotating spherical fluid shell. Geophys. Astrophys. Fluid Dyn. 69, 95–131.Google Scholar
Teed, R. J., Jones, C. A. & Hollerbach, R. 2012 On the necessary conditions for bursts of convection within the rapidly rotating cylindrical annulus. Phys. Fluids 24 (6), 066604.Google Scholar
Tilgner, A. & Busse, F. H. 1997 Finite-amplitude convection in rotating spherical fluid shells. J. Fluid Mech. 332 (1), 359–376.Google Scholar
Veronis, G. 1966 Motions at subcritical values of the Rayleigh number in a rotating fluid. J. Fluid Mech. 24 (03), 545–554.Google Scholar
Yadav, R. K., Gastine, T., Christensen, U. R., Duarte, L. D. V. & Reiners, A. 2016 Effect of shear and magnetic field on the heat-transfer efficiency of convection in rotating spherical shells. Geophys. J. Int. 204 (2), 1120–1133.Google Scholar
Yano, J.-I. 1992 Asymptotic theory of thermal convection in rapidly rotating systems. J. Fluid Mech. 243, 103–131.CrossRefGoogle Scholar
Zhang, K. 1992a Convection in a rapidly rotating spherical shell at infinite prandtl number: transition to vacillating flows. Phys. Earth Planet. Inter. 72 (3–4), 236–248.Google Scholar
Zhang, K. 1992a Spiralling columnar convection in rapidly rotating spherical fluid shells. J. Fluid Mech. 236, 535–556.Google Scholar
Zhang, K.-K. & Busse, F. H. 1987 On the onset of convection in rotating spherical shells. Geophys. Astrophys. Fluid Dyn. 39, 119–147.CrossRefGoogle Scholar
Zhang, K. & Jones, C. A. 1993 The influence of Ekman boundary layers on rotating convection. Geophys. Astrophys. Fluid Dyn. 71 (1–4), 145–162.Google Scholar