Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Thomas, Jim
and
Yamada, Ray
2019.
Geophysical turbulence dominated by inertia–gravity waves.
Journal of Fluid Mechanics,
Vol. 875,
Issue. ,
p.
71.
Shakespeare, Callum J.
2019.
Spontaneous generation of internal waves.
Physics Today,
Vol. 72,
Issue. 6,
p.
34.
Thomas, Leif N.
2019.
Enhanced Radiation of Near-Inertial Energy by Frontal Vertical Circulations.
Journal of Physical Oceanography,
Vol. 49,
Issue. 9,
p.
2407.
Torres, H. S.
Klein, P.
Siegelman, L.
Qiu, B.
Chen, S.
Ubelmann, C.
Wang, J.
Menemenlis, D.
and
Fu, L.‐L.
2019.
Diagnosing Ocean‐Wave‐Turbulence Interactions From Space.
Geophysical Research Letters,
Vol. 46,
Issue. 15,
p.
8933.
Asselin, Olivier
and
Young, William R.
2019.
An improved model of near-inertial wave dynamics.
Journal of Fluid Mechanics,
Vol. 876,
Issue. ,
p.
428.
Klein, Patrice
Lapeyre, Guillaume
Siegelman, Lia
Qiu, Bo
Fu, Lee‐Lueng
Torres, Hector
Su, Zhan
Menemenlis, Dimitris
and
Le Gentil, Sylvie
2019.
Ocean‐Scale Interactions From Space.
Earth and Space Science,
Vol. 6,
Issue. 5,
p.
795.
Su, Zhan
Torres, Hector
Klein, Patrice
Thompson, Andrew F.
Siegelman, Lia
Wang, Jinbo
Menemenlis, Dimitris
and
Hill, Christopher
2020.
High‐Frequency Submesoscale Motions Enhance the Upward Vertical Heat Transport in the Global Ocean.
Journal of Geophysical Research: Oceans,
Vol. 125,
Issue. 9,
Callies, Jörn
Barkan, Roy
and
Garabato, Alberto Naveira
2020.
Time Scales of Submesoscale Flow Inferred from a Mooring Array.
Journal of Physical Oceanography,
Vol. 50,
Issue. 4,
p.
1065.
Xie, Jin-Han
2020.
Downscale transfer of quasigeostrophic energy catalyzed by near-inertial waves.
Journal of Fluid Mechanics,
Vol. 904,
Issue. ,
Kung, Hiu Suet
and
Gan, Jianping
2020.
Response of near-inertial energy to a supercritical tropical cyclone and jet in the South China Sea: modelling study.
Ocean Science,
Vol. 16,
Issue. 5,
p.
1095.
Asselin, Olivier
and
Young, William R.
2020.
Penetration of Wind-Generated Near-Inertial Waves into a Turbulent Ocean.
Journal of Physical Oceanography,
Vol. 50,
Issue. 6,
p.
1699.
Erickson, Zachary K.
Thompson, Andrew F.
Callies, Jörn
Yu, Xiaolong
Garabato, Alberto Naveira
and
Klein, Patrice
2020.
The Vertical Structure of Open-Ocean Submesoscale Variability during a Full Seasonal Cycle.
Journal of Physical Oceanography,
Vol. 50,
Issue. 1,
p.
145.
Thomas, Jim
and
Daniel, Don
2020.
Turbulent exchanges between near-inertial waves and balanced flows.
Journal of Fluid Mechanics,
Vol. 902,
Issue. ,
Taylor, Stephanne
and
Straub, David
2020.
Effects of Adding Forced Near-Inertial Motion to a Wind-Driven Channel Flow.
Journal of Physical Oceanography,
Vol. 50,
Issue. 10,
p.
2983.
Thomas, Leif N.
Rainville, Luc
Asselin, Olivier
Young, William R.
Girton, James
Whalen, Caitlin B.
Centurioni, Luca
and
Hormann, Verena
2020.
Direct Observations of Near‐Inertial Wave ζ‐Refraction in a Dipole Vortex.
Geophysical Research Letters,
Vol. 47,
Issue. 21,
Asselin, Olivier
Thomas, Leif N.
Young, William R.
and
Rainville, Luc
2020.
Refraction and Straining of Near-Inertial Waves by Barotropic Eddies.
Journal of Physical Oceanography,
Vol. 50,
Issue. 12,
p.
3439.
Thomas, Jim
and
Arun, S.
2020.
Near-inertial waves and geostrophic turbulence.
Physical Review Fluids,
Vol. 5,
Issue. 1,
Seshasayanan, Kannabiran
and
Gallet, Basile
2020.
Onset of three-dimensionality in rapidly rotating turbulent flows.
Journal of Fluid Mechanics,
Vol. 901,
Issue. ,
Cusack, Jesse M.
Brearley, J. Alexander
Naveira Garabato, Alberto C.
Smeed, David A.
Polzin, Kurt L.
Velzeboer, Nick
and
Shakespeare, Callum J.
2020.
Observed Eddy–Internal Wave Interactions in the Southern Ocean.
Journal of Physical Oceanography,
Vol. 50,
Issue. 10,
p.
3043.
Thomas, Jim
and
Daniel, Don
2021.
Forward flux and enhanced dissipation of geostrophic balanced energy.
Journal of Fluid Mechanics,
Vol. 911,
Issue. ,
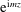 $\text{e}^{\text{i}mz}$ vertical structure, where
$\text{e}^{\text{i}mz}$ vertical structure, where 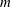 $m$ is the vertical wavenumber and
$m$ is the vertical wavenumber and  $z$ is the vertical coordinate. A detailed description of the conservation laws of this vertical-plane-wave model illuminates the mechanism of stimulated generation associated with vertical vorticity and lateral strain. There are two sources of wave potential energy, and corresponding sinks of balanced kinetic energy: the refractive convergence of wave action density into anti-cyclones (and divergence from cyclones); and the enhancement of wave-field gradients by geostrophic straining. We quantify these energy transfers and describe the phenomenology of stimulated generation using numerical solutions of an initially uniform inertial oscillation interacting with mature freely evolving two-dimensional turbulence. In all solutions, stimulated generation co-exists with a transfer of balanced kinetic energy to large scales via vortex merging. Also, geostrophic straining accounts for most of the generation of wave potential energy, representing a sink of 10 %–20 % of the initial balanced kinetic energy. However, refraction is fundamental because it creates the initial eddy-scale lateral gradients in the near-inertial field that are then enhanced by advection. In these quasi-inviscid solutions, wave dispersion is the only mechanism that upsets stimulated generation: with a barotropic balanced flow, lateral straining enhances the wave group velocity, so that waves accelerate and rapidly escape from straining regions. This wave escape prevents wave energy from cascading to dissipative scales.
$z$ is the vertical coordinate. A detailed description of the conservation laws of this vertical-plane-wave model illuminates the mechanism of stimulated generation associated with vertical vorticity and lateral strain. There are two sources of wave potential energy, and corresponding sinks of balanced kinetic energy: the refractive convergence of wave action density into anti-cyclones (and divergence from cyclones); and the enhancement of wave-field gradients by geostrophic straining. We quantify these energy transfers and describe the phenomenology of stimulated generation using numerical solutions of an initially uniform inertial oscillation interacting with mature freely evolving two-dimensional turbulence. In all solutions, stimulated generation co-exists with a transfer of balanced kinetic energy to large scales via vortex merging. Also, geostrophic straining accounts for most of the generation of wave potential energy, representing a sink of 10 %–20 % of the initial balanced kinetic energy. However, refraction is fundamental because it creates the initial eddy-scale lateral gradients in the near-inertial field that are then enhanced by advection. In these quasi-inviscid solutions, wave dispersion is the only mechanism that upsets stimulated generation: with a barotropic balanced flow, lateral straining enhances the wave group velocity, so that waves accelerate and rapidly escape from straining regions. This wave escape prevents wave energy from cascading to dissipative scales.