Hostname: page-component-586b7cd67f-2brh9 Total loading time: 0 Render date: 2024-12-02T18:17:14.012Z Has data issue: false hasContentIssue false
  • English
  • Français

A stable tripole vortex model in two-dimensional Euler flows

Published online by Cambridge University Press:  17 September 2019

A. Viúdez Open the ORCID record for A. Viúdez [Opens in a new window]
Get access Rights & Permissions [Opens in a new window]

Abstract

An exact solution of a stable vortex tripole in two-dimensional (2-D) Euler flows is provided. The stable tripole is composed of an inner elliptical vortex and two small-amplitude lateral vortices. The non-vanishing vorticity field of this tripole, referred to as here as an embedded tripole because of the closeness of its vortices, is given in elliptical coordinates 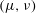$(\unicode[STIX]{x1D707},\unicode[STIX]{x1D708})$ by the even radial and angular order-0 Mathieu functions $\text{Je}_{0}(\unicode[STIX]{x1D707})\text{ce}_{0}(\unicode[STIX]{x1D708})$ truncated at the external branch of the vorticity isoline passing through the two critical points closest to the vortex centre. This tripole mode has a rigid vorticity field which rotates with constant angular velocity equal to $\unicode[STIX]{x1D701}_{0}\text{Je}_{0}(\unicode[STIX]{x1D707}_{1})\text{ce}_{0}(0)/2$, where 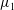$\unicode[STIX]{x1D707}_{1}$ is the first zero of 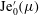$\text{Je}_{0}^{\prime }(\unicode[STIX]{x1D707})$ and 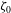$\unicode[STIX]{x1D701}_{0}$ is a constant modal amplitude. It is argued that embedded 2-D tripoles may be conceptually regarded as the superposition of two asymmetric Chaplygin–Lamb dipoles, separated a distance equal to 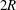$2R$, as long as their individual trajectory curvature radius 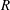$R$ is much shorter than their dipole extent radius.

JFM classification

Vortex Flows: Vortex dynamics Vortex Flows: Vortex instability Vortex Flows: Vortex interactions
Type
JFM Rapids
Information
Journal of Fluid Mechanics , Volume 878 , 10 November 2019 , R5
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abramowitz, M. & Stegun, I. 1964 Handbook of Mathematical Functions. Dover.Google Scholar
Carton, X. J., Flierl, G. R. & Polvani, L. M. 1989 The generation of tripoles from unstable axisymmetric isolated vortex structures. Europ. Phys. Lett. 9, 339–344.Google Scholar
Chaplygin, S. A. 1903 One case of vortex motion in fluid. Trans. Phys. Sect. Imperial Moscow Soc. Friends of Natural Sciences 11 (N2), 11–14.Google Scholar
Dritschel, D. G. & Viúdez, A. 2003 A balanced approach to modelling rotating stably-stratified geophysical flows. J. Fluid Mech. 488, 123–150.Google Scholar
Flierl, G. R., Stern, M. E. & Whitehead, J. A. 1983 The physical significance of modons: laboratory experiments and general integral constraints. Dyn. Atmos. Oceans 7, 233–263.Google Scholar
Gutiérrez-Vega, J. C., Rodríguez-Dagnino, R. M., Meneses-Nava, M. A. & Chávez-Cerda, S. 2003 Mathieu functions, a visual approach. Am. J. Phys. 71, 233–242.Google Scholar
van Heijst, G. J. F. & Kloosterziel, R. C. 1989 Tripolar vortices in a rotating fluid. Nature 338, 569–571.Google Scholar
van Heijst, G. J. F., Kloosterziel, R. C. & Williams, C. W. M. 1991 Laboratory experiments on the tripolar vortex in a rotating fluid. J. Fluid Mech. 225, 301–331.Google Scholar
Kizner, Z. & Khvoles, R. 2004 The tripole vortex: experimental evidence and explicit solutions. Phys. Rev. E 70, 016307.Google Scholar
Lamb, H. 1895 Hydrodynamics, 2nd edn. Cambridge University Press.Google Scholar
McLachlan, N. W. 1951 Theory and Application of Mathieu Functions. Oxford Press.Google Scholar
Meleshko, V. V. & van Heijst, G. J. F. 1994 On Chaplygin’s investigations of two-dimensional vortex structures in an inviscid fluid. J. Fluid Mech. 272, 157–182.Google Scholar
Okamoto, A., Hara, K., Nagaoka, K., Yoshimura, S., Vranješ, J., Kono, M. & Tanaka, M. Y. 2003 Experimental observation of a tripolar vortex in a plasma. Phys. Plasmas 10 (6), 2211–2216.Google Scholar
Orlandi, P. & van Heijst, G. J. F. 1992 Numerical simulation of tripolar vortices in 2D flow. Fluid Dyn. Res. 9, 179–206.Google Scholar
Pingree, R. D. & Le Cann, B. 1992 Three anticyclonic slope water oceanic eDDIES (SWODDIES) in the Southern Bay of Biscay in 1990. Deep Sea Res. 39, 1147–1175.Google Scholar
Polvani, M. L. & Carton, X. J. 1990 The tripole: a new coherent vortex structure of incompressible two-dimensional flows. Geophys. Astrophys. Fluid Dyn. 51 (1–4), 87–102.Google Scholar
Viúdez, A. 2019 Azimuthal-mode solutions of two-dimensional Euler flows and the Chaplygin–Lamb dipole. J. Fluid Mech. 859, R1.Google Scholar

Viúdez supplementary movie 1

See separate caption file

Download Viúdez supplementary movie 1(Video)
Video 9.7 MB
Supplementary material: PDF

Viúdez supplementary material

Movie 1 caption

Download Viúdez supplementary material(PDF)
PDF 20.7 KB

Viúdez supplementary movie 2

See separate caption file

Download Viúdez supplementary movie 2(Video)
Video 8.4 MB
Supplementary material: PDF

Viúdez supplementary material

Movie 2 caption

Download Viúdez supplementary material(PDF)
PDF 20.8 KB
Supplementary material: File

Viúdez supplementary material

Supplementary data

Download Viúdez supplementary material(File)
File 4.8 MB