Hostname: page-component-586b7cd67f-2brh9 Total loading time: 0 Render date: 2024-12-01T02:48:36.878Z Has data issue: false hasContentIssue false
  • English
  • Français

Stabilization of fluidized beds of particles magnetized by an external field: effects of particle size and field orientation

Published online by Cambridge University Press:  02 September 2013

M. J. Espin ,
J. M. Valverde  and
M. A. S. Quintanilla
M. J. Espin
Affiliation:
Department of Applied Physics II, University of Seville, Avenida Reina Mercedes s/n, 41012 Sevilla, Spain
J. M. Valverde*
Affiliation:
Department of Applied Physics II, University of Seville, Avenida Reina Mercedes s/n, 41012 Sevilla, Spain
M. A. S. Quintanilla
Affiliation:
Department of Electronics and Electromagnetism, University of Seville, Avenida Reina Mercedes s/n, 41012 Sevilla, Spain
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

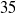This paper reports experimental measurements on the yield stress, the permeability to gas flow and the gas velocity at the jamming transition of gas-fluidized beds of magnetizable particles as affected by particle size and orientation and strength of an externally imposed magnetic field. Tested samples consisted of relatively monodisperse magnetite powders of $35$, 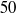$50$ and 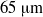$65~\unicode[.5,0][STIXGeneral,Times]{x03BC} \mathrm{m} $ particle size. The permeability to gas flow and jamming transition velocity increase with particle size and in a specially marked way when the magnetic field is applied along the gas flow direction. The magnetic contribution to the yield stress is also particularly enhanced for co-flow magnetic fields. However, the effect of particle size on the yield stress shows a dependence on the microstructure packing as affected by particle size and orientation of the field. The magnetic yield stress increases with particle size for magnetic fields applied in the cross-flow configuration while the opposite trend is observed when the direction of the magnetic field is parallel to the gas flow. The observations reported in this paper are generally explained by the formation of chains of particles due to attractive magnetic forces between the magnetized particles and the orientation of these chains with respect to the magnetic field.

JFM classification

Multiphase and Particle-laden Flows: Fluidized beds Multiphase and Particle-laden Flows: Multiphase and Particle-laden Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 732 , 10 October 2013 , pp. 282 - 303
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Albert, R. V. & Tien, C. 1985 Particle collection in magnetically stabilized fluidized filters. AIChE J. 31 (2), 288–295.CrossRefGoogle Scholar
Batchelor, G. K. 1988 A new theory of the instability of a uniform fluidized bed. J. Fluid Mech. 193, 75–110.CrossRefGoogle Scholar
Carman, P. C. 1937 Fluid flow through granular beds. Trans. Inst. Chem. Engrs 15, 150–167.Google Scholar
Castellanos, A., Valverde, J. M. & Quintanilla, M. A. S. 2004 The sevilla powder tester: a tool for characterizing and investigating the physical properties of fine cohesive powders. KONA Powder Part. 22, 66–81.CrossRefGoogle Scholar
Castellanos, A., Valverde, J. M. & Quintanilla, M. A. S. 2005 Physics of compaction of fine cohesive particles. Phys. Rev. Lett. 94, 075501.CrossRefGoogle ScholarPubMed
Clercx, H. J. H. & Bossis, G. 1993 Many-body electrostatic interactions in electrorheological fluids. Phys. Rev. E 48, 2721–2738.CrossRefGoogle ScholarPubMed
Cohen, A. H. & Tien, C. 1991 Aerosol filtration in a magnetically stabilized fluidized bed. Powder Technol. 64 (1–2), 147–158.CrossRefGoogle Scholar
Colver, G. M. 1979 The influence of electric and magnetic fields on air-fluidized beds. In Proc. NSF Workshop on Fluidization and Fluid-Particle Systems: Research Needs and Priorities (ed. Littman, H.), p. 57. National Science Foundation.Google Scholar
Constantineau, J. P., Grace, J. R., Lim, C. J. & Richards, G. G. 2007 Generalized bubbling-slugging fluidized bed reactor model. Chem. Engng Sci. 62 (1–2), 70–81.CrossRefGoogle Scholar
De Gans, B. J., Duin, N. J., van den Ende, D. & Mellema, J. 2000 The influence of particle size on the magnetorheological properties of an inverse ferrofluid. J. Chem. Phys. 113, 2032.CrossRefGoogle Scholar
Elliott, R. J., Krumhansl, J. A. & Leath, P. L. 1974 The theory and properties of randomly disordered crystals and related physical systems. Rev. Mod. Phys. 46, 465–543.CrossRefGoogle Scholar
Espin, M. J., Quintanilla, M. A. S., Valverde, J. M. & Castellanos, A. 2010a Rheology of magnetofluidized fine powders: the role of interparticle contact forces. J. Rheol. 54, 719–734.CrossRefGoogle Scholar
Espin, M. J., Valverde, J. M., Quintanilla, M. A. S. & Castellanos, A. 2010b Magnetic field induced inversion in the effect of particle size on powder cohesiveness. J. Chem. Phys. 133 (2), 024706.CrossRefGoogle ScholarPubMed
Espin, M. J., Valverde, J. M., Quintanilla, M. A. S. & Castellanos, A. 2011 Stabilization of gas-fluidized beds of magnetic powders by a cross-flow magnetic field. J. Fluid Mech. 680, 80–113.CrossRefGoogle Scholar
Filippov, M. V. 1960 The effect of a magnetic field on a ferromagnetic particle suspension bed. Prikl. Magnitogidrodin. Tr. Inst. Fiz. Akad. Nauk. Latvia SSR 12, 215–236.Google Scholar
Filippov, M. V. 1961 Resistance and expansion of a fluidized bed of magnetite in a magnetic field. Izv. Akad. Nauk. Latv. SSR 12 (173), 47–51.Google Scholar
Filippov, M. V. 1962a Fluidization of a suspended layer of magnetite in a magnetic field. Latv. PSR Zinat. Akad. Vestis 1 (174), 69–73.Google Scholar
Filippov, M. V. 1962b Some properties of a suspended bed of ferromagnetic particles in a magnetic field. Voprosy Magnitnoi. Gidrodinamiki I Dinamiki Plazmy 635–642.Google Scholar
Geldart, D. 1973 Types of gas fluidization. Powder Technol. 7 (5), 285–292.CrossRefGoogle Scholar
Hamby, R. K. & Liu, Y. A. 1991 Studies in magnetochemical engineering: Part 4. An experimental study of screen-packed and conventional fluidized beds in axial and transverse magnetic fields. Powder Technol. 64 (1–2), 103–113.CrossRefGoogle Scholar
Herschler, A. 1965 Fluid treating method and apparatus. Tech. Rep. 3219318. U.S. Pat.Google Scholar
Herschler, A. 1969 Method for the production and control of fluidized beds. Tech. Rep. 3439899. U.S. Pat.Google Scholar
Hristov, J. 2002 Magnetic field assisted fluidization. A unified approach. Part 1. Fundamentals and relevant hydrodynamics. Rev. Chem. Engng 18 (4–5), 295–512.Google Scholar
Hristov, J. 2003a Magnetic field assisted fluidization. A unified approach. Part 2. Solids batch gas-fluidized beds: versions and rheology. Rev. Chem. Engng 19 (1), 1–132.CrossRefGoogle Scholar
Hristov, J. 2003b Magnetic field assisted fluidization. A unified approach. Part 3. Heat transfer in gas–solid fluidized beds – a critical re-evaluation of the results. Rev. Chem. Engng 19 (3), 229–355.Google Scholar
Hristov, J. 2004 Magnetic field assisted fluidization. A unified approach. Part 4. Moving gas-fluidized beds. Rev. Chem. Engng 20 (5–6), 380–550.Google Scholar
Hristov, J. 2006 Magnetic field assisted fluidization. A unified approach. Part 5. A hydrodynamic treatise on liquid–solid fluidized beds. Rev. Chem. Engng 22 (4–5), 195–375.Google Scholar
Hristov, J. 2007 Magnetic field assisted fluidization. A unified approach. Part 6. Topics of gas–liquid–solid fluidized bed hydrodynamics. Rev. Chem. Engng 23 (6), 373–526.Google Scholar
Hristov, J. 2009 Magnetic field assisted fluidization. A unified approach. Part 7. Mass transfer: chemical reactors, basic studies and practical implementations thereof. Rev. Chem. Engng 25 (1–3), 1–254.CrossRefGoogle Scholar
Hristov, J. 2010 Magnetic field assisted fluidization. A unified approach. Part 8. Mass transfer: magnetically assisted bioprocesses. Rev. Chem. Engng 26 (3–4), 55–128.Google Scholar
Hristov, J. 2012 Magnetic field assisted fluidization. A unified approach. Part 9. Mechanical processing with emphasis on separations. Rev. Chem. Engng 28 (4–6), 243–308.Google Scholar
Hristov, J. Y. 1996 Fluidization of ferromagnetic particles in a magnetic field Part 1. The effect of field line orientation on bed stability. Powder Technol. 87 (1), 59–66.CrossRefGoogle Scholar
Hristov, J. Y. 1998 Fluidization of ferromagnetic particles in a magnetic field Part 2. Field effects on preliminarily gas fluidized bed. Powder Technol. 97 (1), 35–44.CrossRefGoogle Scholar
Ivanov, D. G. & Grozev, G. T. 1970a Determination of the critical fluidization velocity of an iron-chromium catalyst bed in a magnetic field. J. Appl. Chem. USSR 43, 2224–2227.Google Scholar
Ivanov, D. G. & Grozev, G. T. 1970b Determining the critical velocity of a fluidized bed of ferrochrome catalyst for conversion of carbon oxide with water vapour in a magnetic field. C. R. Bulg. Acad. Sci. 23 (7), 787–790.Google Scholar
Ivanov, D. G., Zruncher, I. A. & Vodenichavov, P. L. 1969 Improvements in or relating to the manufacture of ammonia. Tech. Rep. 1148513. Brit. Pat.Google Scholar
Johnson, T. W. & Melcher, J. R. 1975 Electromechanics of electrofluidized beds. Ind. Engng Chem. Fundam. 14, 146–153.CrossRefGoogle Scholar
Jun, J.-B., Uhm, S.-Y., Cho, S.-H. & Suh, K.-D. 2004 Bidisperse electrorheological fluids using hydrolyzed styrene-acrylonitrile copolymer particles: synergistic effect of mixed particle size. Langmuir 20 (6), 2429–2434.CrossRefGoogle ScholarPubMed
Karkkainen, K., Sihvola, A. & Nikoskinen, K. 2001 Analysis of a three-dimensional dielectric mixture with finite difference method. IEEE Trans. Geosci. Remote Sens. 39 (5), 1013–1018.CrossRefGoogle Scholar
Katz, H. 1967 Method of stabilizing a fluidized bed using a glow discharge. Tech. Rep. 3304249. U.S. Pat.Google Scholar
Katz, H. & Sears, J. T. 1969 Electric field phenomena in fluidized and fixed beds. Can. J. Chem. Engng 47 (1), 50–53.CrossRefGoogle Scholar
Kirko, I. M. & Filippov, M. V. 1960 Standard correlations for a fluidized bed of ferromagnetic particles in a magnetic field. Zh. Tekh. Fiz. 30 (9), 1081.Google Scholar
Koch, D. L. & Sangani, A. S. 1999 Particle pressure and marginal stability limits for a homogeneous monodisperse gas-fluidized bed: kinetic theory and numerical simulations. J. Fluid Mech. 400, 229–263.CrossRefGoogle Scholar
Kohler, W. E. & Papanicolau, G. C. 1981 Some applications of the coherent potential approximation. In Multiple Scattering and Waves in Random Media (ed. Chow, P. L., Kohler, W. E. & Papanicolau, G. C.), pp. 199–223. North-Holland.Google Scholar
Lee, W. K. 1991 A review of the rheology of magnetically stabilized fluidized beds. Powder Technol. 64 (1–2), 69–80.Google Scholar
Lemaire, E., Meunier, A., Bossis, G., Liu, J., Felt, D., Bashtovoi, P. & Matoussevitch, N. 1995 Influence of the particle size on the rheology of magnetorheological fluids. J. Rheol. 39 (5), 1011–1021.CrossRefGoogle Scholar
Liu, Y. A., Hamby, R. K. & Colberg, R. D. 1991 Fundamental and practical developments of magnetofluidized beds: a review. Powder Technol. 64 (1–2), 3–41.CrossRefGoogle Scholar
Lumay, G. & Vandewalle, N. 2008 Controlled flow of smart powders. Phys. Rev. E 78, 061302.CrossRefGoogle ScholarPubMed
Lumay, G. & Vandewalle, N. 2010 Flow of magnetized grains in a rotating drum. Phys. Rev. E 82, 040301.CrossRefGoogle Scholar
Melcher, J. R., Sachar, K. S. & Warren, E. P. 1977 Overview of electrostatic devices for control of submicrometer particles. Proc. IEEE 65 (12), 1659–1669.CrossRefGoogle Scholar
Quintanilla, M. A. S., Valverde, J. M. & Castellanos, A. 2006 Adhesion force between fine particles with controlled surface properties. AIChE J. 52 (5), 1715–1728.CrossRefGoogle Scholar
Rhodes, M. J., Wang, X. S., Forsyth, A. J., Gan, K. S. & Phadtajaphan, S. 2001 Use of a magnetic fluidized bed in studying Geldart Group B to A transition. Chem. Engng Sci. 56 (18), 5429–5436.CrossRefGoogle Scholar
Rietema, K. 1991 The Dynamics of Fine Powders. Kluwer.CrossRefGoogle Scholar
Rosensweig, R. E. 1979a Fluidization: hydrodynamic stabilization with a magnetic field. Science 204 (4388), 57–60.CrossRefGoogle ScholarPubMed
Rosensweig, R. E. 1979b Magnetic stabilization of the state of uniform fluidization. Ind. Engng Chem. Fundam. 18 (3), 260–269.CrossRefGoogle Scholar
Rosensweig, R. E. 1997 Ferrohydrodynamics. Dover.Google Scholar
Seville, J. P. K. & Clift, R. 1984 The effect of thin liquid layers on fluidisation characteristics. Powder Technol. 37 (1), 117–129.CrossRefGoogle Scholar
Shumkov, S. Kh. & Ivanov, D. G. 1972 Research on the stabilization of electromagnetic field on a fluidized bed with a solid phase of ammonia synthesis catalyst under conditions of high pressure. Annu. Rep. UCTM-Sofia 19 (1), 219–224.Google Scholar
Shumkov, S. Kh. & Ivanov, D. G. 1976 Hydrodynamic characteristics of a fluidized bed in an electromagnetic field. J. Appl. Chem. USSR 49 (1), 2406–2409.Google Scholar
Siegell, J. H. 1987 Liquid-fluidized magnetically stabilized beds. Powder Technol. 52 (2), 139–148.CrossRefGoogle Scholar
Siegell, J. H. 1989 Early studies of magnetized-fluidized beds. Powder Technol. 57 (3), 213–220.CrossRefGoogle Scholar
Sonolikar, R. L. 1989 Magneto-fluidized Beds. In Transport in Fluidized Particle Systems (ed. Doraiswamy, L. K. & Mujumdar, A. S.), pp. 359–423. Elsevier.Google Scholar
Sonolikar, R. L., Ingle, S. G., Giradkar, J. R. & Mene, P. S. 1972 Influence of magnetic field on fluidization of iron particles. Indian J. Tecknol. 10, 377–379.Google Scholar
Sundaresan, S. 2003 Instabilities in fluidized beds. Annu. Rev. Fluid Mech. 35, 63–68.CrossRefGoogle Scholar
Tsinontides, S. C. & Jackson, R. 1993 The mechanics of gas fluidized beds with an interval of stable fluidization. J. Fluid Mech. 255, 237–274.CrossRefGoogle Scholar
Tuthill, E. J. 1969 Magnetically stabilized fluidized bed. Tech. Rep. 3440731. U.S. Pat.Google Scholar
Valverde, J. M., Castellanos, A. & Quintanilla, M. A. S. 2001 Effect of vibration on the stability of a gas-fluidized bed of fine powder. Phys. Rev. E 64, 021302.CrossRefGoogle ScholarPubMed
Valverde, J. M., Castellanos, A. & Quintanilla, M. A. S. 2003 The memory of granular materials. Contemp. Phys. 44 (5), 389–399.Google Scholar
Valverde, J. M., Castellanos, A., Ramos, A., Perez, A. T., Morgan, M. A. & Watson, P. K. 2000 An automated apparatus for measuring the tensile strength and compressibility of fine cohesive powders. Rev. Sci. Instrum. 71 (7), 2791–2795.CrossRefGoogle Scholar
Valverde, J. M., Espin, M. J. & Quintanilla, M. A. S. 2011 Jamming and rheology of fluidized beds of magnetized particles. Appl. Rheol. 21 (3), 1–9.Google Scholar
Valverde, J. M., Espin, M. J., Quintanilla, M. A. S. & Castellanos, A. 2009a Magnetofluidization of fine magnetite powder. Phys. Rev. E 79, 031306.CrossRefGoogle ScholarPubMed
Valverde, J. M., Espin, M. J., Quintanilla, M. A. S. & Castellanos, A. 2009b Mesoscopic structuring and yield stress of magnetofluidized fine particles. Europhys. Lett. 88 (2), 24003.CrossRefGoogle Scholar
Valverde, J. M., Espin, M. J., Quintanilla, M. A. S. & Castellanos, A. 2010 Fluid to solid transition in magnetofluidized beds of fine powders. J. Appl. Phys. 108, 054903.CrossRefGoogle Scholar
Valverde, J. M., Quintanilla, M. A. S. & Castellanos, A. 2004 Jamming threshold of dry fine powders. Phys. Rev. Lett. 92, 258303.CrossRefGoogle ScholarPubMed
Valverde, J. M., Ramos, A., Castellanos, A. & Watson, P. K. 1998 The tensile strength of cohesive powders and its relationship to consolidation, free volume and cohesivity. Powder Technol. 97 (3), 237–245.CrossRefGoogle Scholar
Wu, C. W. & Conrad, H. 1998 Influence of mixed particle size on electrorheological response. J. Appl. Phys. 83, 3880.CrossRefGoogle Scholar
Xie, H.-Y. & Geldart, D. 1995 Fluidization of FCC powders in the bubble-free regime: effect of types of gases and temperature. Powder Technol. 82 (3), 269–277.CrossRefGoogle Scholar
Zahn, M. & Rosensweig, R. E. 1987 Magnetic field gradient effects on magnetic fluid stabilization. J. Magn. Magn. Mater. 65 (2–3), 293–300.CrossRefGoogle Scholar
Zahn, R. E., Rosensweig, M., Lee, W. K. & Hagan, P. S. 1983 Theory and experiments in the mechanics of magnetically stabilized solids. In Theory of Dispersed Multiphase Flow (ed. Meyer, R.), p. 359. Academic.Google Scholar