Published online by Cambridge University Press: 26 April 2023
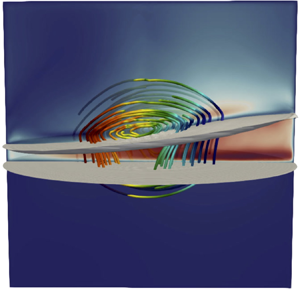
When liquid metal batteries are charged or discharged, strong electrical currents are passing through the three liquid layers that we find in their interior. This may result in the metal pad roll instability that drives gravity waves on the interfaces between the layers. In this paper, we investigate theoretically metal pad roll instability in idealised cylindrical liquid metal batteries that were simulated previously by Weber et al. (Phys. Fluids, vol. 29, no. 5, 2017b, 054101) and Horstmann et al. (J. Fluid Mech., vol. 845, 2018, pp. 1–35). Near the instability threshold, we expect weakly destabilised gravity waves, and in this parameter regime, we can use perturbation methods to find explicit formulas for the growth rate of all possible waves. This perturbative approach also allows us to include dissipative effects, hence we can locate the instability threshold with good precision. We show that our theoretical growth rates are in quantitative agreement with previous and new direct numerical simulations. We explain how our theory can be used to estimate a lower bound on cell size beneath which metal pad roll instability is unlikely.