Hostname: page-component-586b7cd67f-r5fsc Total loading time: 0 Render date: 2024-12-02T22:05:50.411Z Has data issue: false hasContentIssue false
  • English
  • Français

Spilling breakers in shallow water: applications to Favre waves and to the shoaling and breaking of solitary waves

Published online by Cambridge University Press:  02 November 2016

S. L. Gavrilyuk ,
V. Yu. Liapidevskii  and
A. A. Chesnokov
S. L. Gavrilyuk*
Affiliation:
IUSTI, UMR CNRS 7343, Aix-Marseille Université, 5 rue Enrico Fermi, 13453 Marseille CEDEX 13, France Novosibirsk State University, 2 Pirogova Street, 630090 Novosibirsk, Russia
V. Yu. Liapidevskii
Affiliation:
Novosibirsk State University, 2 Pirogova Street, 630090 Novosibirsk, Russia Lavrentyev Institute of Hydrodynamics, 15 Lavrentyev Prospect, 630090 Novosibirsk, Russia
A. A. Chesnokov
Affiliation:
Novosibirsk State University, 2 Pirogova Street, 630090 Novosibirsk, Russia Lavrentyev Institute of Hydrodynamics, 15 Lavrentyev Prospect, 630090 Novosibirsk, Russia
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

A two-layer long-wave approximation of the homogeneous Euler equations for a free-surface flow evolving over mild slopes is derived. The upper layer is turbulent and is described by depth-averaged equations for the layer thickness, average fluid velocity and fluid turbulent energy. The lower layer is almost potential and can be described by Serre–Su–Gardner–Green–Naghdi equations (a second-order shallow water approximation with respect to the parameter 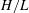$H/L$, where 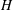$H$ is a characteristic water depth and 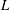$L$ is a characteristic wavelength). A simple model for vertical turbulent mixing is proposed governing the interaction between these layers. Stationary supercritical solutions to this model are first constructed, containing, in particular, a local turbulent subcritical zone at the forward slope of the wave. The non-stationary model was then numerically solved and compared with experimental data for the following two problems. The first one is the study of surface waves resulting from the interaction of a uniform free-surface flow with an immobile wall (the water hammer problem with a free surface). These waves are sometimes called ‘Favre waves’ in homage to Henry Favre and his contribution to the study of this phenomenon. When the Froude number is between 1 and approximately 1.3, an undular bore appears. The characteristics of the leading wave in an undular bore are in good agreement with experimental data by Favre (Ondes de Translation dans les Canaux Découverts, 1935, Dunod) and Treske (J. Hydraul Res., vol. 32 (3), 1994, pp. 355–370). When the Froude number is between 1.3 and 1.4, the transition from an undular bore to a breaking (monotone) bore occurs. The shoaling and breaking of a solitary wave propagating in a long channel (300 m) of mild slope (1/60) was then studied. Good agreement with experimental data by Hsiao et al. (Coast. Engng, vol. 55, 2008, pp. 975–988) for the wave profile evolution was found.

JFM classification

Geophysical and Geological Flows: Shallow water flows Waves/Free-surface Flows: Shear waves Waves/Free-surface Flows: Wave breaking
Type
Papers
Information
Journal of Fluid Mechanics , Volume 808 , 10 December 2016 , pp. 441 - 468
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Antuono, M. & Brocchini, M. 2013 Beyond Boussinesq-type equations: semi-integrated models for coastal dynamics. Phys. Fluids 25, 016603.CrossRefGoogle Scholar
Bardos, C. & Lannes, D. 2012 Mathematics for 2D interfaces. In Singularities in Mechanics: Formation, Propagation and Microscopic Description, Panoramas et Syntheses, vol. 38. Société Mathématique de France.Google Scholar
Barros, R., Gavrilyuk, S. & Teshukov, V. 2007 Dispersive nonlinear waves in two-layer flows with free surface. I. Model derivation and general properties. Stud. Appl. Maths 119, 191–211.Google Scholar
Bonneton, P., Chazel, F., Lannes, D., Marche, F. & Tissier, M. 2011 A splitting approach for the fully nonlinear and weakly dispersive Green–Naghdi model. J. Comput. Phys. 230, 1479–1498.Google Scholar
Bradshaw, P., Ferriss, D. H. & Atwell, N. P. 1967 Calculation of boundary-layer development using the turbulent energy equation. J. Fluid Mech. 28, 593–616.Google Scholar
Brocchini, M. 2002 Free surface boundary conditions at a bubbly/weakly splashing air–water interface. Phys. Fluids 14 (6), 1834–1840.CrossRefGoogle Scholar
Brocchini, M. & Peregrine, D. H. 2001a The dynamics of strong turbulence at free surfaces. Part 1. Description. J. Fluid Mech. 449, 225–254.CrossRefGoogle Scholar
Brocchini, M. & Peregrine, D. H. 2001b The dynamics of strong turbulence at free surfaces. Part 2. Free-surface boundary conditions. J. Fluid Mech. 449, 255–290.Google Scholar
Castro, A. & Lannes, D. 2014 Fully nonlinear long-wave models in the presence of vorticity. J. Fluid Mech. 759, 642–675.CrossRefGoogle Scholar
Chanson, H. 2009 Current knowledge in hydraulic jumps and related phenomena. A survey of experimental results. Eur. J. Mech. (B/Fluids) 28 (2), 191–210.Google Scholar
Chanson, H. & Montes, J. S. 1995 Characteristics of undular hydraulic jumps. Experimental apparatus and flow patterns. J. Hydraul. Engng ASCE 121 (2), 129–144.Google Scholar
Duncan, J. H. 2001 Spilling breakers. Annu. Rev. Fluid Mech. 33, 519–547.Google Scholar
El, G. A., Grimshaw, R. H. J. & Kamchatnov, A. M. 2005 Analytic model for a weakly dissipative shallow water undular bore. Chaos 15, 037102.Google Scholar
El, G. A., Grimshaw, R. H. J. & Smyth, N. F. 2006 Unsteady undular bores in fully non-linear shallow-water theory. Phys. Fluids 18, 027104.Google Scholar
Favre, H. 1935 Ondes de Translation dans les Canaux Découverts. Dunod.Google Scholar
Gavrilyuk, S., Kalisch, H. & Khorsand, Z. 2015 A kinematic conservation law in free surface flow. Nonlinearity 28 (6), 1805–1821.Google Scholar
Gavrilyuk, S. L. & Teshukov, V. M. 2001 Generalized vorticity for bubbly liquid and dispersive shallow water equations. Contin. Mech. Thermodyn. 13, 365–382.Google Scholar
Green, A. E. & Naghdi, P. M. 1976 A derivation of equations for wave propagation in water of variable depth. J. Fluid Mech. 78, 237–246.Google Scholar
Hsiao, S.-C., Hsu, T.-W., Lin, T.-C. & Chang, Y.-H. 2008 On the evolution and run-up of breaking solitary waves on a mild sloping beach. Coast. Engng 55, 975–988.Google Scholar
Le Metayer, O., Gavrilyuk, S. & Hank, S. 2010 A numerical scheme for the Green–Naghdi model. J. Comput. Phys. 229, 2034–2045.CrossRefGoogle Scholar
Lemoine, R. 1948 Sur les ondes positives de translation dans les canaux et sur le ressault ondulé de faible amplitude. La Houille Blanche 1948 (Mars–Avril), 183–185.Google Scholar
Liapidevskii, V. Yu. & Chesnokov, A. A. 2014 Mixing layer under a free surface. J. Appl. Mech. Tech. Phys. 55 (2), 299–310.Google Scholar
Liapidevskii, V. Yu. & Gavrilova, K. N. 2008 Dispersion and blockage effects in the flow over a sill. J. Appl. Mech. Tech. Phys. 49 (1), 34–45.CrossRefGoogle Scholar
Liapidevskii, V. Yu. & Teshukov, V. M. 2000 Mathematical Models of Long Wave Propagation in Non-Homogeneous Fluid. Siberian Division of the Russian Academy of Sciences, Novosibirsk (in Russian).Google Scholar
Liapidevskii, V. Yu. & Xu, Z. 2006 Breaking of waves of limiting amplitude over an obstacle. J. Appl. Mech. Tech. Phys. 47 (3), 307–313.Google Scholar
Lin, J.-C. & Rockwell, D. 1994 Instantaneous structure of a breaking wave. Phys. Fluids 6, 2877–2879.Google Scholar
Longuet-Higgins, M. S. & Turner, J. S. 1974 An ‘entraining plume’ model of a spilling breaker. J. Fluid Mech. 63, 1–20.CrossRefGoogle Scholar
Misra, S. K., Brocchini, M. & Kirby, J. T. 2006 Turbulent interfacial boundary conditions for spilling breakers. In Proc. 30th ICCE, vol. 1, pp. 214–226. World Scientific.Google Scholar
Misra, S. K., Kirby, J. T., Brocchini, M., Veron, F., Thomas, M. & Kambhamettu, C. 2008 The mean and turbulent flow structure of a weak hydraulic jump. Phys. Fluids 20, 035106.Google Scholar
Nadaoka, K., Hino, M. & Koyano, Y. 1989 Structure of the turbulent flow field under breaking waves in the surf zone. J. Fluid Mech. 204, 359–387.Google Scholar
Nessyahu, H. & Tadmor, E. 1990 Non-oscillatory central differencing schemes for hyperbolic conservation laws. J. Comput. Phys. 87, 408–463.Google Scholar
Richard, G. L. & Gavrilyuk, S. L. 2012 A new model of roll waves: comparison with Brock’s experiments. J. Fluid Mech. 698, 374–405.CrossRefGoogle Scholar
Richard, G. L. & Gavrilyuk, S. L. 2013 The classical hydraulic jump in a model of shear shallow water flows. J. Fluid Mech. 725, 492–521.Google Scholar
Richard, G. L. & Gavrilyuk, S. L. 2015 Modelling turbulence generation in solitary waves on shear shallow water flows. J. Fluid Mech. 698, 374–405.Google Scholar
Russo, G. 2005 Central schemes for conservation laws with application to shallow water equations. In Trends and Applications of Mathematics to Mechanics (ed. Rionero, S. & Romano, G.), pp. 225–246. Springer.Google Scholar
Ryabenko, A. A. 1990 Conditions favorable to the existence of an undulating jump. In Hydrotechnical Construction, pp. 29–34; translated from Gidrotechnicheskoe Stroitel’stvo, no. 12.Google Scholar
Serre, F. 1953 Contribution à l’étude des écoulements permanents et variables dans les cannaux. La Houille Blanche 8 (3), 374–388.Google Scholar
Soares-Frazão, S. & Guinot, V. 2008 A second-order semi-implicit hybrid scheme for one-dimensional Boussinesq-type waves in rectangular channels. Intl J. Numer. Meth. Fluids 58 (3), 237–261.Google Scholar
Soares-Frazão, S. & Zech, Y. 2002 Undular bores and secondary waves – experiments and hybrid finite-volume modeling. J. Hydraul Res. 40 (1), 33–43.Google Scholar
Su, C. H. & Gardner, C. S. 1969 Korteweg–de Vries equation and generalisations. III. Derivation of the Korteweg–de Vries equation and Burgers equation. J.  Math. Phys. 10, 536–539.CrossRefGoogle Scholar
Svendsen, I. A. & Madsen, P. A. 1984 A turbulent bore on a beach. J. Fluid Mech. 148, 73–96.CrossRefGoogle Scholar
Teshukov, V. M. 2007 Gas-dynamics analogy for vortex free-boundary flows. J. Appl. Mech. Tech. Phys. 48 (3), 303–309.Google Scholar
Tissier, M., Bonneton, P., Marche, F., Chazel, F. & Lannes, D. 2011 Nearshore dynamics of tsunami-like undular bore using a fully nonlinear Boussinesq model. J. Coast. Res. SI 64, 603–607; (Proceedings of the 11th International Coastal Symposium).Google Scholar
Townsend, A. A. 1956 The Structure of Turbulent Shear Flow. Cambridge University Press.Google Scholar
Treske, A. 1994 Undular bores (Favre-waves) in open channels: experimental studies. J. Hydraul Res. 32 (3), 355–370.Google Scholar