1 Introduction
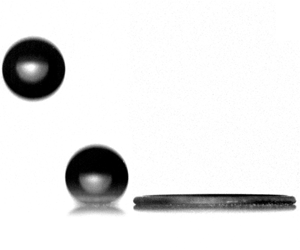
By observing nature on a misty morning, one will notice that a number of plants are naturally engineered to repel water. Tiny droplets appear as shiny marbles which glide at the faintest quivering of the leaves. This remarkable feature is derived from the presence of a micrometre- or nanometre-scaled hydrophobic roughness on the surface (Neinhuis & Barthlott Reference Neinhuis and Barthlott1997). In the presence of water, large pockets of air remain entrapped between the textures: the rough material thus behaves as if it had a hybrid surface made of both solid and gas. Air is thus at the heart of two highly unusual attributes of the so-called superhydrophobic materials: extreme water-repellency and large-scale slipperiness. While water-repellency is captured by the contact angle of droplets, typically higher than  $160^{\circ }$ (figure 1a), the amplitude of the slippage is measured through the slip length
$160^{\circ }$ (figure 1a), the amplitude of the slippage is measured through the slip length  $b$ – the extrapolated distance where the liquid tangential velocity vanishes (figure 1b). This length
$b$ – the extrapolated distance where the liquid tangential velocity vanishes (figure 1b). This length  $b$, which is typically molecular on hydrophilic materials and of the order of 10 nm on hydrophobic surfaces, is multiplied by a factor of 1000 on superhydrophobic substrates. The two distinct properties of these materials have attracted the attention of different communities, drawn either by the unusual dynamics of drops deposited on the surfaces (Blossey Reference Blossey2003) or by the drag-reduction potential of the immersed solids (Rothstein Reference Rothstein2010).
$b$, which is typically molecular on hydrophilic materials and of the order of 10 nm on hydrophobic surfaces, is multiplied by a factor of 1000 on superhydrophobic substrates. The two distinct properties of these materials have attracted the attention of different communities, drawn either by the unusual dynamics of drops deposited on the surfaces (Blossey Reference Blossey2003) or by the drag-reduction potential of the immersed solids (Rothstein Reference Rothstein2010).
Figure 1. Some properties of superhydrophobic surfaces. (a) Non-wetting: droplets deposited on the surface keep the spherical shape they have in air. (b) Large-scale slipperiness: surfaces covered with water are extremely slippery, with a slip length  $b$ of
$b$ of  ${\sim}10~\unicode[STIX]{x03BC}\text{m}$ (defined as the distance below the top of the pillars for which the velocity profile of a flowing liquid vanishes), 1000 times higher than on hydrophobic materials. (c) Drop bouncing on a non-wetting surface.
${\sim}10~\unicode[STIX]{x03BC}\text{m}$ (defined as the distance below the top of the pillars for which the velocity profile of a flowing liquid vanishes), 1000 times higher than on hydrophobic materials. (c) Drop bouncing on a non-wetting surface.
While a nanostructure alone is enough to promote excellent water repellency, most plants and insects possess a hierarchical structure. The celebrated lotus leaf, for example, is covered with  $20~\unicode[STIX]{x03BC}\text{m}$ tall papillae, themselves covered with waxy nano-structures at the scale of 100 nm. On the one hand, the presence of a large texture can be seen as detrimental for the lotus leaf: it makes it sensitive to condensation (Cheng & Rodak Reference Cheng and Rodak2005) and to droplet intrusion at impact (Deng et al. Reference Deng, Varanasi, Hsu, Bhate, Keimel, Stein and Blohm2009). On the other hand, it does make the surface extremely slippery: tall pillars separated by a relatively large distance, generate giant slip lengths of the order of
$20~\unicode[STIX]{x03BC}\text{m}$ tall papillae, themselves covered with waxy nano-structures at the scale of 100 nm. On the one hand, the presence of a large texture can be seen as detrimental for the lotus leaf: it makes it sensitive to condensation (Cheng & Rodak Reference Cheng and Rodak2005) and to droplet intrusion at impact (Deng et al. Reference Deng, Varanasi, Hsu, Bhate, Keimel, Stein and Blohm2009). On the other hand, it does make the surface extremely slippery: tall pillars separated by a relatively large distance, generate giant slip lengths of the order of  $10~\unicode[STIX]{x03BC}\text{m}$ (Ybert et al. Reference Ybert, Barentin, Cottin-Bizonne, Joseph and Bocquet2007). One might then wonder: What role does the slip play when the leaf is lashed by rain? This original question is the starting point of the work of Martouzet et al. (Reference Martouzet, Lee, Pirat, Ybert and Biance2020), whose approach also sheds new light onto a much wider and debated issue on the role of friction on the impact dynamics of droplets on both wetting and non-wetting substrates.
$10~\unicode[STIX]{x03BC}\text{m}$ (Ybert et al. Reference Ybert, Barentin, Cottin-Bizonne, Joseph and Bocquet2007). One might then wonder: What role does the slip play when the leaf is lashed by rain? This original question is the starting point of the work of Martouzet et al. (Reference Martouzet, Lee, Pirat, Ybert and Biance2020), whose approach also sheds new light onto a much wider and debated issue on the role of friction on the impact dynamics of droplets on both wetting and non-wetting substrates.
2 Overview
Martouzet et al. (Reference Martouzet, Lee, Pirat, Ybert and Biance2020) focus on one of the most emblematic properties of non-wetting surfaces: their ability to repel impacting drops (figure 1c). An important parameter here is the time the droplets spend in contact with the substrate, which determines the amount of mechanical and thermal exchange with the solid. In practical problems, such as the freezing of aeroplane wings, it is crucial to make the contact time as short as possible. Recent studies have demonstrated that the addition of well-chosen textures dramatically decrease the recoiling time, leading to a contact time reduction by a factor of two or more (Bird et al. Reference Bird, Dhiman, Kwon and Varanasi2013; Liu et al. Reference Liu, Moevius, Xu, Qian, Yeomans and Wang2014; Gauthier et al. Reference Gauthier, Symon, Clanet and Quéré2015). It is much harder, however, to reduce the duration of the spreading. Numerous experiments and simulations show that the first stage of impact is only marginally influenced by the surface wetting properties, or by the impact velocity of the droplet. Despite numerous studies, the physical parameters that limit the spreading are still being discussed. More importantly, conflicting theoretical models (Clanet et al. Reference Clanet, Béguin, Richard and Quéré2004; Roisman Reference Roisman2009; Eggers et al. Reference Eggers, Fontelos, Josserand and Zaleski2010) show reasonably good agreement with both simulations and experiments (see Josserand & Thoroddsen (Reference Josserand and Thoroddsen2016) for a review). In their recent paper, Martouzet et al. (Reference Martouzet, Lee, Pirat, Ybert and Biance2020) tackle experimentally and theoretically this complex problem. Through a clever choice of surfaces, they clearly evidence the role of a lubricating air layer on the impact. They present their results in a global phase diagram in terms of Reynolds ( $Re$) and Weber (
$Re$) and Weber ( $We$) numbers (which compare inertial forces, respectively, to viscous and surface tension forces) – an original approach which reconciles the theoretical models to the spreading dynamics.
$We$) numbers (which compare inertial forces, respectively, to viscous and surface tension forces) – an original approach which reconciles the theoretical models to the spreading dynamics.
To disentangle the effects of wetting and friction, Martouzet et al. (Reference Martouzet, Lee, Pirat, Ybert and Biance2020) compare drop impact dynamics on two surfaces with identical non-wetting properties but with very different textures. Scanning electron microscope images of the substrates are presented in figure 2: the first surface (figure 2a) has a hierarchical structure, similar to the lotus leaf, made of tall pillars covered with nanometre-sized hairs. The large texture confers to the surface an extreme slipperiness, with a slip length  $b=35~\unicode[STIX]{x03BC}\text{m}$. The second surface (figure 2b) is covered with a unique texture at a smaller scale, associated with a moderate slip (
$b=35~\unicode[STIX]{x03BC}\text{m}$. The second surface (figure 2b) is covered with a unique texture at a smaller scale, associated with a moderate slip ( $b=8~\unicode[STIX]{x03BC}\text{m}$). An originality of the work of Martouzet et al. (Reference Martouzet, Lee, Pirat, Ybert and Biance2020) is to focus on the spreading time
$b=8~\unicode[STIX]{x03BC}\text{m}$). An originality of the work of Martouzet et al. (Reference Martouzet, Lee, Pirat, Ybert and Biance2020) is to focus on the spreading time  $\unicode[STIX]{x1D70F}$, which is essential in impact dynamics. Their experiment, presented in figure 2, gives a counter-intuitive result: the spreading lasts longer on the hierarchical surface than on the nanotextured one. The surface which is covered by the larger, thicker air pockets is thus slower at repelling drops! The difference, of the order of 20 % at high
$\unicode[STIX]{x1D70F}$, which is essential in impact dynamics. Their experiment, presented in figure 2, gives a counter-intuitive result: the spreading lasts longer on the hierarchical surface than on the nanotextured one. The surface which is covered by the larger, thicker air pockets is thus slower at repelling drops! The difference, of the order of 20 % at high  $Re$, is the first clear evidence of the influence of the friction on impact dynamics. The experiment, therefore, validates the idea that spreading is stopped by the growth of a viscous boundary layer at the solid/liquid interface (Roisman Reference Roisman2009; Eggers et al. Reference Eggers, Fontelos, Josserand and Zaleski2010; Lastakowski et al. Reference Lastakowski, Boyer, Biance, Pirat and Ybert2014). On a slippery surface,
$Re$, is the first clear evidence of the influence of the friction on impact dynamics. The experiment, therefore, validates the idea that spreading is stopped by the growth of a viscous boundary layer at the solid/liquid interface (Roisman Reference Roisman2009; Eggers et al. Reference Eggers, Fontelos, Josserand and Zaleski2010; Lastakowski et al. Reference Lastakowski, Boyer, Biance, Pirat and Ybert2014). On a slippery surface,  $\unicode[STIX]{x1D70F}$ asymptotically increases with
$\unicode[STIX]{x1D70F}$ asymptotically increases with  $b$ as
$b$ as  $\unicode[STIX]{x1D70F}=\unicode[STIX]{x1D70F}_{0}(1+\unicode[STIX]{x1D6FC})$, with
$\unicode[STIX]{x1D70F}=\unicode[STIX]{x1D70F}_{0}(1+\unicode[STIX]{x1D6FC})$, with  $\unicode[STIX]{x1D6FC}\sim (2b/5R)Re^{1/5}$ (with
$\unicode[STIX]{x1D6FC}\sim (2b/5R)Re^{1/5}$ (with  $R$ the drop size and
$R$ the drop size and  $\unicode[STIX]{x1D70F}_{0}$ the spreading time in absence of slip).
$\unicode[STIX]{x1D70F}_{0}$ the spreading time in absence of slip).
Martouzet et al. (Reference Martouzet, Lee, Pirat, Ybert and Biance2020) interestingly consider these results in light of the much more general question of drop impact dynamics on wetting and non-wetting substrates. The authors propose a scaling analysis based on the comparison of characteristic timescales associated either with inertia ( $\unicode[STIX]{x1D70F}_{i}=R/U$ with
$\unicode[STIX]{x1D70F}_{i}=R/U$ with  $U$ the drop impact velocity), capillarity (
$U$ the drop impact velocity), capillarity ( $\unicode[STIX]{x1D70F}_{c}=(R/U)We^{1/2}$) and viscous friction (
$\unicode[STIX]{x1D70F}_{c}=(R/U)We^{1/2}$) and viscous friction ( $\unicode[STIX]{x1D70F}_{v}=(R/U)Re^{1/5}$). They evidence three main regimes in a
$\unicode[STIX]{x1D70F}_{v}=(R/U)Re^{1/5}$). They evidence three main regimes in a  $Re$–
$Re$– $We$ phase diagram, corresponding to different impact dynamics. In each regime, the spreading is governed either by the growth of a boundary layer (in the ‘viscous’ region), by the rim dynamics (in the ‘capillary’ region) or by the drop deceleration (in the ‘inertial’ region). As demonstrated by the authors, the effect of the slip is thus only visible for viscous impacts, a region defined by spreading times
$We$ phase diagram, corresponding to different impact dynamics. In each regime, the spreading is governed either by the growth of a boundary layer (in the ‘viscous’ region), by the rim dynamics (in the ‘capillary’ region) or by the drop deceleration (in the ‘inertial’ region). As demonstrated by the authors, the effect of the slip is thus only visible for viscous impacts, a region defined by spreading times  $\unicode[STIX]{x1D70F}=\unicode[STIX]{x1D70F}_{v}<\unicode[STIX]{x1D70F}_{c}$, i.e. for
$\unicode[STIX]{x1D70F}=\unicode[STIX]{x1D70F}_{v}<\unicode[STIX]{x1D70F}_{c}$, i.e. for  $Re<We^{5/2}$. This global approach disentangles the existing models of drop spreading and provides a general framework for the analysis of drop impact dynamics. More generally, it demonstrates that the regime of impact should be considered with care, as it might dramatically influence the physics of spreading.
$Re<We^{5/2}$. This global approach disentangles the existing models of drop spreading and provides a general framework for the analysis of drop impact dynamics. More generally, it demonstrates that the regime of impact should be considered with care, as it might dramatically influence the physics of spreading.
3 Future
The work of Martouzet et al. (Reference Martouzet, Lee, Pirat, Ybert and Biance2020) shows that the subtle interplay between wetting and slip generates unexpected effects, such as an effective reduction of water-repellency by hierarchical textures, which are the most lubricated superhydrophobic materials. Is all then lost for the hierarchical lotus leaf? Not necessarily: typical raindrops impact at high Reynolds number, typically  $Re>1000$. The spreading dynamics of the drops are then much more likely governed by the rim formation than by friction. And indeed, the authors find a converse effect in this region of the phase diagram: the spreading time on the hierarchical surface is reduced by 30 % compared with a smooth surface. This interesting and surprising additional observation surely deserves a more in-depth analysis. On another note, this original approach at the interface between wetting and drag reduction opens many questions. One might wonder, for example: What is the impact of the slip on the receding and take-off of droplets? Would a larger slip length facilitate the evacuation of viscous rolling droplets, which are usually much harder to repel than water?
$Re>1000$. The spreading dynamics of the drops are then much more likely governed by the rim formation than by friction. And indeed, the authors find a converse effect in this region of the phase diagram: the spreading time on the hierarchical surface is reduced by 30 % compared with a smooth surface. This interesting and surprising additional observation surely deserves a more in-depth analysis. On another note, this original approach at the interface between wetting and drag reduction opens many questions. One might wonder, for example: What is the impact of the slip on the receding and take-off of droplets? Would a larger slip length facilitate the evacuation of viscous rolling droplets, which are usually much harder to repel than water?

 $b$ of
$b$ of  ${\sim}10~\unicode[STIX]{x03BC}\text{m}$ (defined as the distance below the top of the pillars for which the velocity profile of a flowing liquid vanishes), 1000 times higher than on hydrophobic materials. (c) Drop bouncing on a non-wetting surface.
${\sim}10~\unicode[STIX]{x03BC}\text{m}$ (defined as the distance below the top of the pillars for which the velocity profile of a flowing liquid vanishes), 1000 times higher than on hydrophobic materials. (c) Drop bouncing on a non-wetting surface.
 $b=35~\unicode[STIX]{x03BC}\text{m}$. (b) Image of the nanotextured surface, with
$b=35~\unicode[STIX]{x03BC}\text{m}$. (b) Image of the nanotextured surface, with  $b=8~\unicode[STIX]{x03BC}\text{m}$. (c) Effect of the slipperiness on the non-dimensional spreading time
$b=8~\unicode[STIX]{x03BC}\text{m}$. (c) Effect of the slipperiness on the non-dimensional spreading time  $\unicode[STIX]{x1D70F}/\unicode[STIX]{x1D70F}_{0}$ on two superhydrophobic surfaces, with different slip lengths: in blue,
$\unicode[STIX]{x1D70F}/\unicode[STIX]{x1D70F}_{0}$ on two superhydrophobic surfaces, with different slip lengths: in blue,  $b=35~\unicode[STIX]{x03BC}\text{m}$ and in red
$b=35~\unicode[STIX]{x03BC}\text{m}$ and in red  $b=8~\unicode[STIX]{x03BC}\text{m}$. Here,
$b=8~\unicode[STIX]{x03BC}\text{m}$. Here,  $\unicode[STIX]{x1D70F}_{0}$ is the spreading time on a smooth hydrophilic silicone substrate. From Martouzet et al. (2020).
$\unicode[STIX]{x1D70F}_{0}$ is the spreading time on a smooth hydrophilic silicone substrate. From Martouzet et al. (2020).


1 Introduction
By observing nature on a misty morning, one will notice that a number of plants are naturally engineered to repel water. Tiny droplets appear as shiny marbles which glide at the faintest quivering of the leaves. This remarkable feature is derived from the presence of a micrometre- or nanometre-scaled hydrophobic roughness on the surface (Neinhuis & Barthlott Reference Neinhuis and Barthlott1997). In the presence of water, large pockets of air remain entrapped between the textures: the rough material thus behaves as if it had a hybrid surface made of both solid and gas. Air is thus at the heart of two highly unusual attributes of the so-called superhydrophobic materials: extreme water-repellency and large-scale slipperiness. While water-repellency is captured by the contact angle of droplets, typically higher than $160^{\circ }$ (figure 1a), the amplitude of the slippage is measured through the slip length
$160^{\circ }$ (figure 1a), the amplitude of the slippage is measured through the slip length  $b$ – the extrapolated distance where the liquid tangential velocity vanishes (figure 1b). This length
$b$ – the extrapolated distance where the liquid tangential velocity vanishes (figure 1b). This length  $b$, which is typically molecular on hydrophilic materials and of the order of 10 nm on hydrophobic surfaces, is multiplied by a factor of 1000 on superhydrophobic substrates. The two distinct properties of these materials have attracted the attention of different communities, drawn either by the unusual dynamics of drops deposited on the surfaces (Blossey Reference Blossey2003) or by the drag-reduction potential of the immersed solids (Rothstein Reference Rothstein2010).
$b$, which is typically molecular on hydrophilic materials and of the order of 10 nm on hydrophobic surfaces, is multiplied by a factor of 1000 on superhydrophobic substrates. The two distinct properties of these materials have attracted the attention of different communities, drawn either by the unusual dynamics of drops deposited on the surfaces (Blossey Reference Blossey2003) or by the drag-reduction potential of the immersed solids (Rothstein Reference Rothstein2010).
Figure 1. Some properties of superhydrophobic surfaces. (a) Non-wetting: droplets deposited on the surface keep the spherical shape they have in air. (b) Large-scale slipperiness: surfaces covered with water are extremely slippery, with a slip length $b$ of
$b$ of  ${\sim}10~\unicode[STIX]{x03BC}\text{m}$ (defined as the distance below the top of the pillars for which the velocity profile of a flowing liquid vanishes), 1000 times higher than on hydrophobic materials. (c) Drop bouncing on a non-wetting surface.
${\sim}10~\unicode[STIX]{x03BC}\text{m}$ (defined as the distance below the top of the pillars for which the velocity profile of a flowing liquid vanishes), 1000 times higher than on hydrophobic materials. (c) Drop bouncing on a non-wetting surface.
While a nanostructure alone is enough to promote excellent water repellency, most plants and insects possess a hierarchical structure. The celebrated lotus leaf, for example, is covered with $20~\unicode[STIX]{x03BC}\text{m}$ tall papillae, themselves covered with waxy nano-structures at the scale of 100 nm. On the one hand, the presence of a large texture can be seen as detrimental for the lotus leaf: it makes it sensitive to condensation (Cheng & Rodak Reference Cheng and Rodak2005) and to droplet intrusion at impact (Deng et al. Reference Deng, Varanasi, Hsu, Bhate, Keimel, Stein and Blohm2009). On the other hand, it does make the surface extremely slippery: tall pillars separated by a relatively large distance, generate giant slip lengths of the order of
$20~\unicode[STIX]{x03BC}\text{m}$ tall papillae, themselves covered with waxy nano-structures at the scale of 100 nm. On the one hand, the presence of a large texture can be seen as detrimental for the lotus leaf: it makes it sensitive to condensation (Cheng & Rodak Reference Cheng and Rodak2005) and to droplet intrusion at impact (Deng et al. Reference Deng, Varanasi, Hsu, Bhate, Keimel, Stein and Blohm2009). On the other hand, it does make the surface extremely slippery: tall pillars separated by a relatively large distance, generate giant slip lengths of the order of  $10~\unicode[STIX]{x03BC}\text{m}$ (Ybert et al. Reference Ybert, Barentin, Cottin-Bizonne, Joseph and Bocquet2007). One might then wonder: What role does the slip play when the leaf is lashed by rain? This original question is the starting point of the work of Martouzet et al. (Reference Martouzet, Lee, Pirat, Ybert and Biance2020), whose approach also sheds new light onto a much wider and debated issue on the role of friction on the impact dynamics of droplets on both wetting and non-wetting substrates.
$10~\unicode[STIX]{x03BC}\text{m}$ (Ybert et al. Reference Ybert, Barentin, Cottin-Bizonne, Joseph and Bocquet2007). One might then wonder: What role does the slip play when the leaf is lashed by rain? This original question is the starting point of the work of Martouzet et al. (Reference Martouzet, Lee, Pirat, Ybert and Biance2020), whose approach also sheds new light onto a much wider and debated issue on the role of friction on the impact dynamics of droplets on both wetting and non-wetting substrates.
2 Overview
Martouzet et al. (Reference Martouzet, Lee, Pirat, Ybert and Biance2020) focus on one of the most emblematic properties of non-wetting surfaces: their ability to repel impacting drops (figure 1c). An important parameter here is the time the droplets spend in contact with the substrate, which determines the amount of mechanical and thermal exchange with the solid. In practical problems, such as the freezing of aeroplane wings, it is crucial to make the contact time as short as possible. Recent studies have demonstrated that the addition of well-chosen textures dramatically decrease the recoiling time, leading to a contact time reduction by a factor of two or more (Bird et al. Reference Bird, Dhiman, Kwon and Varanasi2013; Liu et al. Reference Liu, Moevius, Xu, Qian, Yeomans and Wang2014; Gauthier et al. Reference Gauthier, Symon, Clanet and Quéré2015). It is much harder, however, to reduce the duration of the spreading. Numerous experiments and simulations show that the first stage of impact is only marginally influenced by the surface wetting properties, or by the impact velocity of the droplet. Despite numerous studies, the physical parameters that limit the spreading are still being discussed. More importantly, conflicting theoretical models (Clanet et al. Reference Clanet, Béguin, Richard and Quéré2004; Roisman Reference Roisman2009; Eggers et al. Reference Eggers, Fontelos, Josserand and Zaleski2010) show reasonably good agreement with both simulations and experiments (see Josserand & Thoroddsen (Reference Josserand and Thoroddsen2016) for a review). In their recent paper, Martouzet et al. (Reference Martouzet, Lee, Pirat, Ybert and Biance2020) tackle experimentally and theoretically this complex problem. Through a clever choice of surfaces, they clearly evidence the role of a lubricating air layer on the impact. They present their results in a global phase diagram in terms of Reynolds ( $Re$) and Weber (
$Re$) and Weber ( $We$) numbers (which compare inertial forces, respectively, to viscous and surface tension forces) – an original approach which reconciles the theoretical models to the spreading dynamics.
$We$) numbers (which compare inertial forces, respectively, to viscous and surface tension forces) – an original approach which reconciles the theoretical models to the spreading dynamics.
Figure 2. (a) Scanning electron microscope image of the slippery hierarchical surface, with $b=35~\unicode[STIX]{x03BC}\text{m}$. (b) Image of the nanotextured surface, with
$b=35~\unicode[STIX]{x03BC}\text{m}$. (b) Image of the nanotextured surface, with  $b=8~\unicode[STIX]{x03BC}\text{m}$. (c) Effect of the slipperiness on the non-dimensional spreading time
$b=8~\unicode[STIX]{x03BC}\text{m}$. (c) Effect of the slipperiness on the non-dimensional spreading time  $\unicode[STIX]{x1D70F}/\unicode[STIX]{x1D70F}_{0}$ on two superhydrophobic surfaces, with different slip lengths: in blue,
$\unicode[STIX]{x1D70F}/\unicode[STIX]{x1D70F}_{0}$ on two superhydrophobic surfaces, with different slip lengths: in blue,  $b=35~\unicode[STIX]{x03BC}\text{m}$ and in red
$b=35~\unicode[STIX]{x03BC}\text{m}$ and in red  $b=8~\unicode[STIX]{x03BC}\text{m}$. Here,
$b=8~\unicode[STIX]{x03BC}\text{m}$. Here,  $\unicode[STIX]{x1D70F}_{0}$ is the spreading time on a smooth hydrophilic silicone substrate. From Martouzet et al. (Reference Martouzet, Lee, Pirat, Ybert and Biance2020).
$\unicode[STIX]{x1D70F}_{0}$ is the spreading time on a smooth hydrophilic silicone substrate. From Martouzet et al. (Reference Martouzet, Lee, Pirat, Ybert and Biance2020).
To disentangle the effects of wetting and friction, Martouzet et al. (Reference Martouzet, Lee, Pirat, Ybert and Biance2020) compare drop impact dynamics on two surfaces with identical non-wetting properties but with very different textures. Scanning electron microscope images of the substrates are presented in figure 2: the first surface (figure 2a) has a hierarchical structure, similar to the lotus leaf, made of tall pillars covered with nanometre-sized hairs. The large texture confers to the surface an extreme slipperiness, with a slip length $b=35~\unicode[STIX]{x03BC}\text{m}$. The second surface (figure 2b) is covered with a unique texture at a smaller scale, associated with a moderate slip (
$b=35~\unicode[STIX]{x03BC}\text{m}$. The second surface (figure 2b) is covered with a unique texture at a smaller scale, associated with a moderate slip ( $b=8~\unicode[STIX]{x03BC}\text{m}$). An originality of the work of Martouzet et al. (Reference Martouzet, Lee, Pirat, Ybert and Biance2020) is to focus on the spreading time
$b=8~\unicode[STIX]{x03BC}\text{m}$). An originality of the work of Martouzet et al. (Reference Martouzet, Lee, Pirat, Ybert and Biance2020) is to focus on the spreading time  $\unicode[STIX]{x1D70F}$, which is essential in impact dynamics. Their experiment, presented in figure 2, gives a counter-intuitive result: the spreading lasts longer on the hierarchical surface than on the nanotextured one. The surface which is covered by the larger, thicker air pockets is thus slower at repelling drops! The difference, of the order of 20 % at high
$\unicode[STIX]{x1D70F}$, which is essential in impact dynamics. Their experiment, presented in figure 2, gives a counter-intuitive result: the spreading lasts longer on the hierarchical surface than on the nanotextured one. The surface which is covered by the larger, thicker air pockets is thus slower at repelling drops! The difference, of the order of 20 % at high  $Re$, is the first clear evidence of the influence of the friction on impact dynamics. The experiment, therefore, validates the idea that spreading is stopped by the growth of a viscous boundary layer at the solid/liquid interface (Roisman Reference Roisman2009; Eggers et al. Reference Eggers, Fontelos, Josserand and Zaleski2010; Lastakowski et al. Reference Lastakowski, Boyer, Biance, Pirat and Ybert2014). On a slippery surface,
$Re$, is the first clear evidence of the influence of the friction on impact dynamics. The experiment, therefore, validates the idea that spreading is stopped by the growth of a viscous boundary layer at the solid/liquid interface (Roisman Reference Roisman2009; Eggers et al. Reference Eggers, Fontelos, Josserand and Zaleski2010; Lastakowski et al. Reference Lastakowski, Boyer, Biance, Pirat and Ybert2014). On a slippery surface,  $\unicode[STIX]{x1D70F}$ asymptotically increases with
$\unicode[STIX]{x1D70F}$ asymptotically increases with  $b$ as
$b$ as  $\unicode[STIX]{x1D70F}=\unicode[STIX]{x1D70F}_{0}(1+\unicode[STIX]{x1D6FC})$, with
$\unicode[STIX]{x1D70F}=\unicode[STIX]{x1D70F}_{0}(1+\unicode[STIX]{x1D6FC})$, with  $\unicode[STIX]{x1D6FC}\sim (2b/5R)Re^{1/5}$ (with
$\unicode[STIX]{x1D6FC}\sim (2b/5R)Re^{1/5}$ (with  $R$ the drop size and
$R$ the drop size and  $\unicode[STIX]{x1D70F}_{0}$ the spreading time in absence of slip).
$\unicode[STIX]{x1D70F}_{0}$ the spreading time in absence of slip).
Martouzet et al. (Reference Martouzet, Lee, Pirat, Ybert and Biance2020) interestingly consider these results in light of the much more general question of drop impact dynamics on wetting and non-wetting substrates. The authors propose a scaling analysis based on the comparison of characteristic timescales associated either with inertia ( $\unicode[STIX]{x1D70F}_{i}=R/U$ with
$\unicode[STIX]{x1D70F}_{i}=R/U$ with  $U$ the drop impact velocity), capillarity (
$U$ the drop impact velocity), capillarity ( $\unicode[STIX]{x1D70F}_{c}=(R/U)We^{1/2}$) and viscous friction (
$\unicode[STIX]{x1D70F}_{c}=(R/U)We^{1/2}$) and viscous friction ( $\unicode[STIX]{x1D70F}_{v}=(R/U)Re^{1/5}$). They evidence three main regimes in a
$\unicode[STIX]{x1D70F}_{v}=(R/U)Re^{1/5}$). They evidence three main regimes in a  $Re$–
$Re$– $We$ phase diagram, corresponding to different impact dynamics. In each regime, the spreading is governed either by the growth of a boundary layer (in the ‘viscous’ region), by the rim dynamics (in the ‘capillary’ region) or by the drop deceleration (in the ‘inertial’ region). As demonstrated by the authors, the effect of the slip is thus only visible for viscous impacts, a region defined by spreading times
$We$ phase diagram, corresponding to different impact dynamics. In each regime, the spreading is governed either by the growth of a boundary layer (in the ‘viscous’ region), by the rim dynamics (in the ‘capillary’ region) or by the drop deceleration (in the ‘inertial’ region). As demonstrated by the authors, the effect of the slip is thus only visible for viscous impacts, a region defined by spreading times  $\unicode[STIX]{x1D70F}=\unicode[STIX]{x1D70F}_{v}<\unicode[STIX]{x1D70F}_{c}$, i.e. for
$\unicode[STIX]{x1D70F}=\unicode[STIX]{x1D70F}_{v}<\unicode[STIX]{x1D70F}_{c}$, i.e. for  $Re<We^{5/2}$. This global approach disentangles the existing models of drop spreading and provides a general framework for the analysis of drop impact dynamics. More generally, it demonstrates that the regime of impact should be considered with care, as it might dramatically influence the physics of spreading.
$Re<We^{5/2}$. This global approach disentangles the existing models of drop spreading and provides a general framework for the analysis of drop impact dynamics. More generally, it demonstrates that the regime of impact should be considered with care, as it might dramatically influence the physics of spreading.
3 Future
The work of Martouzet et al. (Reference Martouzet, Lee, Pirat, Ybert and Biance2020) shows that the subtle interplay between wetting and slip generates unexpected effects, such as an effective reduction of water-repellency by hierarchical textures, which are the most lubricated superhydrophobic materials. Is all then lost for the hierarchical lotus leaf? Not necessarily: typical raindrops impact at high Reynolds number, typically $Re>1000$. The spreading dynamics of the drops are then much more likely governed by the rim formation than by friction. And indeed, the authors find a converse effect in this region of the phase diagram: the spreading time on the hierarchical surface is reduced by 30 % compared with a smooth surface. This interesting and surprising additional observation surely deserves a more in-depth analysis. On another note, this original approach at the interface between wetting and drag reduction opens many questions. One might wonder, for example: What is the impact of the slip on the receding and take-off of droplets? Would a larger slip length facilitate the evacuation of viscous rolling droplets, which are usually much harder to repel than water?
$Re>1000$. The spreading dynamics of the drops are then much more likely governed by the rim formation than by friction. And indeed, the authors find a converse effect in this region of the phase diagram: the spreading time on the hierarchical surface is reduced by 30 % compared with a smooth surface. This interesting and surprising additional observation surely deserves a more in-depth analysis. On another note, this original approach at the interface between wetting and drag reduction opens many questions. One might wonder, for example: What is the impact of the slip on the receding and take-off of droplets? Would a larger slip length facilitate the evacuation of viscous rolling droplets, which are usually much harder to repel than water?
Declaration of interests
The author reports no conflicts of interest.