Hostname: page-component-745bb68f8f-g4j75 Total loading time: 0 Render date: 2025-01-11T00:08:34.908Z Has data issue: false hasContentIssue false
  • English
  • Français

Slender-body theory for the generation of micrometre-sized emulsions through tip streaming

Published online by Cambridge University Press:  30 March 2012

E. Castro-Hernández ,
F. Campo-Cortés  and
José Manuel Gordillo
E. Castro-Hernández
Affiliation:
Área de Mecánica de Fluidos, Departamento de Ingenería Aeroespacial y Mecánica de Fluidos, Universidad de Sevilla, Avenida de los Descubrimientos s/n, 41092 Sevilla, Spain
F. Campo-Cortés
Affiliation:
Área de Mecánica de Fluidos, Departamento de Ingenería Aeroespacial y Mecánica de Fluidos, Universidad de Sevilla, Avenida de los Descubrimientos s/n, 41092 Sevilla, Spain
José Manuel Gordillo*
Affiliation:
Área de Mecánica de Fluidos, Departamento de Ingenería Aeroespacial y Mecánica de Fluidos, Universidad de Sevilla, Avenida de los Descubrimientos s/n, 41092 Sevilla, Spain
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We report experiments in which a flow rate of a fluid with a viscosity 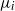discharges into an immiscible liquid of viscosity 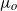that flows in parallel with the axis of the injector. When the outer capillary number verifies the condition 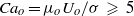, where and 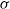indicate, respectively, the outer velocity and the interfacial tension coefficient, and if the inner-to-outer velocity ratio is such that 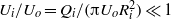, with the inner radius of the injector, a jet is formed with the same type of cone–jet geometry as predicted by the numerical results of Suryo & Basaran (Phys. Fluids, vol. 18, 2006, p. 082102). For extremely low values of the velocity ratio 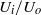, we find that the diameter of the jet emanating from the tip of the cone is so small that drops with sizes below can be formed. We also show that, through this simple method, concentrated emulsions composed of micrometre-sized drops with a narrow size distribution can be generated. Moreover, thanks to the information extracted from numerical simulations of boundary-integral type and using the slender-body approximation due to Taylor (Proceedings of the 11th International Congress of Applied Mechanics, Munich, 1964, pp. 790–796), we deduce a third-order, ordinary differential equation that predicts, for arbitrary values of the three dimensionless numbers that control this physical situation, namely, 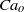, 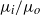and 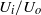, the shape of the jet and the sizes of the drops generated. Most interestingly, the influence of the geometry of the injector system on the jet shape and drop size enters explicitly into the third-order differential equation through two functions that can be easily calculated numerically. Therefore, our theory can be used as an efficient tool for the design of new emulsification devices.

JFM classification

Drops and Bubbles: Drops and Bubbles Low-Reynolds-number flows: Low-Reynolds-number flows Micro-/Nano-fluid dynamics: Microfluidics
Type
Papers
Information
Journal of Fluid Mechanics , Volume 698 , 10 May 2012 , pp. 423 - 445
Copyright
Copyright © Cambridge University Press 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Abramowitz, M. & Stegun, I. A. 1970 Handbook of Mathematical Functions. Dover.Google Scholar
2. Acrivos, A. & Lo, T. S. 1977 Deformation and breakup of a single slender drop in an extensional flow. J. Fluid Mech. 86, 641–672.CrossRefGoogle Scholar
3. Anna, S. L., Bontoux, N. & Stone, H. A. 2003 Formation of dispersions using flow focusing in microchannels. Appl. Phys. Lett. 82, 364–366.CrossRefGoogle Scholar
4. Barrero, A. & Loscertales, I. G. 2007 Micro- and nanoparticles via capillary flows. Annu. Rev. Fluid Mech. 39, 89–106.CrossRefGoogle Scholar
5. Basaran, O. A. 2002 Small-scale free surface flows with breakup: drop formation and emerging applications. AIChE J. 48, 1842–1848.CrossRefGoogle Scholar
6. Buckmaster, J. D. 1971 Pointed bubbles in slow viscous flow. J. Fluid Mech. 55, 385–400.CrossRefGoogle Scholar
7. Castro-Hernández, E. 2011 Analysis of the mechanisms of generation and breakup of bubbles and droplets in gas–liquid and liquid–liquid streams. PhD thesis, Universidad de Sevilla.Google Scholar
8. Castro-Hernández, E., Gundabala, V., Fernández-Nieves, A. & Gordillo, J. M. 2009 Scaling the drop size in coflow experiments. New J. Phys. 11, 075021.CrossRefGoogle Scholar
9. Castro-Hernández, E., Hoeve, W., Lohse, D. & Gordillo, J. M. 2011 Microbubble generation in a co-flow device operated in a new regime. Lab on a Chip 11, 2023–2029.CrossRefGoogle Scholar
10. Fernandez de la Mora, J. 2007 The fluid dynamics of Taylor cones. Annu. Rev. Fluid Mech. 39, 217–243.CrossRefGoogle Scholar
11. Fernandez de la Mora, J. & Loscertales, I. G. 1994 The current emitted by highly conducting Taylor cones. J. Fluid. Mech. 260, 155–184.CrossRefGoogle Scholar
12. Gañán Calvo, A. M. 1998 Generation of steady liquid microthreads and micron-sized monodisperse sprays in gas streams. Phys. Rev. Lett. 80, 285–288.CrossRefGoogle Scholar
13. Gañán Calvo, A. M. & Gordillo, J. M. 2001 Perfectly monodisperse microbubbling by capillary flow focusing. Phys. Rev. Lett. 87, 274501.CrossRefGoogle ScholarPubMed
14. Garstecki, P., Gitlin, I., DiLuzio, W., Whitesides, G. M., Kumacheva, E. & Stone, H. A. 2004 Formation of monodisperse bubbles in a microfluidic flow-focusing device. Appl. Phys. Lett. 85, 2649–2651.CrossRefGoogle Scholar
15. Ladyzhenskaya, O. A. 1969 The Mathematical Theory of Viscous Incompressible Flows. Gordon & Breach.Google Scholar
16. Leal, L. G. 2007 Advanced Transport Phenomena: Fluid Mechanics and Convective Transport Processes. Cambridge University Press.CrossRefGoogle Scholar
17. Loscertales, I. G., Barrero, A., Guerrero, I., Cortijo, R., Márquez, M. & Gañán Calvo, A. M. 2002 Micro/nano encapsulation via electrified coaxial liquid jets. Science 295, 5560.CrossRefGoogle ScholarPubMed
18. Marín, A. G., Campo-Cortés, F. & Gordillo, J. M. 2009 Generation of micron-sized drops and bubbles through viscous coflows. Colloids Surf. A: Physicochem. Engng Aspects 344, 2–7.CrossRefGoogle Scholar
19. Marín, A. G., Loscertales, I. G., Márquez, M. & Barrero, A. 2007 Simple and double emulsions via coaxial jet electrosprays. Phys. Rev. Lett. 98, 014502.CrossRefGoogle ScholarPubMed
20. Pozrikidis, C. 1992 Boundary Integral and Singularity Methods for Linearized Viscous Flows. Cambridge University Press.CrossRefGoogle Scholar
21. Rallison, J. M. 1984 The deformation of small viscous drops and bubbles in shear flows. Annu. Rev. Fluid Mech. 16, 45–66.CrossRefGoogle Scholar
22. Sherwood, J. D. 1983 Tip streaming from slender drops in a nonlinear extensional flow. J. Fluid Mech. 144, 281–295.CrossRefGoogle Scholar
23. Sierou, A. & Lister, J. R. 2003 Self-similar solutions for viscous capillary pinch-off. J. Fluid Mech. 497, 381–403.CrossRefGoogle Scholar
24. Stone, H. A. 1994 Dynamics of drop deformation and breakup in viscous fluids. Annu. Rev. Fluid Mech. 26, 65–102.CrossRefGoogle Scholar
25. Stone, H. A. & Leal, L. G. 1989 Relaxation and breakup of an initially extended drop in an otherwise quiescent fluid. J. Fluid Mech. 198, 399–427.CrossRefGoogle Scholar
26. Stone, H. A., Stroock, A. D. & Adjari, A. 2004 Engineering flows in small devices: microfluidics toward a lab-on-a-chip. Annu. Rev. Fluid Mech. 36, 381–411.CrossRefGoogle Scholar
27. Suryo, R. & Basaran, O. A. 2006 Tip streaming from a liquid drop forming from a tube in a co-flowing outer fluid. Phys. Fluids 18, 082102.CrossRefGoogle Scholar
28. Taylor, G. I. 1964 Conical free surfaces and fluid interfaces. In Proceedings of the 11th International Congress of Applied Mechanics, Munich (ed. Batchelor, G. K. ), pp. 790–796. Springer.Google Scholar
29. Tomotika, S. 1935 On the instability of a cylindrical thread of a viscous liquid surrounded by another viscous fluid. Proc. R. Soc. Lond. 150, 322–337.Google Scholar
30. Utada, A. S., Fernández-Nieves, A., Stone, H. A. & Weitz, D. 2007 Dripping to jetting transitions in co-flowing liquid streams. Phys. Rev. Lett. 99, 094502.CrossRefGoogle Scholar
31. Wong, H., Rumschitki, D. & Maldarelli, C. 1997 Theory and experiment on the low-Reynolds-number expansion and contraction of a bubble pinned at a submerged tip. J. Fluid Mech. 356, 93–124.CrossRefGoogle Scholar
32. Zhang, W. W. 2004 Viscous entrainment from a nozzle: singular liquid spouts. Phys. Rev. Lett. 93, 184502.CrossRefGoogle ScholarPubMed
33. Zhang, D. F. & Stone, H. A. 1997 Drop formation in viscous flows at a vertical capillary tube. Phys. Fluids 9, 2234–2242.CrossRefGoogle Scholar