Hostname: page-component-745bb68f8f-b95js Total loading time: 0 Render date: 2025-01-09T21:37:15.596Z Has data issue: false hasContentIssue false
  • English
  • Français

Simultaneous skin friction and velocity measurements in high Reynolds number pipe and boundary layer flows

Published online by Cambridge University Press:  21 May 2019

R. Baidya Open the ORCID record for R. Baidya [Opens in a new window] ,
W. J. Baars Open the ORCID record for W. J. Baars [Opens in a new window] ,
S. Zimmerman Open the ORCID record for S. Zimmerman [Opens in a new window] ,
M. Samie Open the ORCID record for M. Samie [Opens in a new window] ,
R. J. Hearst Open the ORCID record for R. J. Hearst [Opens in a new window] ,
E. Dogan ,
L. Mascotelli ,
X. Zheng ,
G. Bellani  and
A. Talamelli
...Show all authors
R. Baidya*
Affiliation:
Department of Mechanical Engineering, University of Melbourne, Melbourne, Victoria 3010, Australia
W. J. Baars
Affiliation:
Department of Mechanical Engineering, University of Melbourne, Melbourne, Victoria 3010, Australia
S. Zimmerman
Affiliation:
Department of Mechanical Engineering, University of Melbourne, Melbourne, Victoria 3010, Australia
M. Samie
Affiliation:
Department of Mechanical Engineering, University of Melbourne, Melbourne, Victoria 3010, Australia
R. J. Hearst
Affiliation:
Aerodynamics and Flight Mechanics Research Group, University of Southampton, HampshireSO17 1BJ, UK Department of Energy and Process Engineering, Norwegian University of Science and Technology, Trondheim, NO-7491, Norway
E. Dogan
Affiliation:
Aerodynamics and Flight Mechanics Research Group, University of Southampton, HampshireSO17 1BJ, UK
L. Mascotelli
Affiliation:
Department of Industrial Engineering, CIRI Aerospace, Alma Mater Studiorum, Università di Bologna, 47100 Forlì, Italy
X. Zheng
Affiliation:
Department of Industrial Engineering, CIRI Aerospace, Alma Mater Studiorum, Università di Bologna, 47100 Forlì, Italy
G. Bellani
Affiliation:
Department of Industrial Engineering, CIRI Aerospace, Alma Mater Studiorum, Università di Bologna, 47100 Forlì, Italy
A. Talamelli
Affiliation:
Department of Industrial Engineering, CIRI Aerospace, Alma Mater Studiorum, Università di Bologna, 47100 Forlì, Italy
B. Ganapathisubramani
Affiliation:
Aerodynamics and Flight Mechanics Research Group, University of Southampton, HampshireSO17 1BJ, UK
N. Hutchins
Affiliation:
Department of Mechanical Engineering, University of Melbourne, Melbourne, Victoria 3010, Australia
I. Marusic
Affiliation:
Department of Mechanical Engineering, University of Melbourne, Melbourne, Victoria 3010, Australia
J. Klewicki
Affiliation:
Department of Mechanical Engineering, University of Melbourne, Melbourne, Victoria 3010, Australia
J. P. Monty
Affiliation:
Department of Mechanical Engineering, University of Melbourne, Melbourne, Victoria 3010, Australia
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Streamwise velocity and wall-shear stress are acquired simultaneously with a hot-wire and an array of azimuthal/spanwise-spaced skin friction sensors in large-scale pipe and boundary layer flow facilities at high Reynolds numbers. These allow for a correlation analysis on a per-scale basis between the velocity and reference skin friction signals to reveal which velocity-based turbulent motions are stochastically coherent with turbulent skin friction. In the logarithmic region, the wall-attached structures in both the pipe and boundary layers show evidence of self-similarity, and the range of scales over which the self-similarity is observed decreases with an increasing azimuthal/spanwise offset between the velocity and the reference skin friction signals. The present empirical observations support the existence of a self-similar range of wall-attached turbulence, which in turn are used to extend the model of Baars et al. (J. Fluid Mech., vol. 823, p. R2) to include the azimuthal/spanwise trends. Furthermore, the region where the self-similarity is observed correspond with the wall height where the mean momentum equation formally admits a self-similar invariant form, and simultaneously where the mean and variance profiles of the streamwise velocity exhibit logarithmic dependence. 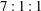The experimental observations suggest that the self-similar wall-attached structures follow an aspect ratio of $7:1:1$ in the streamwise, spanwise and wall-normal directions, respectively.

JFM classification

Boundary Layers: Boundary layer structure Boundary Layers: Pipe flow boundary layer Turbulent Flows: Turbulent boundary layers
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 871 , 25 July 2019 , pp. 377 - 400
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Adrian, R. J., Meinhart, C. D. & Tomkins, C. D. 2000 Vortex organization in the outer region of the turbulent boundary layer. J. Fluid Mech. 422, 1–54.Google Scholar
Ahn, J., Lee, J. H., Lee, J., Kang, J. & Sung, H. J. 2015 Direct numerical simulation of a 30R long turbulent pipe flow at Re 𝜏 = 3008. Phys. Fluids 27 (6), 065110.Google Scholar
Alfredsson, P. H., Johansson, A. V., Haritonidis, J. H. & Eckelmann, H. 1988 The fluctuating wall-shear stress and the velocity field in the viscous sublayer. Phys. Fluids 31 (5), 1026–1033.Google Scholar
Baars, W. J., Hutchins, N. & Marusic, I. 2016 Spectral stochastic estimation of high-Reynolds-number wall-bounded turbulence for a refined inner-outer interaction model. Phys. Rev. Fluids 1 (5), 054406.Google Scholar
Baars, W. J., Hutchins, N. & Marusic, I. 2017 Self-similarity of wall-attached turbulence in boundary layers. J. Fluid Mech. 823, R2.Google Scholar
Baidya, R., Philip, J., Hutchins, N., Monty, J. P. & Marusic, I. 2017 Distance-from-the-wall scaling of turbulent motions in wall-bounded flows. Phys. Fluids 29 (2), 020712.Google Scholar
Bellani, G. & Talamelli, A. 2016 The final design of the long pipe in CICLoPE. In Progress in Turbulence VI, pp. 205–209. Springer.Google Scholar
Bendat, J. S. & Piersol, A. G. 2010 Random Data: Analysis and Measurement Procedures, 4th edn. John Wiley & Sons.Google Scholar
Chandran, D., Baidya, R., Monty, J. P. & Marusic, I. 2017 Two-dimensional energy spectra in high-Reynolds-number turbulent boundary layers. J. Fluid Mech. 826, R1.Google Scholar
Chauhan, K., Philip, J., de Silva, C. M., Hutchins, N. & Marusic, I. 2014 The turbulent/non-turbulent interface and entrainment in a boundary layer. J. Fluid Mech. 742, 119–151.Google Scholar
Chung, D., Marusic, I., Monty, J. P., Vallikivi, M. & Smits, A. J. 2015 On the universality of inertial energy in the log layer of turbulent boundary layer and pipe flows. Exp. Fluids 56 (7), 1–10.Google Scholar
Davidson, P. A. & Krogstad, P.-Å. 2008 On the deficiency of even-order structure functions as inertial-range diagnostics. J. Fluid Mech. 602, 287–302.Google Scholar
del Álamo, J. C. & Jiménez, J. 2009 Estimation of turbulent convection velocities and corrections to Taylor’s approximation. J. Fluid Mech. 640, 5–26.Google Scholar
del Álamo, J. C., Jiménez, J., Zandonade, P. & Moser, R. D. 2004 Scaling of the energy spectra of turbulent channels. J. Fluid Mech. 500, 135–144.Google Scholar
del Álamo, J. C., Jiménez, J., Zandonade, P. & Moser, R. D. 2006 Self-similar vortex clusters in the turbulent logarithmic region. J. Fluid Mech. 561, 329–358.Google Scholar
Hellström, L. H., Marusic, I. & Smits, A. J. 2016 Self-similarity of the large-scale motions in turbulent pipe flow. J. Fluid Mech. 792, R1.Google Scholar
Hutchins, N. & Marusic, I. 2007a Evidence of very long meandering features in the logarithmic region of turbulent boundary layers. J. Fluid Mech. 579, 1–28.Google Scholar
Hutchins, N. & Marusic, I. 2007b Large-scale influences in near-wall turbulence. Phil. Trans. R. Soc. Lond. A 365, 647–664.Google Scholar
Hutchins, N., Monty, J. P., Ganapathisubramani, B., Ng, H. C. H. & Marusic, I. 2011 Three-dimensional conditional structure of a high-Reynolds-number turbulent boundary layer. J. Fluid Mech. 673, 255–285.Google Scholar
Hutchins, N., Nickels, T. B., Marusic, I. & Chong, M. S. 2009 Hot-wire spatial resolution issues in wall-bounded turbulence. J. Fluid Mech. 635, 103–136.Google Scholar
Hwang, Y. 2015 Statistical structure of self-sustaining attached eddies in turbulent channel flow. J. Fluid Mech. 767, 254–289.Google Scholar
Jiménez, J. 2011 Cascades in wall-bounded turbulence. Annu. Rev. Fluid Mech. 44 (1), 27.Google Scholar
Jiménez, J., del Álamo, J. C. & Flores, O. 2004 The large-scale dynamics of near-wall turbulence. J. Fluid Mech. 505, 179–199.Google Scholar
Kim, K. C. & Adrian, R. J. 1999 Very large-scale motion in the outer layer. Phys. Fluids 11 (2), 417–422.Google Scholar
Krug, D., Baars, W. J., Hutchins, N. & Marusic, I. 2019 Vertical coherence of turbulence in the atmospheric surface layer: connecting the hypotheses of Townsend and Davenport. Boundary-Layer Meteorol. doi:10.1007/s10546-019-00445-4.Google Scholar
Kwon, Y.2016 The quiesecent core of turbulent channel and pipe flows. PhD thesis, University of Melbourne.Google Scholar
Kwon, Y. S., Hutchins, N. & Monty, J. P. 2016 On the use of the Reynolds decomposition in the intermittent region of turbulent boundary layers. J. Fluid Mech. 794, 5–16.Google Scholar
Ligrani, P. M. & Bradshaw, P. 1987 Spatial resolution and measurments of turbulence in the viscous sublayer using subminature hot-wire probes. Exp. Fluids 5, 407–417.Google Scholar
Marusic, I., McKeon, B. J., Monkewitz, P. A., Nagib, H. M., Smits, A. J. & Sreenivasan, K. R. 2010 Wall-bounded turbulent flows at high Reynolds numbers: recent advances and key issues. Phys. Fluids 22 (6), 065103.Google Scholar
Marusic, I. & Monty, J. P. 2019 Attached eddy model of wall turbulence. Annu. Rev. Fluid Mech. 51, 49–74.Google Scholar
Marusic, I., Monty, J. P., Hultmark, M. & Smits, A. J. 2013 On the logarithmic region in wall turbulence. J. Fluid Mech. 716, 716.Google Scholar
Mathis, R., Hutchins, N. & Marusic, I. 2009 Large-scale amplitude modulation of the small-scale structures in turbulent boundary layers. J. Fluid Mech. 628, 311–337.Google Scholar
Monty, J. P.2005 Developments in smooth wall turbulent duct flows. PhD thesis, The University of Melbourne.Google Scholar
Monty, J. P., Hutchins, N., Ng, H. C. H., Marusic, I. & Chong, M. S. 2009 A comparison of turbulent pipe, channel and boundary layer flows. J. Fluid Mech. 632, 431–442.Google Scholar
Monty, J. P., Stewart, J. A., Williams, R. C. & Chong, M. S. 2007 Large-scale features in turbulent pipe and channel flows. J. Fluid Mech. 589, 147–156.Google Scholar
Morrill-Winter, C., Philip, J. & Klewicki, J. 2017 An invariant representation of mean inertia: theoretical basis for a log law in turbulent boundary layers. J. Fluid Mech. 813, 594–617.Google Scholar
Nickels, T. B., Marusic, I., Hafez, S. & Chong, M. S. 2005 Evidence of the k 1 -1 law in a high-Reynolds-number turbulent boundary layer. Phys. Rev. Lett. 95 (7), 074501.Google Scholar
Örlü, R., Fiorini, T., Segalini, A., Bellani, G., Talamelli, A. & Alfredsson, P. H. 2017 Reynolds stress scaling in pipe flow turbulence – first results from CICLoPE. Phil. Trans. R. Soc. Lond. A 375 (2089), 20160187.Google Scholar
Perry, A. E. & Chong, M. S. 1982 On the mechanism of wall turbulence. J. Fluid Mech. 119, 173–217.Google Scholar
Perry, A. E., Marusic, I. & Jones, M. B. 2002 On the streamwise evolution of turbulent boundary layers in arbitrary pressure gradients. J. Fluid Mech. 461, 61–91.Google Scholar
Piomelli, U., Balint, J.-L. & Wallace, J. M. 1989 On the validity of Taylor’s hypothesis for wall-bounded flows. Phys. Fluids 1 (3), 609–611.Google Scholar
Robinson, S. K. 1991 Coherent motions in the turbulent boundary layer. Annu. Rev. Fluid Mech. 23 (1), 601–639.Google Scholar
Sillero, J. A., Jiménez, J. & Moser, R. D. 2014 Two-point statistics for turbulent boundary layers and channels at Reynolds numbers up to 𝛿+ ≈ 2000. Phys. Fluids 26 (10), 105109.Google Scholar
de Silva, C. M., Gnanamanickam, E. P., Atkinson, C., Buchmann, N. A., Hutchins, N., Soria, J. & Marusic, I. 2014 High spatial range velocity measurements in a high Reynolds number turbulent boundary layer. Phys. Fluids 26 (2), 025117.Google Scholar
de Silva, C. M., Marusic, I., Woodcock, J. D. & Meneveau, C. 2015 Scaling of second- and higher-order structure functions in turbulent boundary layers. J. Fluid Mech. 769, 654–686.Google Scholar
Smits, A. J., McKeon, B. J. & Marusic, I. 2011 High-Reynolds number wall turbulence. Annu. Rev. Fluid Mech. 43, 353–375.Google Scholar
Talamelli, A., Persiani, F., Fransson, J. H. M., Alfredsson, P. H., Johansson, A. V., Nagib, H. M., Rüedi, J., Sreenivasan, K. R. & Monkewitz, P. A. 2009 CICLoPE – a response to the need for high Reynolds number experiments. Fluid Dyn. Res. 41 (2), 021407.Google Scholar
Taylor, G. I. 1938 The spectrum of turbulence. Proc. R. Soc. Lond. A 164 (919), 476–490.Google Scholar
Tennekes, H. & Lumley, J. L. 1972 A First Course in Turbulence. MIT Press.Google Scholar
Townsend, A. A. 1976 The Structure of Turbulent Shear Flow, 2nd edn. Cambridge University Press.Google Scholar
Wei, T., Fife, P., Klewicki, J. & McMurtry, P. 2005 Properties of the mean momentum balance in turbulent boundary layer, pipe and channel flows. J. Fluid Mech. 522, 303–327.Google Scholar
Willert, C. E., Soria, J., Stanislas, M., Klinner, J., Amili, O., Eisfelder, M., Cuvier, C., Bellani, G., Fiorini, T. & Talamelli, A. 2017 Near-wall statistics of a turbulent pipe flow at shear Reynolds numbers up to 40 000. J. Fluid Mech. 826, R5.Google Scholar
Yang, X. I. A., Baidya, R., Johnson, P., Marusic, I. & Meneveau, C. 2017 Structure function tensor scaling in the logarithmic region derived from the attached eddy model of wall-bounded turbulent flows. Phys. Rev. Fluids 2 (6), 064602.Google Scholar
Zagarola, M. V. & Smits, A. J. 1998 Mean-flow scaling of turbulent pipe flow. J. Fluid Mech. 373, 33–79.Google Scholar