Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Wong, Man Long
Livescu, Daniel
and
Lele, Sanjiva K.
2019.
High-resolution Navier-Stokes simulations of Richtmyer-Meshkov instability with reshock.
Physical Review Fluids,
Vol. 4,
Issue. 10,
Cobos-Campos, F.
and
Wouchuk, J. G.
2019.
Analytic solution for the zero-order postshock profiles when an incident planar shock hits a planar contact surface.
Physical Review E,
Vol. 100,
Issue. 3,
Zhou, Ye
Clark, Timothy T.
Clark, Daniel S.
Gail Glendinning, S.
Aaron Skinner, M.
Huntington, Channing M.
Hurricane, Omar A.
Dimits, Andris M.
and
Remington, Bruce A.
2019.
Turbulent mixing and transition criteria of flows induced by hydrodynamic instabilities.
Physics of Plasmas,
Vol. 26,
Issue. 8,
Thornber, B.
Griffond, J.
Bigdelou, P.
Boureima, I.
Ramaprabhu, P.
Schilling, O.
and
Williams, R. J. R.
2019.
Turbulent transport and mixing in the multimode narrowband Richtmyer-Meshkov instability.
Physics of Fluids,
Vol. 31,
Issue. 9,
Mohaghar, Mohammad
Carter, John
Pathikonda, Gokul
and
Ranjan, Devesh
2019.
The transition to turbulence in shock-driven mixing: effects of Mach number and initial conditions.
Journal of Fluid Mechanics,
Vol. 871,
Issue. ,
p.
595.
Noble, Christopher D.
Herzog, Josh M.
Ames, Alex M.
Oakley, Jason
Rothamer, David A.
and
Bonazza, Riccardo
2020.
High speed PLIF study of the Richtmyer–Meshkov instability upon re-shock.
Physica D: Nonlinear Phenomena,
Vol. 410,
Issue. ,
p.
132519.
Gao, Xiangyu
Bermejo-Moreno, Ivan
and
Larsson, Johan
2020.
Parametric numerical study of passive scalar mixing in shock turbulence interaction.
Journal of Fluid Mechanics,
Vol. 895,
Issue. ,
Жалнин, Руслан Викторович
Zhalnin, Ruslan V.
Масягин, Виктор Федорович
Masyagin, Victor Fedorovich
Пескова, Елизавета Евгеньевна
Peskova, Elizaveta Evgenievna
Тишкин, Владимир Федорович
and
Tishkin, Vladimir Fedorovich
2020.
Моделирование развития неустойчивости Рихтмайера-Мешкова с использованием разрывного метода Галеркина на локально-адаптивных сетках.
Математическое моделирование,
Vol. 32,
Issue. 10,
p.
34.
Livescu, Daniel
2020.
Turbulence with Large Thermal and Compositional Density Variations.
Annual Review of Fluid Mechanics,
Vol. 52,
Issue. 1,
p.
309.
Pereira, F.S.
Grinstein, F.F.
and
Israel, D.
2020.
Effect of the numerical discretization scheme in Shock-Driven turbulent mixing simulations.
Computers & Fluids,
Vol. 201,
Issue. ,
p.
104487.
Luo, Xisheng
Liu, Lili
Liang, Yu
Ding, Juchun
and
Wen, Chih-yung
2020.
Richtmyer–Meshkov instability on a dual-mode interface.
Journal of Fluid Mechanics,
Vol. 905,
Issue. ,
Groom, M.
and
Thornber, B.
2020.
The influence of initial perturbation power spectra on the growth of a turbulent mixing layer induced by Richtmyer–Meshkov instability.
Physica D: Nonlinear Phenomena,
Vol. 407,
Issue. ,
p.
132463.
Bury, Yannick
Graumer, Pierre
Jamme, Stéphane
and
Griffond, Jérôme
2020.
Turbulent transition of a gaseous mixing zone induced by the Richtmyer-Meshkov instability.
Physical Review Fluids,
Vol. 5,
Issue. 2,
El Rafei, M.
and
Thornber, B.
2020.
Numerical study and buoyancy–drag modeling of bubble and spike distances in three-dimensional spherical implosions.
Physics of Fluids,
Vol. 32,
Issue. 12,
Wadas, Michael J.
and
Johnsen, Eric
2020.
Interactions of two bubbles along a gaseous interface undergoing the Richtmyer–Meshkov instability in two dimensions.
Physica D: Nonlinear Phenomena,
Vol. 409,
Issue. ,
p.
132489.
Groom, M.
and
Thornber, B.
2021.
Reynolds number dependence of turbulence induced by the Richtmyer–Meshkov instability using direct numerical simulations.
Journal of Fluid Mechanics,
Vol. 908,
Issue. ,
Schilling, Oleg
2021.
Self-similar Reynolds-averaged mechanical–scalar turbulence models for Rayleigh–Taylor, Richtmyer–Meshkov, and Kelvin–Helmholtz instability-induced mixing in the small Atwood number limit.
Physics of Fluids,
Vol. 33,
Issue. 8,
Zhalnin, R. V.
Masyagin, V. F.
Peskova, E. E.
and
Tishkin, V. F.
2021.
Modeling the Richtmyer–Meshkov Instability Development Using the Discontinuous Galerkin Method and Locally Adaptive Meshes.
Mathematical Models and Computer Simulations,
Vol. 13,
Issue. 3,
p.
474.
Zhou, Ye
2021.
Turbulence theories and statistical closure approaches.
Physics Reports,
Vol. 935,
Issue. ,
p.
1.
Li, Xinliang
Fu, Yaowei
Yu, Changping
and
Li, Li
2021.
Statistical characteristics of turbulent mixing in spherical and cylindrical converging Richtmyer–Meshkov instabilities.
Journal of Fluid Mechanics,
Vol. 928,
Issue. ,
 $A\approx 0.7$). In the present work, a shear layer is introduced at the interface to serve as a statistically repeatable, broadband initial condition to the RMI, and the density interface is accelerated by either an
$A\approx 0.7$). In the present work, a shear layer is introduced at the interface to serve as a statistically repeatable, broadband initial condition to the RMI, and the density interface is accelerated by either an  $M=1.6$ or
$M=1.6$ or  $M=2.2$ planar shock wave. The development of the ensuing mixing layer is investigated using simultaneous planar laser-induced fluorescence (PLIF) and particle image velocimetry (PIV). PLIF images are processed to reveal the light-gas mole fraction, while PIV particle image pairs yield corresponding two-component planar velocity results. Field structure and distribution are explored through probability density functions (PDFs), and a decomposition is performed on concentration and velocity results to obtain a mean flow field and define fluctuations. Simultaneous concentration and velocity field measurements allow – for the first time in this regime – experimentally determined turbulence quantities such as Reynolds stresses, turbulent mass-flux velocities and turbulent kinetic energy to be obtained. We show that by the latest times the mixing layer has passed the turbulent threshold, and there is evidence of turbulent mixing occurring sooner for the higher Mach number case. Interface measurements show nonlinear growth with a power-law fit to the thickness data, and that integral measurements of mixing layer thickness are proportional to threshold measurements. Spectral analysis demonstrates the emergence of an inertial range with a slope
$M=2.2$ planar shock wave. The development of the ensuing mixing layer is investigated using simultaneous planar laser-induced fluorescence (PLIF) and particle image velocimetry (PIV). PLIF images are processed to reveal the light-gas mole fraction, while PIV particle image pairs yield corresponding two-component planar velocity results. Field structure and distribution are explored through probability density functions (PDFs), and a decomposition is performed on concentration and velocity results to obtain a mean flow field and define fluctuations. Simultaneous concentration and velocity field measurements allow – for the first time in this regime – experimentally determined turbulence quantities such as Reynolds stresses, turbulent mass-flux velocities and turbulent kinetic energy to be obtained. We show that by the latest times the mixing layer has passed the turbulent threshold, and there is evidence of turbulent mixing occurring sooner for the higher Mach number case. Interface measurements show nonlinear growth with a power-law fit to the thickness data, and that integral measurements of mixing layer thickness are proportional to threshold measurements. Spectral analysis demonstrates the emergence of an inertial range with a slope 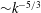 ${\sim}k^{-5/3}$ when considering both density and velocity effects in planar turbulent kinetic energy (TKE) measurements.
${\sim}k^{-5/3}$ when considering both density and velocity effects in planar turbulent kinetic energy (TKE) measurements.


