Hostname: page-component-586b7cd67f-2brh9 Total loading time: 0 Render date: 2024-11-24T18:23:25.576Z Has data issue: false hasContentIssue false
  • English
  • Français

Shape optimization of tumbling wings

Published online by Cambridge University Press:  21 February 2020

Lionel Vincent Open the ORCID record for Lionel Vincent [Opens in a new window] ,
Yucen Liu  and
Eva Kanso Open the ORCID record for Eva Kanso [Opens in a new window]
Lionel Vincent
Affiliation:
Department of Aerospace and Mechanical Engineering, University of Southern California, Los Angeles, CA 90089-1191, USA
Yucen Liu
Affiliation:
Department of Aerospace and Mechanical Engineering, University of Southern California, Los Angeles, CA 90089-1191, USA
Eva Kanso*
Affiliation:
Department of Aerospace and Mechanical Engineering, University of Southern California, Los Angeles, CA 90089-1191, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

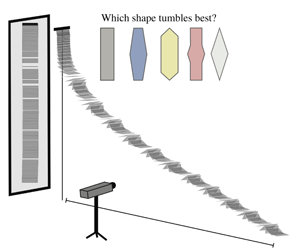
Tumbling wings are one of Nature’s many tricks to enhance the dispersal efficiency of flying seedpods. However, the interplay between the seedpod morphology and its dispersal range is not well understood. Here, we investigate the question of how planform geometry affects two-dimensional tumbling flight by designing wings of various planform and length-to-width ratios. Through a combination of experiments and modelling, we compare the wings’ flight characteristics, specifically the rotation rate and descent angle, both of which are key parameters in the wing’s ability to drift away from its initial location. Starting from the quasi-steady flight model proposed by Wang et al. (J. Fluid Mech., vol. 733, 2013, pp. 650–679), we derive theoretical predictions of the performance of wings of arbitrary planform. Upon further simplifications, we arrive at a performance index based purely on wing geometry and we use it to obtain theoretically optimal wing shapes. These optimal predictions are then tested experimentally. We conclude by discussing the advantages and limitations of the theoretical approach and its utility in informing the design of aerodynamically efficient tumbling wings.

JFM classification

Biological Fluid Dynamics: Swimming/flying Nonlinear Dynamical Systems: Low-dimensional models
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 889 , 25 April 2020 , A9
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Andersen, A., Pesavento, U. & Wang, Z. J. 2005a Analysis of transitions between fluttering, tumbling and steady descent of falling cards. J. Fluid Mech. 541, 91–104.CrossRefGoogle Scholar
Andersen, A., Pesavento, U. & Wang, Z. J. 2005b Unsteady aerodynamics of fluttering and tumbling plates. J. Fluid Mech. 541, 65–90.CrossRefGoogle Scholar
Anderson, J. D. 2001 Fundamentals of Aerodynamics, 3rd edn. McGraw-Hill Higher Education.Google Scholar
Auguste, F., Magnaudet, J. & Fabre, D. 2013 Falling styles of disks. J. Fluid Mech. 719, 388–405.CrossRefGoogle Scholar
Azuma, A. & Okuno, Y. 1987 Flight of a samara, alsomitra macrocarpa. J. Theor. Biol. 129, 263–274.CrossRefGoogle Scholar
Belmonte, A., Eisenberg, H. & Moses, E. 1998 From flutter to tumble: inertial drag and Froude similarity in falling paper. Phy. Rev. Lett. 81 (2), 345–348.CrossRefGoogle Scholar
Cummins, C., Seale, M., Macente, A., Certini, D., Mastropaolo, E., Viola, I. M. & Nakayama, N. 2018 A separated vortex ring underlies the flight of the dandelion. Nature 562, 414–418.CrossRefGoogle ScholarPubMed
Drzewiecki, S.1892 Méthode pour la détermination des éléments mécaniques des propulseurs hélicoïdaux. Association technique maritime.Google Scholar
Dupleich, P.1941 Rotation in free fall of rectangular wings of elongated shape. NACA Tech. Memo. 1201.Google Scholar
Eloy, C. 2013 On the best design for undulatory swimming. J. Fluid Mech. 717, 48–89.CrossRefGoogle Scholar
Greene, D. F. & Johnson, E. A. 1990 The aerodynamics of plumed seeds. Funct. Ecol. 4 (1), 117–125.CrossRefGoogle Scholar
Hoerner, S.1949 Aerodynamic shape of the wing tips. Tech. Rep. 5752. Air Force Materiel Command.Google Scholar
Huang, W., Liu, H., Wang, F., Wu, J. & Zhang, H. P. 2013 Experimetal study of a freely falling plate with an inhomogeneous mass distribution. Phys. Rev. E 88, 053008.Google ScholarPubMed
Iversen, J. D. 1979 Autorotating flat-plate wings: the effect of the moment of inertia, geometry and Reynolds number. J. Fluid Mech. 92 (02), 327–348.CrossRefGoogle Scholar
Kern, S., Koumoutsakos, P. & Eschler, K. 2007 Optimization of anguilliform swimming. Phys. Fluids 19 (9), 91102.CrossRefGoogle Scholar
Kowarik, I. & Säumel, I. 2007 Biological flora of Central Europe: Ailanthus altissima (mill.) swingle. Perspect plant ecol. 7, 207–237.CrossRefGoogle Scholar
Kroo, I. 2001 Drag due to lift: concepts for prediction and reduction. Annu. Rev. Fluid Mech. 33, 587–617.CrossRefGoogle Scholar
Lee, S. J., Lee, E. J. & Sohn, M. H. 2014 Mechanism of autorotation flight of maple samaras (Acer palmatum). Exp. Fluids 55, 1718–4.Google Scholar
Lentink, D., Dickson, W. B., van Leeuwen, J. L. & Dickinson, M. H. 2009 Leading-edge vortices elevate lift of leading-edge vortices elevate lift of autorotating plant seeds. Science 324, 1438–1440.CrossRefGoogle ScholarPubMed
Lugt, H. J. 1983 Autorotation. Annu. Rev. Fluid Mech. 15, 123–147.CrossRefGoogle Scholar
Magnus, G. 1853 Ueber die abseichung der geschosse. Poggendorfer Ann. Phys. 88, 604.CrossRefGoogle Scholar
Mahadevan, L., Ryu, W. S. & Samuel, A. D. T. 1999 Tumbling cards. Phys. Fluids 11 (1), 1–3.CrossRefGoogle Scholar
Matlack, G. R. 1987 Diaspore size, shape, and fall behavior in wind-dispersed plant species. Am. J. Bot. 74 (8), 1150–1160.Google Scholar
Maxwell, J. C. 1853 On a particular case of a descent of a heavy body in a residing medium. Camb. Dublin Math. J. 9, 145–148.Google Scholar
McCormick, B. W. 1994 Aerodynamics, Aeronautics and Flight Mechanics, 2nd edn. Wiley.Google Scholar
Munk, M. M. 1925 Note on the air forces on a wing caused by pitching. NACA Tech. Notes 217, 1–6.Google Scholar
Norberg, R. A. 1973 Autorotation, self-stability, and structure of single-winged fruits and seeds (samaras) with comparative remarks on animal flight. Biol. Rev. 48, 561–596.CrossRefGoogle Scholar
Quinn, D. B., Lauder, G. V. & Smits, A. J. 2015 Maximizing the efficiency of a flexible propulsor using experimental optimization. J. Fluid Mech. 767, 430–448.CrossRefGoogle Scholar
Ramananarivo, S., Mitchel, T. & Ristroph, L. 2019 Improving the propulsion speed of a heaving wing through artificial evolution of shape. Proc. R. Soc. Lond. A 475 (2221), 20180375.CrossRefGoogle Scholar
Ruifeng, H. 2015 Three-dimensional flow past rotating wing at low Reynolds number: a computational study. Fluid Dyn. Res. 47, 045503.Google Scholar
Sedov, L. I. 1965 Two-dimensional Problems in Hydrodynamics and Aerodynamics. Wiley.CrossRefGoogle Scholar
Smith, E. H. 1971 Autorotation wings: an experimental investigation. J. Fluid Mech. 50, 513–534.CrossRefGoogle Scholar
Tam, D., Bush, J. W. M., Robitaille, M. & Kudrolli, A. 2010 Tumbling dynamics of passive flexible wings. Phys. Rev. Lett. 104, 184504.CrossRefGoogle ScholarPubMed
Varshney, K., Chang, S. & Wang, Z. J. 2012 The kinematics of falling maple seeds and the initial transition to a helical motion. Nonlinearity 25 (1), C1.CrossRefGoogle Scholar
Varshney, K., Chang, S. & Wang, Z. J. 2013 Unsteady aerodynamic forces and torques on falling parallelograms in coupled tumbling-helical motions. Phys. Rev. E 87, 053021.Google ScholarPubMed
Vogel, S. 1994 Life in Moving Fluids: The Physical Biology of Flow. Princeton University Press.Google Scholar
Wang, W. B., Hu, R. F., Xu, S. J. & Wu, Z. N. 2013 Influence of aspect ratio on tumbling plates. J. Fluid Mech. 733, 650–679.CrossRefGoogle Scholar