Article contents
Shallow, gravity-driven flow in a poro-elastic layer
Published online by Cambridge University Press: 05 August 2015
Abstract
By combining Biot’s theory of poro-elasticity with standard shallow-layer scalings, a theoretical model is developed to describe axisymmetric gravity-driven flow through a shallow deformable porous medium. Motivated in part by observations of surface uplift around  $\text{CO}_{2}$ sequestration sites, the model is used to explore the injection of a dense fluid into a horizontal, deformable porous layer that is initially saturated with another, less dense, fluid. The layer lies between a rigid base and a flexible overburden, both of which are impermeable. As the injected fluid spreads under gravity, the matrix deforms and the overburden lifts up. The coupled model predicts the location of the injected fluid as it spreads and the resulting uplift of the overburden due to deformation of the solid matrix. In general, the uplift spreads diffusively far ahead of the injected fluid. If fluid is injected with a constant flux and the medium is unbounded, both the uplift and the injected fluid spread in a self-similar fashion with the same similarity variable
$\text{CO}_{2}$ sequestration sites, the model is used to explore the injection of a dense fluid into a horizontal, deformable porous layer that is initially saturated with another, less dense, fluid. The layer lies between a rigid base and a flexible overburden, both of which are impermeable. As the injected fluid spreads under gravity, the matrix deforms and the overburden lifts up. The coupled model predicts the location of the injected fluid as it spreads and the resulting uplift of the overburden due to deformation of the solid matrix. In general, the uplift spreads diffusively far ahead of the injected fluid. If fluid is injected with a constant flux and the medium is unbounded, both the uplift and the injected fluid spread in a self-similar fashion with the same similarity variable 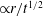 $\propto r/t^{1/2}$. The asymptotic form of this spreading is established. Results from a series of laboratory experiments, using polyacrylamide hydrogel particles to create a soft poro-elastic material, are compared qualitatively with the predictions of the model.
$\propto r/t^{1/2}$. The asymptotic form of this spreading is established. Results from a series of laboratory experiments, using polyacrylamide hydrogel particles to create a soft poro-elastic material, are compared qualitatively with the predictions of the model.
- Type
- Papers
- Information
- Copyright
- © 2015 Cambridge University Press
References
- 15
- Cited by



