Article contents
Self-similar rupture of thin films of power-law fluids on a substrate
Published online by Cambridge University Press: 04 August 2017
Abstract
Thinning and rupture of a thin film of a power-law fluid on a solid substrate under the balance between destabilizing van der Waals pressure and stabilizing capillary pressure is analysed. In a power-law fluid, viscosity is not constant but is proportional to the deformation rate raised to the 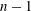 $n-1$ power, where
$n-1$ power, where 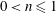 $0<n\leqslant 1$ is the power-law exponent (
$0<n\leqslant 1$ is the power-law exponent (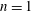 $n=1$ for a Newtonian fluid). In the first part of the paper, use is made of the slenderness of the film and the lubrication approximation is applied to the equations of motion to derive a spatially one-dimensional nonlinear evolution equation for film thickness. The variation with time remaining until rupture of the film thickness, the lateral length scale, fluid velocity and viscosity is determined analytically and confirmed by numerical simulations for both line rupture and point rupture. The self-similarity of the numerically computed film profiles in the vicinity of the location where the film thickness is a minimum is demonstrated by rescaling of the transient profiles with the scales deduced from theory. It is then shown that, in contrast to films of Newtonian fluids undergoing rupture for which inertia is always negligible, inertia can become important during thinning of films of power-law fluids in certain situations. The critical conditions for which inertia becomes important and the lubrication approximation is no longer valid are determined analytically. In the second part of the paper, thinning and rupture of thin films of power-law fluids in situations when inertia is important are simulated by solving numerically the spatially two-dimensional, transient Cauchy momentum and continuity equations. It is shown that as such films continue to thin, a change of scaling occurs from a regime in which van der Waals, capillary and viscous forces are important to one where the dominant balance of forces is between van der Waals, capillary and inertial forces while viscous force is negligible.
$n=1$ for a Newtonian fluid). In the first part of the paper, use is made of the slenderness of the film and the lubrication approximation is applied to the equations of motion to derive a spatially one-dimensional nonlinear evolution equation for film thickness. The variation with time remaining until rupture of the film thickness, the lateral length scale, fluid velocity and viscosity is determined analytically and confirmed by numerical simulations for both line rupture and point rupture. The self-similarity of the numerically computed film profiles in the vicinity of the location where the film thickness is a minimum is demonstrated by rescaling of the transient profiles with the scales deduced from theory. It is then shown that, in contrast to films of Newtonian fluids undergoing rupture for which inertia is always negligible, inertia can become important during thinning of films of power-law fluids in certain situations. The critical conditions for which inertia becomes important and the lubrication approximation is no longer valid are determined analytically. In the second part of the paper, thinning and rupture of thin films of power-law fluids in situations when inertia is important are simulated by solving numerically the spatially two-dimensional, transient Cauchy momentum and continuity equations. It is shown that as such films continue to thin, a change of scaling occurs from a regime in which van der Waals, capillary and viscous forces are important to one where the dominant balance of forces is between van der Waals, capillary and inertial forces while viscous force is negligible.
- Type
- Papers
- Information
- Copyright
- © 2017 Cambridge University Press
References
- 21
- Cited by



