Hostname: page-component-586b7cd67f-gb8f7 Total loading time: 0 Render date: 2024-11-30T19:58:38.924Z Has data issue: false hasContentIssue false
  • English
  • Français

Sediment suspension and bed morphology in a mean shear free turbulent boundary layer

Published online by Cambridge University Press:  29 April 2020

Blair A. Johnson Open the ORCID record for Blair A. Johnson [Opens in a new window]  and
Edwin A. Cowen Open the ORCID record for Edwin A. Cowen [Opens in a new window]
Blair A. Johnson*
Affiliation:
Department of Civil, Architectural and Environmental Engineering, The University of Texas at Austin, Austin, TX78712, USA
Edwin A. Cowen
Affiliation:
DeFrees Hydraulics Laboratory, School of Civil and Environmental Engineering, Cornell University, Ithaca, NY14853, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

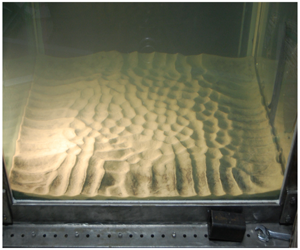
We experimentally characterize turbulence in boundary layers generated by different levels of nearly isotropic homogeneous turbulence over flat impervious boundaries and over non-cohesive sediment beds with and without ripples. We use randomly actuated synthetic jet arrays (RASJA – Variano & Cowen, J. Fluid Mech., vol. 604, 2008, pp. 1–32) to generate high Reynolds number ($Re_{\unicode[STIX]{x1D706}}\sim 300$) turbulence with negligible secondary mean flows or mean bed shear. The isotropic region and the boundary layer connecting this isotropic region to the bed are investigated using particle image velocimetry measurements. Surprisingly, we observe the development of ripples on the sediment bed ($D_{50}=260~\unicode[STIX]{x03BC}\text{m}$). We draw comparisons between the mean shear free turbulent boundary layer formed above a flat stationary solid boundary (Johnson & Cowen, J. Fluid Mech., vol. 835, 2018, pp. 217–251) and its sediment counterpart by considering statistical metrics including root mean square velocity fluctuations, turbulent kinetic energy, dissipation rates, production, integral scales, Reynolds stresses and spatial spectra. Using an 8 × 8 RASJA, we find the damping of turbulence and dissipation rates at flat and rippled sediment beds with low levels of suspended sediments relative to an impermeable glass bed, whereas with a 16 × 16 RASJA we find the enhancement of turbulence and dissipation rates of a resuspending sediment bed relative to an impermeable glass bed. We hypothesize that this may be a result of a change in direction of the bed-normal mean flows at the porous boundary. We explore a relationship between the integral length scale of the turbulence with the resulting sediment ripple spacing by varying the mean on-time of the RASJA algorithm.

JFM classification

Geophysical and Geological Flows: Sediment transport Turbulent Flows: Turbulent boundary layers Turbulent Flows: Isotropic turbulence
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 894 , 10 July 2020 , A8
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Andersen, P. S., Kays, W. M. & Moffat, R. J.1972 The turbulent boundary layer on a porous plate: an experimental sudy of the fluid mechanics for adverse free stream pressure gradients. NASA Tech. Rep. 127817.Google Scholar
Brumley, B. H. & Jirka, G. H. 1987 Near-surface turbulence in a grid-stirred tank. J. Fluid Mech. 183, 235–263.CrossRefGoogle Scholar
Buffington, J. M. 1999 The legend of A. F. Shields. ASCE J. Hydraul. Engng 125 (4), 376–387.CrossRefGoogle Scholar
Butt, T. & Russell, P. 1999 Suspended sediment transport mechanisms in high-energy swash. Mar. Geol. 161, 361–375.Google Scholar
Calmet, I. & Magnaudet, J. 2003 Statistical structure of high-Reynolds-number turbulence close to the free surface of an open-channel flow. J. Fluid Mech. 474, 355–378.CrossRefGoogle Scholar
Cowen, E. A. & Monismith, S. G. 1997 A hybrid digital particle tracking velocimetry technique. Exp. Fluids 22, 199–211.CrossRefGoogle Scholar
Cowen, E. A., Sou, I. M., Liu, P. L. & Raubenheimer, B. 2003 Particle image velocimetry measurements within a laboratory-generated swash zone. ASCE J. Engng Mech. 129 (10), 1119–1129.CrossRefGoogle Scholar
De Angelis, V., Lombardi, P. & Banerjee, S. 1997 Direct numerical simulation of turbulent flow over a wavy wall. Phys. Fluids 9, 2429–2442.CrossRefGoogle Scholar
Diplas, P., Dancey, C. L., Celik, A. O., Valyrakis, M., Greer, K. & Akar, T. 2008 The role of impulse on the initiation of particle movement under turbulent flow conditions. Science 322 (5902), 717–720.CrossRefGoogle ScholarPubMed
Doron, P., Bertuccioli, L., Katz, J. & Osborn, T. R. 2000 Turbulence characteristics and dissipation estimates in the coastal ocean bottom boundary layer from PIV data. J. Phys. Oceanogr. 31, 2108–2134.2.0.CO;2>CrossRefGoogle Scholar
Dutton, T. A.1958 The effects of distributed suction on the development of turbulent boundary layers. Tech. Rep. 3155. Aeronautical Research Council RM.Google Scholar
Efron, B. & Tibshirani, R. 1993 An Introduction to the Bootstrap. Chapman and Hall.CrossRefGoogle Scholar
Einstein, H. A.1950 The bed-load function for sediment transportation in open channel flows. Tech. Rep. 1026. United States Department of Agriculture.Google Scholar
Favre, A., Dumas, R. & Verollet, E.1961 Couche limite sur paroi plane poreuse avec aspiration. Tech. Rep. Comm. En. Atom. Rapp.Google Scholar
Ferro, M.2017 Experimental study on turbulent boundary-layer flows with wall transpiration. PhD thesis, Royal Institute of Technology, Stockholm, Sweden.Google Scholar
Finn, J. R., Li, M. & Apte, S. V. 2016 Particle based modelling and simulation of natural sand dynamics in the wave bottom boundary layer. J. Fluid Mech. 796, 340–385.CrossRefGoogle Scholar
Foster, D. L., Bowen, A. J., Holman, R. A. & Natoo, P. 2006 Field evidence of pressure gradient induced incipient motion. J. Geophys. Res. 111, doi:10.1029/2004JC002863.CrossRefGoogle Scholar
Frank, D., D., F., Sou, I. M. & Calatoni, J. 2015 Incipient motion of surf zone sediments. J. Geophys. Res. 120, 5710–5734.CrossRefGoogle Scholar
Heathershaw, A. D. & Thorne, P. D. 1985 Sea-bed noises reveal role of turbulent bursting phenomenon in sediment transport by tidal currents. Nature 316 (25), 339–342.CrossRefGoogle Scholar
Hoffmans, G. J. C. M. 2010 Stability of stones under uniform flow. ASCE J. Hydraul. Engng 136 (2), 129–136.CrossRefGoogle Scholar
Hopfinger, E. J. & Toly, J.-A. 1976 Spatially decaying turbulence and its relation to mixing across density interfaces. J. Fluid Mech. 78 (1), 155–175.CrossRefGoogle Scholar
Hunt, J. C. R. & Graham, J. M. R. 1978 Free-stream turbulence near plane boundaries. J. Fluid Mech. 84, 209–235.CrossRefGoogle Scholar
Johnson, B. A.2012 Turbulent boundary layers and sediment resuspension in the absence of mean shear. Master’s thesis, Cornell University.Google Scholar
Johnson, B. A.2016 Turbulent boundary layers and sediment suspension absent mean flow-induced shear. PhD thesis, Cornell University.CrossRefGoogle Scholar
Johnson, B. A. & Cowen, E. A. 2018 Turbulent boundary layers absent mean shear. J. Fluid Mech. 835, 217–251.CrossRefGoogle Scholar
Kennedy, J. F. 1963 The mechanics of dunes and antidunes in erodible-bed channels. J. Fluid Mech. 16 (4), 521–546.CrossRefGoogle Scholar
King, A. T., Tinoco, R. O. & Cowen, E. A. 2012 A k-𝜖 turbulence model based on the scales of vertical shear and stem wakes valid for emergent and submerged vegetated flows. J. Fluid Mech. 701, 1–39.CrossRefGoogle Scholar
Kornilov, V. I. 2015 Current state and prospects of researches on the control of turbulent boundary layer by air blowing. Prog. Aerosp. Sci. 76, 1–23.CrossRefGoogle Scholar
Kramer, H. 1935 Sand mixtures and sand movement in fluvial models. Trans. Am. Soc. Civil Engrs 100, 798–838.Google Scholar
Lancaster, N. 1989 Star dunes. Prog. Phys. Geog. 13, 67–91.CrossRefGoogle Scholar
Leary, K. C. P. & Schmeeckle, M. W. 2017 The importance of splat events to the spatiotemporal structure of near-bed fluid velocity and bed load motion over bed forms: laboratory experiments downstream of a backward facing step. J. Geophys. Res. 122 (12), 2411–2430.CrossRefGoogle Scholar
Lelouvetel, J., Bigillon, F., Doppler, D., Vinkovic, I. & Champagne, J.-Y. 2009 Experimental investigation of ejections and sweeps involved in particle suspension. Water Resour. Res. 45, doi:10.1029/2007WR006520.CrossRefGoogle Scholar
Liao, Q. & Cowen, E. A. 2005 An efficient anti-aliasing spectral continuous window shifting technique for PIV. Exp. Fluids 38, 197–208.CrossRefGoogle Scholar
van Maanen, B., Coco, G., Bryan, K. R. & Ruessink, B. G. 2010 The use of artificial neural networks to analyze and predict alongshore sediment transport. Nonlinear Process. Geophys. 17, 395–404.CrossRefGoogle Scholar
Masselink, G. & Hughes, M. 1998 Field investigation of sediment transport in the swash zone. Cont. Shelf Res. 18, 1179–1199.CrossRefGoogle Scholar
McCorquodale, M. W. & Munro, R. J. 2017 Experimental study of oscillating-grid turbulence interacting with a solid boundary. J. Fluid Mech. 813, 768–798.CrossRefGoogle Scholar
McCorquodale, M. W. & Munro, R. J. 2018 Analysis of intercomponent energy transfer in the interaction of oscillating-grid turbulence with an impermeable boundary. Phys. Fluids 30, 015105.CrossRefGoogle Scholar
McDougall, T. 1979 Measurements of turbulence in a zero-mean-shear mixed layer. J. Fluid Mech. 94 (3), 409–431.CrossRefGoogle Scholar
McKenna, S. P. & McGillis, W. R. 2004 Observations of flow repeatability and secondary circulation in an oscillating grid-stirred tank. Phys. Fluids 16 (9), 3499–3502.CrossRefGoogle Scholar
Medina, P., Sanchez, M. A. & Redondo, J. M. 2001 Grid stirred turbulence: applications to the initiation of sediment motion and lift-off studies. Phys. Chem. Earth B 26 (4), 299–304.CrossRefGoogle Scholar
Mickley, H. S. & Davis, R. S.1957 Momentum transfer for flow over a flat plate with blowing. NASA Tech. Rep. 2017.Google Scholar
Musa, R. A., Takarrouht, S., Louge, M. Y., Xu, J. & Berberich, M. E. 2014 Pore pressure in a wind-swept rippled bed below the suspension threshold. J. Geophys. Res. 119, 2574–2590.CrossRefGoogle Scholar
Nelson, J. M., Shreve, R. L., McLean, S. R. & Drake, T. G. 1995 Role of near-bed turbulence structure in bed load transport and bed form mechanics. Water Resour. Res. 31 (8), 2071–2086.CrossRefGoogle Scholar
Paintal, A. S. 1971 Concept of critical shear stress in loose boundary open channels. J. Hydraul Res. 9 (1), 91–113.CrossRefGoogle Scholar
Pao, Y.-H. 1965 Structure of turbulent velocity and scalar fields at large wavenumbers. Phys. Fluids 8 (6), 1063–1075.CrossRefGoogle Scholar
Perez-Alvarado, A., Mydlarski, L. & Gaskin, S. 2016 Effect of the driving algorithm on the turbulence generated by a random jet array. Exp. Fluids 57 (2), 20.CrossRefGoogle Scholar
Perot, B. & Moin, P. 1995a Shear-free turbulent boundary layers. Part 1. Physical insights into near-wall turbulence. J. Fluid Mech. 295, 199–227.CrossRefGoogle Scholar
Petti, M. & Longo, S. 2001 Turbulence experiments in the swash zone. Coast. Engng Japan 43, 1–24.CrossRefGoogle Scholar
Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.CrossRefGoogle Scholar
Puleo, J. A., Beach, R. A., Holman, R. A. & Allen, J. S. 2000 Swash zone sediment suspension and transport and the importance of bore-generated turbulence. J. Geophys. Res. 105 (C7), 17021–17044.CrossRefGoogle Scholar
Redondo, J. M., Durrieu de Madron, X., Medina, P., Sanchez, M. A. & Schaaff, E.2001 Comparison of sediment resuspension measurements in sheared and zero-mean turbulent flows. Cont. Shelf Res 21 (18–19), 2095–2103.CrossRefGoogle Scholar
van Rijn, L. C. 1984 Sediment transport. Part I. Bed load transport. ASCE J. Hydraul. Engng 110, 1431–1456.CrossRefGoogle Scholar
Rodriguez-Abudo, S., Foster, D. L. & Henriquez, M. 2013 Spatial variability of the wave bottom boundary layer over movable rippled beds. J. Geophys. Res. 118, 3490–3506.CrossRefGoogle Scholar
Rouse, H. & Dodu, J. 1955 Diffusion turbulente a travers une discontinuite de densite. La Houille Blanche 10, 522–532.CrossRefGoogle Scholar
Sanchez, M. A. & Redondo, J. M. 1998 Observations from grid stirred turbulence. Appl. Sci. Res. 59, 243–254.CrossRefGoogle Scholar
Schmeeckle, M. W. 2015 The role of velocity, pressure, and bed stress fluctuations in bed load transport over bed forms: numerical simulation downstream of a backward-facing step. Earth Surf. Dyn. Discuss. 2 (2), 715–732.CrossRefGoogle Scholar
Shields, A. F.1936 Anwendung der anhlichkeitsmechanik und turbulenzforschung auf die geschiebebewegung. PhD thesis, Technical University Berlin.Google Scholar
Sleath, J. F. A. 1999 Conditions for plug formation in oscillatory flow. Cont. Shelf Res. 19, 1643–1664.CrossRefGoogle Scholar
Sinclair, P. C. 1968 General characteristics of dust devils. J. Appl. Meteorol. 8, 32–45.2.0.CO;2>CrossRefGoogle Scholar
Spalart, P. R. 1988 Direct simulation of a turbulent boundary layer up to R = 1410. J. Fluid Mech. 187, 61–98.CrossRefGoogle Scholar
Stoesser, T., Braun, C., Garcia-Villalba, M. & Rodi, W. 2008 Turbulence structures in flow over two-dimensional dunes. ASCE J. Hydraul. Engng 134 (1), 42–55.CrossRefGoogle Scholar
Teixeira, M. A. C. & Belcher, S. E. 2000 Dissipation of shear-free turbulence near boundaries. J. Fluid Mech. 422, 167–191.CrossRefGoogle Scholar
Tennekes, H. 1965 Similarity laws for turbulent boundary layers with suction of injection. J. Fluid Mech. 21 (04), 689–703.CrossRefGoogle Scholar
Thomas, N. H. & Hancock, P. E. 1977 Grid turbulence near a moving wall. J. Fluid Mech. 82, 481–496.CrossRefGoogle Scholar
Thompson, S. M. & Turner, J. S. 1975 Mixing across an interface due to turbulence generated by an oscillating grid. J. Fluid Mech. 67 (2), 349–368.CrossRefGoogle Scholar
Traykovski, P., Hay, A. E., Irish, J. D. & Lynch, J. F. 1999 Geometry, migration, and evolution of wave orbital ripples at leo-15. J. Geophys. Res. 104 (C1), 1505–1524.CrossRefGoogle Scholar
Tsai, C. H. & Lick, W. 1986 A portable device for measuring sediment resuspension. J. Great Lakes Res. 12 (4), 314–321.CrossRefGoogle Scholar
Turner, J. S. 1968 The influence of molecular diffusivity on turbulent entrainment across a density interface. J. Fluid Mech. 33, 639–656.CrossRefGoogle Scholar
Uzkan, T. & Reynolds, W. C. 1967 A shear-free turbulent boundary layer. J. Fluid Mech. 28, 803–821.CrossRefGoogle Scholar
Variano, E. A., Bodenschatz, E. & Cowen, E. A. 2004 A random synthetic jet array driven turbulence tank. Exp. Fluids 37, 613–615.CrossRefGoogle Scholar
Variano, E. A. & Cowen, E. A. 2008 A random-jet-stirred turbulence tank. J. Fluid Mech. 604, 1–32.CrossRefGoogle Scholar
Voermans, J. J., Ghisalberti, M. & Ivey, G. N. 2018 The hydrodynamic response of the sediment-water interface to coherent turbulent motions. Geophys. Res. Lett. 45 (19), 10520–10527.CrossRefGoogle Scholar
van der Werf, J. J., Doucette, J. S., O’Donoghue, T. O. & Ribberink, J. S. 2007 Detailed measurements of velocities and suspended sand concentrations over full-scale ripples in regular oscillatory flows. J. Geophys. Res. 112, FO2012.CrossRefGoogle Scholar
Westerweel, J. 1994 Efficient detection of spurious vectors in particle image velocimetry data. Exp. Fluids 16, 236–247.CrossRefGoogle Scholar
Yager, E. M., Schmeeckle, M. W. & Badoux, A. 2018 Resistance is not futile: grain resistance controls on observed critical shields stress variations. J. Geophys. Res. 123 (12), 3308–3322.Google Scholar
Zhang, D., Narteau, C., Rozier, O. & Courrech du Pont, S. 2012 Morphology and dynamics of star dunes from numerical modelling. Nature Geosci. Lett. 5, 463–467.CrossRefGoogle Scholar