Article contents
Secondary instabilities in Taylor–Couette flow of shear-thinning fluids
Published online by Cambridge University Press: 17 December 2021
Abstract
The stability of the Taylor vortex flow in Newtonian and shear-thinning fluids is investigated in the case of a wide gap Taylor–Couette system. The considered radius ratio is  $\eta = R_1/R_2=0.4$. The aspect ratio (length over the gap width) of experimental configuration is 32. Flow visualization and measurements of two-dimensional flow fields with particle image velocimetry are performed in a glycerol aqueous solution (Newtonian fluid) and in xanthan gum aqueous solutions (shear-thinning fluids). The experiments are accompanied by axisymmetric numerical simulations of Taylor–Couette flow in the same gap of a Newtonian and a purely viscous shear-thinning fluid described by the Carreau model. The experimentally observed critical Reynolds and wavenumbers at the onset of Taylor vortices are in very good agreement with that obtained from a linear theory assuming a purely viscous shear-thinning fluid and infinitely long cylinders. They are not affected by the viscoelasticity of the used fluids. For the Newtonian fluid, the Taylor vortex flow (TVF) regime is found to bifurcate into a wavy vortex flow with a high frequency and low amplitude of axial oscillations of the vortices at
$\eta = R_1/R_2=0.4$. The aspect ratio (length over the gap width) of experimental configuration is 32. Flow visualization and measurements of two-dimensional flow fields with particle image velocimetry are performed in a glycerol aqueous solution (Newtonian fluid) and in xanthan gum aqueous solutions (shear-thinning fluids). The experiments are accompanied by axisymmetric numerical simulations of Taylor–Couette flow in the same gap of a Newtonian and a purely viscous shear-thinning fluid described by the Carreau model. The experimentally observed critical Reynolds and wavenumbers at the onset of Taylor vortices are in very good agreement with that obtained from a linear theory assuming a purely viscous shear-thinning fluid and infinitely long cylinders. They are not affected by the viscoelasticity of the used fluids. For the Newtonian fluid, the Taylor vortex flow (TVF) regime is found to bifurcate into a wavy vortex flow with a high frequency and low amplitude of axial oscillations of the vortices at  ${Re} = 5.28 \, {Re}_c$. At
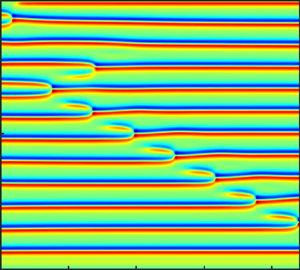
${Re} = 5.28 \, {Re}_c$. At  ${Re} = 6.9 \, {Re}_c$, the frequency of oscillations decreases and the amplitude increases abruptly. For the shear-thinning fluids the secondary instability conserves axisymmetry. The latter is characterized by an instability of the array of vortices leading to a continuous sequence of creation and merging of vortex pairs. Axisymmetric numerical simulations reproduce qualitatively very well the experimentally observed flow behaviour.
${Re} = 6.9 \, {Re}_c$, the frequency of oscillations decreases and the amplitude increases abruptly. For the shear-thinning fluids the secondary instability conserves axisymmetry. The latter is characterized by an instability of the array of vortices leading to a continuous sequence of creation and merging of vortex pairs. Axisymmetric numerical simulations reproduce qualitatively very well the experimentally observed flow behaviour.
JFM classification
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press
References
REFERENCES
- 2
- Cited by



