Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Yong, Wen-An
and
Luo, Li-Shi
2012.
Accuracy of the viscous stress in the lattice Boltzmann equation with simple boundary conditions.
Physical Review E,
Vol. 86,
Issue. 6,
Braz e Silva, Pablo
2012.
The resolvent technique for investigating the stability of plane Couette flow: Bounds for the pressure terms.
Nonlinear Analysis: Real World Applications,
Vol. 13,
Issue. 6,
p.
2680.
Huang, Haibo
Wu, YanFeng
and
Lu, Xiyun
2012.
Shear viscosity of dilute suspensions of ellipsoidal particles with a lattice Boltzmann method.
Physical Review E,
Vol. 86,
Issue. 4,
Wang, Z.
Sui, Y.
Spelt, P. D. M.
and
Wang, W.
2013.
Three-dimensional dynamics of oblate and prolate capsules in shear flow.
Physical Review E,
Vol. 88,
Issue. 5,
Chen, Yu
Cai, Qingdong
Xia, Zhenhua
Wang, Moran
and
Chen, Shiyi
2013.
Momentum-exchange method in lattice Boltzmann simulations of particle-fluid interactions.
Physical Review E,
Vol. 88,
Issue. 1,
Wen, Bing-Hai
Chen, Yan-Yan
Zhang, Ren-Liang
Zhang, Chao-Ying
and
Fang, Hai-Ping
2013.
Lateral Migration and Nonuniform Rotation of Biconcave Particle Suspended in Poiseuille Flow.
Chinese Physics Letters,
Vol. 30,
Issue. 6,
p.
064701.
Nilsen, Christopher
and
Andersson, Helge I.
2013.
Chaotic rotation of inertial spheroids in oscillating shear flow.
Physics of Fluids,
Vol. 25,
Issue. 1,
Huang, Haibo
Yang, Xin
and
Lu, Xi-yun
2014.
Sedimentation of an ellipsoidal particle in narrow tubes.
Physics of Fluids,
Vol. 26,
Issue. 5,
Mao, Wenbin
and
Alexeev, Alexander
2014.
Motion of spheroid particles in shear flow with inertia.
Journal of Fluid Mechanics,
Vol. 749,
Issue. ,
p.
145.
Yu, Chunlong
Huang, Haibo
and
Lu, Xiyun
2014.
Lattice Boltzmann Study of a Vortex Ring Impacting Spheroidal Particles.
Advances in Applied Mathematics and Mechanics,
Vol. 6,
Issue. 4,
p.
461.
Rosén, T.
Lundell, F.
and
Aidun, C. K.
2014.
Effect of fluid inertia on the dynamics and scaling of neutrally buoyant particles in shear flow.
Journal of Fluid Mechanics,
Vol. 738,
Issue. ,
p.
563.
Wen, Binghai
Zhang, Chaoying
Tu, Yusong
Wang, Chunlei
and
Fang, Haiping
2014.
Galilean invariant fluid–solid interfacial dynamics in lattice Boltzmann simulations.
Journal of Computational Physics,
Vol. 266,
Issue. ,
p.
161.
Nie, D.M.
and
Lin, J.Z.
2015.
Behavior of three circular particles in a confined power-law fluid under shear.
Journal of Non-Newtonian Fluid Mechanics,
Vol. 221,
Issue. ,
p.
76.
Rosén, T.
Do-Quang, M.
Aidun, C. K.
and
Lundell, F.
2015.
The dynamical states of a prolate spheroidal particle suspended in shear flow as a consequence of particle and fluid inertia.
Journal of Fluid Mechanics,
Vol. 771,
Issue. ,
p.
115.
Yang, Xin
Huang, Haibo
and
Lu, Xiyun
2015.
Sedimentation of an oblate ellipsoid in narrow tubes.
Physical Review E,
Vol. 92,
Issue. 6,
Rosén, T.
Einarsson, J.
Nordmark, A.
Aidun, C. K.
Lundell, F.
and
Mehlig, B.
2015.
Numerical analysis of the angular motion of a neutrally buoyant spheroid in shear flow at small Reynolds numbers.
Physical Review E,
Vol. 92,
Issue. 6,
Einarsson, J.
Candelier, F.
Lundell, F.
Angilella, J. R.
and
Mehlig, B.
2015.
Rotation of a spheroid in a simple shear at small Reynolds number.
Physics of Fluids,
Vol. 27,
Issue. 6,
Rosén, T.
Do-Quang, M.
Aidun, C. K.
and
Lundell, F.
2015.
Effect of fluid and particle inertia on the rotation of an oblate spheroidal particle suspended in linear shear flow.
Physical Review E,
Vol. 91,
Issue. 5,
Widhalm, Markus
2015.
Lagrangian Trajectory Simulation of Rotating Regular Shaped Ice Particles.
Vol. 1,
Issue. ,
Challabotla, Niranjan Reddy
Nilsen, Christopher
and
Andersson, Helge I.
2015.
On rotational dynamics of inertial disks in creeping shear flow.
Physics Letters A,
Vol. 379,
Issue. 3,
p.
157.
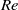 ) exceeding 305. The simulations cover the regime up to
) exceeding 305. The simulations cover the regime up to 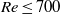 . The rotational behaviour of the spheroid appears to be not only sensitive to the Reynolds number but also to its initial orientation. We discuss the effects of initial orientation in detail. For
. The rotational behaviour of the spheroid appears to be not only sensitive to the Reynolds number but also to its initial orientation. We discuss the effects of initial orientation in detail. For 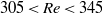 we find that the prolate spheroid reaches a periodic mode characterized by precession and nutation around an inclined axis which is located close to the middle plane where the velocity is zero. For
we find that the prolate spheroid reaches a periodic mode characterized by precession and nutation around an inclined axis which is located close to the middle plane where the velocity is zero. For  , the prolate spheroid precesses around the vorticity direction with a nutation. For
, the prolate spheroid precesses around the vorticity direction with a nutation. For  close to the critical
close to the critical  , a period-doubling phenomenon is observed. We also identify a motionless mode at higher Reynolds numbers (
, a period-doubling phenomenon is observed. We also identify a motionless mode at higher Reynolds numbers (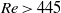 ) for the prolate spheroid. For the oblate spheroid the dynamic equilibrium modes found are log rolling, inclined rolling and different steady states for
) for the prolate spheroid. For the oblate spheroid the dynamic equilibrium modes found are log rolling, inclined rolling and different steady states for 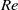 increasing from 0 to 520. The initial-orientation effects are studied by simulations of 57 evenly distributed initial orientations for each
increasing from 0 to 520. The initial-orientation effects are studied by simulations of 57 evenly distributed initial orientations for each 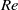 investigated. Only one mode is found for the prolate spheroid for
investigated. Only one mode is found for the prolate spheroid for 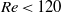 and
and 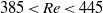 . In other
. In other  regimes, more than one mode is possible and the final mode is sensitive to the initial orientation. However, the oblate spheroid dynamics are insensitive to its initial orientation.
regimes, more than one mode is possible and the final mode is sensitive to the initial orientation. However, the oblate spheroid dynamics are insensitive to its initial orientation.