Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-22T09:09:14.933Z Has data issue: false hasContentIssue false
  • English
  • Français

The rise heights of low- and high-Froude-number turbulent axisymmetric fountains

Published online by Cambridge University Press:  12 December 2011

H. C. Burridge  and
G. R. Hunt
H. C. Burridge
Affiliation:
Department of Civil and Environmental Engineering, Imperial College London, Imperial College Road, London SW7 2AZ, UK
G. R. Hunt*
Affiliation:
Department of Civil and Environmental Engineering, Imperial College London, Imperial College Road, London SW7 2AZ, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

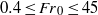We present the results of an experimental investigation across a broad range of source Froude numbers, , into the dynamics, morphology and rise heights of Boussinesq turbulent axisymmetric fountains in quiescent uniform environments. Typically, these fountains are thought to rise to an initial height, , before settling back and fluctuating about a lesser (quasi-) steady height, 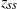. Our measurements show that this is not always the case and the ratio of the fountain’s initial rise height to steady rise height, 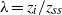, varies widely, 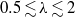, across the range of 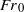investigated. As a result of near-ideal start-up conditions provided by the experimental set-up we were consistently able to form a vortex at the fountain’s front. This enabled new insights into two features of the initial rise of turbulent fountains. Firstly, for the initial rise height is less than the steady rise height. Secondly, for 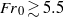, the vortex formed at the fountain’s front pinches off, separates from the main body and rises high above the fountain; there is thus a third rise height to consider, namely, the maximum vortex rise height, 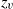. From our observations we propose classifying turbulent axisymmetric fountains into five regimes (as opposed to the current three regimes) and present detailed descriptions of the flow in each. Finally, based on an analysis of the rise height fluctuations and the width of fountains in (quasi-) steady state we provide further insight into the physical cause of height fluctuations.

JFM classification

Convection: Plumes/thermals Turbulent Flows: Turbulent convection
Type
Papers
Information
Journal of Fluid Mechanics , Volume 691 , 25 January 2012 , pp. 392 - 416
Copyright
Copyright © Cambridge University Press 2011

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Ansong, J. K., Kyba, P. J. & Sutherland, B. R. 2008 Fountains impinging on a density interface. J. Fluid Mech. 595, 115–139.CrossRefGoogle Scholar
2. Baines, W. D., Turner, J. S. & Campbell, I. H. 1990 Turbulent fountains in an open chamber. J. Fluid Mech. 212, 557–592.Google Scholar
3. Bloomfield, L. J. & Kerr, R. C. 1998 Turbulent fountains in a stratified fluid. J. Fluid Mech. 358, 335–356.Google Scholar
4. Bloomfield, L. J. & Kerr, R. C. 2000 A theoretical model of a turbulent fountain. J. Fluid Mech. 424, 197–216.Google Scholar
5. Campbell, I. H. & Turner, J. S. 1989 Fountains in magma chambers. J. Petrol. 30, 885–923.CrossRefGoogle Scholar
6. Carazzo, G., Kaminski, E. & Tait, S. 2010 The rise and fall of turbulent fountains: a new model for improved quantitative predictions. J. Fluid Mech. 657, 265–284.Google Scholar
7. Devenish, B. J., Rooney, G. G. & Thompson, D. J. 2010 Large-eddy simulation of a buoyant plume in uniform and stably stratified environments. J. Fluid Mech. 652, 75–103.Google Scholar
8. Fischer, H. B., List, E. J., Koh, R. C. Y., Imberger, J. & Brooks, N. H. 1979 Mixing in Inland and Coastal Waters. Academic.Google Scholar
9. Friedman, P. D. 2006 Oscillation in height of a negatively buoyant jet. Trans. ASME: J. Fluids Engng 128, 880–882.Google Scholar
10. Friedman, P. D. & Katz, J. 1999 The flow and mixing mechanisms caused by the impingement of an immiscible interface with a vertical jet. Phys. Fluids 11, 2598.CrossRefGoogle Scholar
11. Gharib, M., Rambod, E. & Shariff, K. 1998 A universal time scale for vortex ring formation. J. Fluid Mech. 360, 121–140.Google Scholar
12. Kaye, N. B. & Hunt, G. R. 2006 Weak fountains. J. Fluid Mech. 558, 319–328.Google Scholar
13. Lin, W. & Armfield, S. W. 2000a Direct simulation of weak axisymmetric fountains in a homogeneous fluid. J. Fluid Mech. 403, 67–88.Google Scholar
14. Lin, W. & Armfield, S. W. 2000b Very weak fountains in a homogeneous fluid. Numer. Heat Transfer A 38, 377–396.Google Scholar
15. Liu, Q. A. & Linden, P. F. 2006 The fluid dynamics of an underfloor air distribution system. J. Fluid Mech. 554, 323–341.Google Scholar
16. Marugán-Cruz, C., Rodríguez-Rodríguez, J. & Martínez-Bazán, C. 2009 Negatively buoyant starting jets. Phys. Fluids 21, 1–14.Google Scholar
17. McDougall, T. J. 1981 Negatively buoyant vertical jets. Tellus 33, 313–320.Google Scholar
18. Morton, B. R., Taylor, G. I. & Turner, J. S. 1956 Turbulent gravitational convection from maintained and instantaneous sources. Proc. R. Soc. Lond. A 234, 1–23.Google Scholar
19. Tennekes, H. & Lumley, J. L. 1972 A First Course in Turbulence. MIT.Google Scholar
20. Turner, J. S. 1966 Jets and plumes with negative or reversing buoyancy. J. Fluid Mech. 26, 779–792.Google Scholar
21. Wang, R.-Q. W., Law, A. W.-K. & Adams, E. E. 2011 Pinch-off and formation number of negatively buoyant jets. Phys. Fluids 23, 52101.Google Scholar
22. Washburn, E. W.  (Ed.) 2003 International Critical Tables of Numerical Data, Physics, Chemistry and Technology. Knovel.Google Scholar
23. White, F. J. 2003 Fluid Mechanics. McGraw-Hill.Google Scholar
24. Williamson, N., Armfield, S. W. & Lin, W. 2010 Transition behaviour of weak turbulent fountains. J. Fluid Mech. 655, 306–326.CrossRefGoogle Scholar
25. Williamson, N., Armfield, S. W. & Lin, W. 2011 Forced turbulent fountain flow behaviour. J. Fluid Mech. 671, 535–558.CrossRefGoogle Scholar
26. Williamson, N., Srinarayana, N., Armfield, S. W., McBain, G. D. & Lin, W. 2008 Low-Reynolds-number fountain behaviour. J. Fluid Mech. 608, 297–317.Google Scholar
27. Zhang, H. & Baddour, R. E. 1998 Maximum penetration of vertical round dense jets at small and large Froude numbers. J. Hydraul. Engng 124, 550–553.Google Scholar