Hostname: page-component-5cf477f64f-qls9x Total loading time: 0 Render date: 2025-04-06T13:02:55.593Z Has data issue: false hasContentIssue false
  • English
  • Français

Reynolds-number measurements for low-Prandtl-number turbulent convection of large-aspect-ratio samples

Published online by Cambridge University Press:  22 May 2013

James Hogg  and
Guenter Ahlers
James Hogg
Affiliation:
Department of Physics and ITST, University of California, Santa Barbara, CA 93106, USA
Guenter Ahlers*
Affiliation:
Department of Physics and ITST, University of California, Santa Barbara, CA 93106, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We present experimental results for the Reynolds number 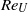${\mathit{Re}}_{U} $ based on the horizontal mean-flow velocity 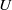$U$ and for 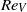${\mathit{Re}}_{V} $ based on the root-mean-square horizontal fluctuation velocity 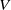$V$ for turbulent Rayleigh–Bénard convection in a cylindrical sample of aspect ratio $\Gamma = 10. 9$ over the Prandtl number range 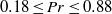$0. 18\leq \mathit{Pr}\leq 0. 88$. The results were derived from space–time cross-correlation functions of shadowgraph images, using the elliptic approximation of He & Zhang (Phys. Rev. E, vol. 73, 2006, 055303). The data cover the Rayleigh number range from 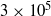$3\times 1{0}^{5} $ to 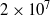$2\times 1{0}^{7} $. We find that 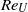${\mathit{Re}}_{U} $ is nearly two orders of magnitude smaller than the values given by the Grossmann–Lohse (GL) model (Grossmann & Lohse, Phys. Rev. E, vol. 66, 2002, 016305) for 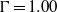$\Gamma = 1. 00$ and attribute this difference to averaging caused by lateral random diffusion of the large-scale circulation cells in large-$\Gamma $ samples. For the fluctuations we found 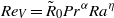${\mathit{Re}}_{V} = {\tilde {R} }_{0} {\mathit{Pr}}^{\alpha } {\mathit{Ra}}^{\eta } $, with 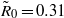${\tilde {R} }_{0} = 0. 31$, 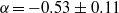$\alpha = - 0. 53\pm 0. 11$ and 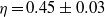$\eta = 0. 45\pm 0. 03$. That result agrees well with the GL model. The close agreement of the coefficient 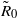${\tilde {R} }_{0} $ must be regarded as a coincidence because the GL model was for 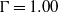$\Gamma = 1. 00$ and for a mean-flow velocity 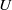$U$.

JFM classification

Convection: Convection Turbulent Flows: Turbulent Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 725 , 25 June 2013 , pp. 664 - 680
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahlers, G. 2009 Turbulent convection. Physics 2, 74–1–7.CrossRefGoogle Scholar
Ahlers, G., Grossmann, S. & Lohse, D. 2009 Heat transfer and large-scale dynamics in turbulent Rayleigh–Bénard convection. Rev. Mod. Phys. 81, 503–538.Google Scholar
Ahlers, G. & Xu, X. 2001 Prandtl-number dependence of heat transport in turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 86, 3320–3323.Google Scholar
Bailon-Cuba, J., Emran, M. S. & Schumacher, J. 2010 Aspect ratio dependence of heat transfer and large-scale flow in turbulent convection. J. Fluid Mech. 655, 152–173.CrossRefGoogle Scholar
Bodenschatz, E., Pesch, W. & Ahlers, G. 2000 Recent developments in Rayleigh–Bénard convection. Annu. Rev. Fluid Mech. 32, 709–778.Google Scholar
Bosbach, J., Weiss, S. & Ahlers, G. 2012 Plume fragmentation by bulk interactions in turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 108, 054501.Google Scholar
Boushehri, A., Bzowski, J., Kestin, J. & Mason, E. A. 1987 Equilibrium and transport properties of eleven polyatomic gases at low density. J. Phys. Chem. Ref. Data 16 (3), 445–466.CrossRefGoogle Scholar
Braker, W. & Mossman, A. L. 1974 The Matheson Unabridged Gas Data Book: A Compilation of Physical and Thermodynamic Properties of Gases. Matheson Gas Products.Google Scholar
Brown, E. & Ahlers, G. 2008 A model of diffusion in a potential well for the dynamics of the large-scale circulation in turbulent Rayleigh–Bénard convection. Phys. Fluids 20, 075101-1–16.Google Scholar
Brown, E., Funfschilling, D. & Ahlers, G. 2007 Anomalous Reynolds-number scaling in turbulent Rayleigh–Bénard convection. J. Stat. Mech. 2007, P10005.Google Scholar
Brown, E., Nikolaenko, A. & Ahlers, G. 2005 Reorientation of the large-scale circulation in turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 95, 084503.Google Scholar
Chavanne, X., Chilla, F., Chabaud, B., Castaing, B. & Hebral, B. 2001 Turbulent Rayleigh–Bénard convection in gaseous and liquid He. Phys. Fluids 13, 1300–1320.Google Scholar
Cross, M. C. & Hohenberg, P. C. 1993 Pattern formation outside of equilibrium. Rev. Mod. Phys. 65, 851–1112.Google Scholar
de Bruyn, J. R., Bodenschatz, E., Morris, S. W., Trainoff, S., Hu, Y., Cannell, D. S. & Ahlers, G. 1996 Apparatus for the study of Rayleigh–Bénard convection in gases under pressure. Rev. Sci. Instrum. 67, 2043.Google Scholar
Funfschilling, D., Brown, E. & Ahlers, G. 2008 Torsional oscillations of the large-scale circulation in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 607, 119–139.Google Scholar
Funfschilling, D., Brown, E., Nikolaenko, A. & Ahlers, G. 2005 Heat transport by turbulent Rayleigh–Bénard convection in cylindrical cells with aspect ratio one and larger. J. Fluid Mech. 536, 145–154.Google Scholar
Gracki, J. A., Flynn, G. P. & Ross, J. 1969 Viscosity of nitrogen, helium, hydrogen, and argon from $- 100$ to 25 C up to 150–250 atm. J. Chem. Phys. 51 (9), 3856–3863.Google Scholar
Grossmann, S. & Lohse, D. 1993 Characteristic scales in Rayleigh–Bénard turbulence. Phys. Lett. A 173, 58–62.Google Scholar
Grossmann, S. & Lohse, D. 2002 Prandtl and Rayleigh number dependence of the Reynolds number in turbulent thermal convection. Phys. Rev. E 66, 016305.CrossRefGoogle ScholarPubMed
Hartlep, T., Tilgner, A. & Busse, F. H. 2005 Transition to turbulent convection in a fluid layer heated from below at moderate aspect ratio. J. Fluid Mech. 544, 309–322.CrossRefGoogle Scholar
He, G.-W. & Zhang, J.-B. 2006 Elliptic model for space–time correlations in turbulent shear flows. Phys. Rev. E 73, 055303.Google Scholar
He, X., Ching, E. & Tong, P. 2011 Locally averaged thermal dissipation rate in turbulent thermal convection: a decomposition into contributions from different temperature gradient components. Phys. Fluids 23, 025106-1–13.Google Scholar
He, X., Funfschilling, D., Nobach, H., Bodenschatz, E. & Ahlers, G. 2012 Transition to the ultimate state of turbulent Rayleigh–Beńard convection. Phys. Rev. Lett. 108, 024502.CrossRefGoogle Scholar
He, X., He, G. & Tong, P. 2010 Small-scale turbulent fluctuations beyond Taylor’s frozen-flow hypothesis. Phys. Rev. E 81, 065303.Google Scholar
He, X. & Tong, P. 2011 Kraichnan’s random sweeping hypothesis in homogeneous turbulent convection. Phys. Rev. E 83, 037302.Google Scholar
Hilsenrath, J., Hoge, H. J., Beckett, C. W., Masi, J. F., Benedict, W. S., Nuttall, R. L., Fano, L., Touloukian, Y. S. & Woodley, H. W. 1960 Tables of Thermodynamic and Transport Properties of Air, Carbon Dioxide, Carbon Monoxide, Hydrogen, Nitrogen, Oxygen, and Steam. Pergamon.Google Scholar
Hoogland, J., van den Berg, H. & Trappeniers, N. 1985 Measurements of the viscosity of sulfur hexafluoride up to 100 bar by a capillary-flow viscometer. Physica A 134, 169–192.Google Scholar
Howard, R. & LaBonte, B. 1980 A search for large-scale convection cells in the solar atmosphere. Astrophys. J. 239, 738–745.Google Scholar
Kestin, J. & Imaishi, N. 1985 Int. J. Thermophys. 6, 107.Google Scholar
Krishnamurti, R. 1970 On the transition to turbulent convection. Part 2. The transition to time-dependent flow. J. Fluid Mech. 42, 309–320.CrossRefGoogle Scholar
Lam, S., Shang, X. D., Zhou, S. Q. & Xia, K.-Q. 2002 Prandtl-number dependence of the viscous boundary layer and the Reynolds-number in Rayleigh–Bénard convection. Phys. Rev. E 65, 066306.Google Scholar
Lappa, M. 2010 Thermal Convection: Patterns, Evolution and Stability. John Wiley & Sons.Google Scholar
Lemmon, E., Huber, M. & McLinden, M. 2007 NIST standard reference database 23: reference fluid thermodynamic and transport properties-REFPROP, version 8.0. National Institute of Standards and Technology, Standard Reference Data Program, Gaithersburg.Google Scholar
Liu, L. & Ahlers, G. 1997 Rayleigh–Bénard convection in binary gas mixtures: thermophysical properties and the onset of convection. Phys. Rev. E 55, 6950.CrossRefGoogle Scholar
Lohse, D. & Xia, K.-Q. 2010 Small-scale properties of turbulent Rayleigh–Bénard convection. Annu. Rev. Fluid Mech. 42, 335–364.Google Scholar
Niemela, J. J., Skrbek, L., Sreenivasan, K. R. & Donnelly, R. 2000 Turbulent convection at very high Rayleigh numbers. Nature 404, 837–840.Google Scholar
Nikolaenko, A., Brown, E., Funfschilling, D & Ahlers, G 2005 Heat transport by turbulent Rayleigh–Bénard convection in cylindrical cells with aspect ratio one and less. J. Fluid Mech. 523, 251–260.Google Scholar
Oda, A., Uematsu, M. & Watanabe, K. 1983 An equation of state for sulfur hexafluoride in the range of temperatures 222 to 500 K up to 50 MPa. Bull. JSME 26 (219), 1590–1596.Google Scholar
Qiu, X. L. & Tong, P. 2001 Large scale velocity structures in turbulent thermal convection. Phys. Rev. E 64, 036304.Google Scholar
Rahmstorf, S. 2000 The thermohaline ocean circulation: a system with dangerous thresholds?. Climate Change 46, 247–256.Google Scholar
Rasenat, S., Hartung, G., Winkler, B. & Rehberg, I. 1989 Exp. Fluids 7, 412–420.CrossRefGoogle Scholar
Sugiyama, K., Calzavarini, E., Grossmann, S. & Lohse, D. 2007 Non-Oberbeck–Boussinesq effects in two-dimensional Rayleigh–Bénard convection in glycerol. Europhys. Lett. 80, 34002.Google Scholar
Trainoff, S. P. & Cannell, D. S. 2002 Physical optics treatment of the shadowgraph. Phys. Fluids 14, 1340–1363.Google Scholar
Uribe, F., Mason, E. & Kestin, J. 1990 Thermal conductivity of nine polyatomic gases at low density. J. Phys. Chem. Ref. Data 19 (5), 1123–1136.Google Scholar
Verzicco, R. & Camussi, R. 1999 Prandtl number effects in convective turbulence. J. Fluid Mech. 383, 55–73.Google Scholar
Weiss, S. & Ahlers, G. 2011 Turbulent Rayleigh–Bénard convection in a cylindrical container with aspect ratio $\Gamma = 0. 50$ and Prandtl number $\mathit{Pr}= 4. 38$ . J. Fluid Mech. 676, 5–40.Google Scholar
Xi, H.-D. & Xia, K.-Q. 2008 Flow mode transition in turbulent thermal convection. Phys. Fluids 20, 055104.Google Scholar
Xia, K.-Q. 2007 Two clocks for a single engine in turbulent convection. J. Stat. Mech. N11001.Google Scholar
Xu, X., Bajaj, K. M. S. & Ahlers, G. 2000 Heat transport in turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 84, 4357–4360.Google Scholar
Zhao, X. & He, G.-W. 2009 Space–time correlations of fluctuating velocities in turbulent shear flows. Phys. Rev. E 79, 046316.Google Scholar
Zhong, J.-Q., Funfschilling, D. & Ahlers, G. 2009 Enhanced heat transport by turbulent two-phase Rayleigh–Bénard convection. Phys. Rev. Lett. 102, 124501.Google Scholar
Zhou, Q., Li, C.-M., Lu, Z.-M. & Liu, Y.-L. 2011 Experimental investigation of longitudinal space–time correlations of the velocity field in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 683, 94–111.CrossRefGoogle Scholar

Hogg Supplementary Material

Movie 1 corresponding to Fig. 1a of the main document, running at approximately half of real-time speed. 11 mm square in the center of the cell, with Pr = 0.18, Ra = 1x10^{6}, 11 pixels/mm

Download Hogg Supplementary Material(Video)
Video 1.2 MB

Hogg Supplementary Material

Movie 2 corresponding to Fig. 1b of the main document, running at approximately real-time speed. 94 mm square, with Pr=0.88, Ra=2x10^{7}, 5 pixels/mm.

Download Hogg Supplementary Material(Video)
Video 5.1 MB

Hogg Supplementary Material

Movie 3 corresponding to Fig. 7a of the main document, running at approximately real-time speed. It shows processed shadowgraph images corresponding to Fig. 1b for Pr = 0.88 and Ra = 2x10^{7}. Only the dark (relatively warm) structures near the bottom of the sample remain. A 94 mm square was used with a resolution of 5 pixels/mm.

Download Hogg Supplementary Material(Video)
Video 5.2 MB

Hogg Supplementary Material

Movie 4 corresponding to Fig. 7b of the main document, running at approximately real-time speed. It shows processed shadowgraph images corresponding to Fig. 1b for Pr = 0.88 and Ra = 2x10^{7}. Only the light (relatively cold) structures near the top of the sample remain. A 94 mm square was used with a resolution of 5 pixels/mm.

Download Hogg Supplementary Material(Video)
Video 5 MB