Published online by Cambridge University Press: 04 October 2018
This paper investigates the effect of a finite Reynolds number (FRN) on the flatness factor (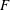 $F$) of the velocity derivative in decaying homogeneous isotropic turbulence by applying the eddy damped quasi-normal Markovian (EDQNM) method to calculate all terms in an analytic expression for
$F$) of the velocity derivative in decaying homogeneous isotropic turbulence by applying the eddy damped quasi-normal Markovian (EDQNM) method to calculate all terms in an analytic expression for  $F$ (Djenidi et al., Phys. Fluids, vol. 29 (5), 2017b, 051702). These terms and hence
$F$ (Djenidi et al., Phys. Fluids, vol. 29 (5), 2017b, 051702). These terms and hence 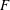 $F$ become constant when the Taylor microscale Reynolds number,
$F$ become constant when the Taylor microscale Reynolds number, 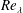 $Re_{\unicode[STIX]{x1D706}}$ exceeds approximately
$Re_{\unicode[STIX]{x1D706}}$ exceeds approximately 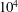 $10^{4}$. For smaller values of
$10^{4}$. For smaller values of 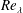 $Re_{\unicode[STIX]{x1D706}}$,
$Re_{\unicode[STIX]{x1D706}}$, 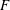 $F$, like the skewness
$F$, like the skewness 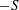 $-S$, increases with
$-S$, increases with 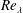 $Re_{\unicode[STIX]{x1D706}}$; this behaviour is in quantitative agreement with experimental and direct numerical simulation data. These results indicate that one must first ensure that
$Re_{\unicode[STIX]{x1D706}}$; this behaviour is in quantitative agreement with experimental and direct numerical simulation data. These results indicate that one must first ensure that 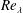 $Re_{\unicode[STIX]{x1D706}}$ is large enough for the FRN effect to be negligibly small before the hypotheses of Kolmogorov (Dokl. Akad. Nauk SSSR, vol. 30, 1941a, pp. 301–305; Dokl. Akad. Nauk SSSR, vol. 32, 1941b, pp. 16–18; J. Fluid Mech., vol. 13, 1962, pp. 82–85) can be assessed unambiguously. An obvious implication is that results from experiments and direct numerical simulations for which
$Re_{\unicode[STIX]{x1D706}}$ is large enough for the FRN effect to be negligibly small before the hypotheses of Kolmogorov (Dokl. Akad. Nauk SSSR, vol. 30, 1941a, pp. 301–305; Dokl. Akad. Nauk SSSR, vol. 32, 1941b, pp. 16–18; J. Fluid Mech., vol. 13, 1962, pp. 82–85) can be assessed unambiguously. An obvious implication is that results from experiments and direct numerical simulations for which 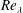 $Re_{\unicode[STIX]{x1D706}}$ is well below
$Re_{\unicode[STIX]{x1D706}}$ is well below 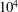 $10^{4}$ may not be immune from the FRN effect. Another implication is that a power-law increase of
$10^{4}$ may not be immune from the FRN effect. Another implication is that a power-law increase of  $F$ with respect to
$F$ with respect to  $Re_{\unicode[STIX]{x1D706}}$, as suggested by the Kolmogorov 1962 theory, is not tenable when
$Re_{\unicode[STIX]{x1D706}}$, as suggested by the Kolmogorov 1962 theory, is not tenable when 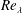 $Re_{\unicode[STIX]{x1D706}}$ is large enough.
$Re_{\unicode[STIX]{x1D706}}$ is large enough.