Hostname: page-component-586b7cd67f-vdxz6 Total loading time: 0 Render date: 2024-12-02T21:29:17.193Z Has data issue: false hasContentIssue false
  • English
  • Français

Rapid distortion theory analysis on the interaction between homogeneous turbulence and a planar shock wave

Published online by Cambridge University Press:  01 August 2016

T. Kitamura ,
K. Nagata ,
Y. Sakai ,
A. Sasoh  and
Y. Ito
T. Kitamura*
Affiliation:
Department of Mechanical Science and Engineering, Nagoya University, Nagoya, Aichi 464-8603, Japan
K. Nagata
Affiliation:
Department of Aerospace Engineering, Nagoya University, Nagoya, Aichi 464-8603, Japan
Y. Sakai
Affiliation:
Department of Mechanical Science and Engineering, Nagoya University, Nagoya, Aichi 464-8603, Japan
A. Sasoh
Affiliation:
Department of Aerospace Engineering, Nagoya University, Nagoya, Aichi 464-8603, Japan
Y. Ito
Affiliation:
Department of Mechanical Science and Engineering, Nagoya University, Nagoya, Aichi 464-8603, Japan
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The interactions between homogeneous turbulence and a planar shock wave are analytically investigated using rapid distortion theory (RDT). Analytical solutions in the solenoidal modes are obtained. Qualitative answers to unsolved questions in a report by Andreopoulos et al. (Annu. Rev. Fluid Mech., vol. 524, 2000, pp. 309–345) are provided within the linear theoretical framework. The results show that the turbulence kinetic energy (TKE) is increased after interaction with a shock wave and that the contributions to the amplification can be interpreted primarily as the combined effect of shock-induced compression, which is a direct consequence of the Rankine–Hugoniot relation, and the nonlinear effect, which is an indirect consequence of the Rankine–Hugoniot relation via the perturbation manner. For initial homogeneous axisymmetric turbulence, the amplification of the TKE depends on the initial degree of anisotropy. Furthermore, the increase in energy at high wavenumbers is confirmed by the one-dimensional spectra. The enstrophy is also increased; its increase is more significant than that of the TKE because of the significant increase in enstrophy at high wavenumbers. The vorticity components perpendicular to the shock-induced compressed direction are amplified more than the parallel vorticity component. These results strongly suggest that a high resolution is needed to obtain accurate results for the turbulence–shock-wave interaction. The integral length scales ($L$) and the Taylor microscales (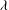$\unicode[STIX]{x1D706}$) are decreased for most cases after the interaction. However, 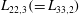$L_{22,3}(=\,L_{33,2})$ and $\unicode[STIX]{x1D706}_{22,3}(=\,\unicode[STIX]{x1D706}_{33,2})$ are amplified. Here, the subscripts 2 and 3 indicate the perpendicular components relative to the shock-induced compressed direction. The dissipation length and TKE dissipation rate are amplified.

JFM classification

Turbulent Flows: Homogeneous turbulence Compressible Flows: Shock waves
Type
Papers
Information
Journal of Fluid Mechanics , Volume 802 , 10 September 2016 , pp. 108 - 146
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Agui, J. H., Briassulis, G. & Andreopoulos, J. 2005 Studies of interactions of a propagating shock wave with decaying grid turbulence: velocity and vorticity fields. J. Fluid Mech. 524, 143–195.Google Scholar
Andreopoulos, J., Agui, J. H. & Briassulis, G. 2000 Shock wave–turbulence interaction. Annu. Rev. Fluid Mech. 524, 309–345.Google Scholar
Armstrong, J. W., Rickett, B. J. & Spranger, S. R. 1995 Electro density power spectrum in the local interstellar medium. Astrophys. J. 443, 209–221.Google Scholar
Barre, S., Alem, D. & Bonnet, J. P. 1996 Experimental study of a normal shock/homogeneous turbulence interaction. AIAA J. 34, 968–974.Google Scholar
Batchelor, G. K. 1946 The theory of axisymmetric turbulence. Proc. R. Soc. Lond. A 186, 480–502.Google Scholar
Batchelor, G. K. 1953 The Theory of Homogeneous Turbulence. Cambridge University Press.Google Scholar
Bertoglio, J. P., Bataille, F. & Marion, J. D. 2001 Two–point closures for weakly compressible turbulence. Phys. Fluids 13, 290–310.Google Scholar
Briassulis, G., Agui, J. H., Andreopoulos, J. & Watkins, C. B. 1996 A shock tube research facility for high-resolution measurements of compressible turbulence. Exp. Therm. Fluid Sci. 13, 430–446.Google Scholar
Cambon, C., Mansour, N. N. & Godefeferd, F. S. 1997 Energy transfer in rotating turbulence. J. Fluid Mech. 337, 303–332.Google Scholar
Cambon, C. & Teissèdre, C. 1985 Application des harmoniques sphériques à la représentation et au calcul des grandeurs cinématiques en turbulence homogène anisotrope. C. R. Acad. Sci. Paris II 301, 65–68.Google Scholar
Chandrasekhar, S. 1950 The theory of axisymetric turbulence. Phil. Trans. R. Soc. Lond. A 242, 557–577.Google Scholar
Craik, A. D. D. & Criminale, W. O. 1986 Evolution of wavelike disturbances in shear flows: a class of exact solutions of Navier–Stokes equations. Proc. R. Soc. Lond. A 406, 13–26.Google Scholar
Davidson, P. A. 2004 Turbulence: An Introduction for Scientists and Engineers. Oxford University Press.Google Scholar
Durbin, P. A. & Zeman, O. 1992 Rapid distortion theory for homogeneous compressed turbulence with application to modelling. J. Fluid Mech. 242, 349–370.Google Scholar
Grube, N. E., Taylor, E. M. & Martin, M. P.2011 Numerical investigation of shock wave/isotropic turbulence interaction. In 49th AIAA Aerospace Sciences Meeting.Google Scholar
Hanazaki, H. & Hunt, J. C. R. 1996 Linear processes in unsteady stably stratified turbulence. J. Fluid. Mech. 318, 303–337.Google Scholar
Hanazaki, H. & Hunt, J. C. R. 2004 Structure of unsteady stably stratified turbulence with mean shear. J. Fluid. Mech. 507, 1–42.Google Scholar
Hannappel, R. & Friedrich, R. 1995 Direct numerical simulation of a Mach 2 shock interacting with isotropic turbulence. Appl. Sci. Res. 54, 205–221.Google Scholar
Honkan, A. & Andreopoulos, J. 1992 Rapid compression of grid-generated turbulence by a moving shock wave. Phys. Fluids 4, 2562–2572.Google Scholar
Hunt, J. C. R. & Carruthers, D. J. 1990 Rapid distortion theory and the ‘problems’ of turbulence. J. Fluid Mech 212, 497–532.Google Scholar
Hunt, J. C. R. & Kevlahan, N. 1993 Rapid distortion theory and the structure of turbulence. In New Approaches and Concepts in Turbulence, pp. 285–316.Google Scholar
Jacquin, L., Cambon, C. & Blin, E. 1993 Turbulence amplification by a shock wave and rapid distortion theory. Phys. Fluids 5, 2539–2550.Google Scholar
Keller, J. & Merzkirch, W. 1990 Interaction of a normal shock wave with a compressible turbulent flow. Exp. Fluids 8, 241–248.Google Scholar
Kevlahan, N. K. R. 1997 The vorticity jump across a shock in a non-uniform flow. J. Fluid Mech. 341, 371–384.Google Scholar
Kevlahan, N. K. R. & Hunt, J. C. R. 1997 Nonlinear interactions in turbulence with strong irrotational straining. J. Fluid Mech. 337, 333–364.Google Scholar
Kitamura, T., Nagata, K., Sakai, Y., Sasoh, A., Terashima, O., Saito, H. & Harasaki, T. 2014 On invariants in grid turbulence at moderate Reynolds numbers. J. Fluid Mech. 738, 378–406.Google Scholar
Krogstad, P. Å. & Davidson, P. A. 2010 Is grid turbulence Saffman turbulence? J. Fluid Mech. 642, 373–394.Google Scholar
Larsson, J., Bermejo-Moreno, I. & Lele, S. K. 2013 Reynolds- and Mach-number effects in canonical shock–turbulence interaction. J. Fluid Mech. 717, 293–321.Google Scholar
Larsson, J. & Lele, S. K. 2009 Direct numerical simulation of canonical shock/turbulence interaction. Phys. Fluids 21, 126101.Google Scholar
Lee, L., Lele, S. K. & Moin, P. 1993 Direct numerical simulation of isotropic turbulence interacting with a weak shock wave. J. Fluid Mech. 251, 533–562.Google Scholar
Lee, L., Lele, S. K. & Moin, P. 1997 Interaction of isotropic turbulence with shock wave: effect of shock strength. J. Fluid Mech. 340, 225–247.Google Scholar
Mahesh, K., Lele, S. K. & Moin, P. 1994 The response of anisotropic turbulence to rapid homogeneous one-dimensional compression. Phys. Fluids 6, 1052–1062.Google Scholar
Mahesh, K., Lele, S. K. & Moin, P. 1997 The influence of entropy fluctuations on the interaction of turbulence with a shock wave. J. Fluid Mech. 84, 497–516.Google Scholar
Marion, J. D., Bertoglio, J. P. & Mathieu, J. 1988 Spectral study of weakly compressible isotropic turbulence: Part I: direct interaction approximation. C. R. Acad. Sci. Paris II 307, 1487–1492.Google Scholar
Miville-Deschenes, M. A., Joncas, G., Falgarone, E. & Boulanger, F. 2003 High resolution 21 cm mapping of the Ursa Major Galatic cirrus: power spectra of the high-latitude H i gas. Astron. Astrophys. 411, 109–121.Google Scholar
Nagata, K., Wong, H., Hunt, J. C. R., Sajjadi, S. G. & Davidson, P. A. 2006 Weak mean flows induced by anisotropic turbulence impinging onto planar and undulating surfaces. J. Fluid Mech. 556, 329–360.Google Scholar
Ribner, H. S. & Tucker, M.1953 Spectrum of turbulence in a contracting stream. NACA Rep. 1113.Google Scholar
Sagaut, P. & Cambon, C. 2008 Homogeneous Turbulence Dynamics. Cambridge University Press.Google Scholar
Sreenivasan, K. R. & Narasimha, R. 1978 Rapid distortion of axisymmetric turbulence. J. Fluid Mech. 84, 497–516.Google Scholar