Hostname: page-component-586b7cd67f-dlnhk Total loading time: 0 Render date: 2024-11-25T07:21:49.446Z Has data issue: false hasContentIssue false
  • English
  • Français

Pseudo-turbulent gas-phase velocity fluctuations in homogeneous gas–solid flow: fixed particle assemblies and freely evolving suspensions

Published online by Cambridge University Press:  31 March 2015

M. Mehrabadi ,
S. Tenneti ,
R. Garg  and
S. Subramaniam
M. Mehrabadi
Affiliation:
Center for Multiphase Flow Research, Department of Mechanical Engineering, Iowa State University, Ames, IA 50011, USA
S. Tenneti
Affiliation:
Center for Multiphase Flow Research, Department of Mechanical Engineering, Iowa State University, Ames, IA 50011, USA
R. Garg
Affiliation:
Center for Multiphase Flow Research, Department of Mechanical Engineering, Iowa State University, Ames, IA 50011, USA
S. Subramaniam*
Affiliation:
Center for Multiphase Flow Research, Department of Mechanical Engineering, Iowa State University, Ames, IA 50011, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Gas-phase velocity fluctuations due to mean slip velocity between the gas and solid phases are quantified using particle-resolved direct numerical simulation. These fluctuations are termed pseudo-turbulent because they arise from the interaction of particles with the mean slip even in ‘laminar’ gas–solid flows. The contribution of turbulent and pseudo-turbulent fluctuations to the level of gas-phase velocity fluctuations is quantified in initially ‘laminar’ and turbulent flow past fixed random particle assemblies of monodisperse spheres. The pseudo-turbulent kinetic energy 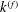$k^{(f)}$ in steady flow is then characterized as a function of solid volume fraction 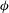${\it\phi}$ and the Reynolds number based on the mean slip velocity $\mathit{Re}_{m}$. Anisotropy in the Reynolds stress is quantified by decomposing it into isotropic and deviatoric parts, and its dependence on 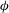${\it\phi}$ and 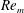$Re_{m}$ is explained. An algebraic stress model is proposed that captures the dependence of the Reynolds stress on 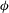${\it\phi}$ and 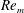$Re_{m}$. Gas-phase velocity fluctuations in freely evolving suspensions undergoing elastic and inelastic particle collisions are also quantified. The flow corresponds to homogeneous gas–solid systems, with high solid-to-gas density ratio and particle diameter greater than dissipative length scales. It is found that for the parameter values considered here, the level of pseudo-turbulence differs by only 15 % from the values for equivalent fixed beds. The principle of conservation of interphase turbulent kinetic energy transfer is validated by quantifying the interphase transfer terms in the evolution equations of kinetic energy for the gas-phase and solid-phase fluctuating velocity. It is found that the collisional dissipation is negligible compared with the viscous dissipation for the cases considered in this study where the freely evolving suspensions attain a steady state starting from an initial condition where the particles are at rest.

JFM classification

Turbulent Flows: Turbulence modelling Turbulent Flows: Turbulence simulation
Type
Papers
Information
Journal of Fluid Mechanics , Volume 770 , 10 May 2015 , pp. 210 - 246
Check for updates
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

‡

Present address: CD-Adapco, Lebanon, NH 03766, USA.

§

Present address: National Energy Technology Laboratory, Morgantown, WV 26508, USA.

References

Agrawal, K., Loezos, P. N., Syamlal, M. & Sundaresan, S. 2001 The role of meso-scale structures in rapid gas–solid flows. J. Fluid Mech. 445, 151–181.CrossRefGoogle Scholar
Ahmadi, G. & Ma., D. 1990a A thermodynamical formulation for dispersed multiphase turbulent flows: II simple shear flows for dense mixtures. Intl J. Multiphase Flow 16 (2), 341–351.Google Scholar
Ahmadi, G. & Ma, D. 1990b A thermodynamical formulation for dispersed multiphase turbulent flows: I basic theory. Intl J. Multiphase Flow 16 (2), 323–340.Google Scholar
Anderson, T. B. & Jackson, R. 1967 A fluid mechanical description of fluidized beds. Ind. Engng Chem. Fundam. 6, 527–539.Google Scholar
Bagchi, P. & Balachandar, S. 2003 Effect of turbulence on the drag and lift of a particle. Phys. Fluids 15 (11), 3496–3513.Google Scholar
Balachandar, S. & Eaton, J. K. 2010 Turbulent dispersed multiphase flow. Annu. Rev. Fluid Mech. 42, 111–133.Google Scholar
Balzer, G., Boelle, A. & Simonin, O. 1998 Eulerian gas–solid flow modelling of dense fluidized bed. In Fluidization VIII, International Symposium of Engineering Foundation, pp. 1125–1134. American Institute of Chemical Engineering.Google Scholar
Beetstra, R., van der Hoef, M. A. & Kuipers, J. A. M. 2007 Drag force of intermediate Reynolds number flows past mono- and bidisperse arrays of spheres. AIChE J. 53, 489–501.Google Scholar
Benyahia, S., Syamlal, M. & O’Brien, T. J. 2005 Evaluation of boundary conditions used to model dilute, turbulent gas/solids flows in a pipe. Powder Technol. 156, 62–72.CrossRefGoogle Scholar
Boivin, M., Simonin, O. & Squires, K. D. 1998 Direct numerical simulation of turbulence modulation by particles in isotropic turbulence. J. Fluid Mech. 375, 235–263.Google Scholar
Bolio, E. & Sinclair, J. 1995 Gas turbulence modulation in the pneumatic conveying of massive particles in vertical tubes. Intl J. Multiphase Flow 21 (6), 985–1001.Google Scholar
Bolio, E., Yasuna, J. & Sinclair, J. 1995 Dilute turbulent gas–solid flow in risers with particle–particle interactions. AIChE J. 41 (5), 1375–1388.Google Scholar
Breault, R. W., Guenther, C. P. & Shadle, L. J. 2008 Velocity fluctuation interpretation in the near wall region of a dense riser. Powder Technol. 182 (2), 137–145.Google Scholar
Burton, T. M. & Eaton, J. K. 2005 Fully resolved simulations of particle–turbulence interaction. J. Fluid Mech. 545, 67–111.Google Scholar
Calzavarini, E., Volk, R., Bourgoin, M., Leveque, E., Pinton, J. F. & Toschi, F. 2009 Acceleration statistics of finite-sized particles in turbulent flow: the role of Faxén forces. J. Fluid Mech. 630, 179–189.Google Scholar
Capecelatro, J. & Desjardins, O. 2013 An Euler–Lagrange strategy for simulating particle-laden flows. J. Comput. Phys. 238, 1–31.Google Scholar
Cate, A. T., Derksen, J. J., Portela, L. M. & van den Akker, H. E. A. 2004 Fully resolved simulations of colliding monodisperse spheres in forced isotropic turbulence. J. Fluid Mech. 519, 233–271.Google Scholar
Clift, R., Grace, J. R. & Weber, M. E. 1978 Bubbles, Drops and Particles. Academic.Google Scholar
Cocco, R., Shaffer, F., Hays, R., Reddy Karri, S. B. & Knowlton, T. 2010 Particle clusters in and above fluidized beds. Powder Technol. 203 (1), 3–11.Google Scholar
Cornish, R. J. 1928 Flow in a pipe of rectangular cross-section. Proc. R. Soc. Lond. A (786), 691–700.Google Scholar
Crowe, C. T. 2000 On models for turbulence modulation in fluid–particle flows. Intl J. Multiphase Flow 26, 719–727.Google Scholar
Crowe, C. T., Troutt, T. R. & Chung, J. N. 1996 Numerical models for two-phase turbulent flows. Annu. Rev. Fluid Mech. 28, 11–43.Google Scholar
Cundall, P. A. & Strack, O. D. L. 1979 A discrete numerical model for granular assemblies. Gèotechnique 29, 47–65.Google Scholar
Drew, D. A. 1983 Mathematical modeling of two-phase flow. Annu. Rev. Fluid Mech. 15, 261–291.Google Scholar
Drew, D. A. & Passman, S. L. 1998 Theory of Multicomponent Fluids. Springer.Google Scholar
Elghobashi, S. E. & Truesdell, G. C. 1993 On the two-way interaction between homogeneous turbulence and dispersed solid particles. I: turbulence modification. Phys. Fluids A 5, 1790–1801.Google Scholar
Ergun, S. 1952 Fluid flow through packed columns. Chem. Engng Prog. 48, 89–94.Google Scholar
Garg, R.2009 Modeling and simulation of two-phase flows. PhD thesis, Iowa State University.Google Scholar
Garg, R., Galvin, J., Li, T. & Pannala, S.2010a Documentation of open-source MFIX–DEM software for gas–solids flows. Tech. Rep. National Energy Technology Laboratory, Department of Energy.Google Scholar
Garg, R., Tenneti, S., Mohd-Yusof, J. & Subramaniam, S. 2010b Direct numerical simulation of gas–solids flow based on the immersed boundary method. In Computational Gas–Solids Flows and Reacting Systems: Theory, Methods and Practice (ed. Pannala, S., Syamlal, M. & O’Brien, T. J.). IGI Global.Google Scholar
Garzo, V., Tenneti, S., Subramaniam, S. & Hrenya, C. M. 2012 Enskog kinetic theory for monodisperse gas–solid flows. J. Fluid Mech. 712, 129–168.CrossRefGoogle Scholar
Gidaspow, D. 1994 Multiphase Flow and Fluidization. Academic.Google Scholar
Halvorsen, B., Guenther, C. & O’Brien, T. J. 2003 CFD calculations for scaling of a bubbling fluidized bed. In Proceedings of the AIChE Annual Meeting, pp. 16–21. AIChE.Google Scholar
Hertz, P. 1909 Über den gegenseitigen durchschnittlichen Abstand von Punkten, die mit bekannter mittlerer Dichte im Raume angeordnet sind. Math. Ann. 67, 387–398.Google Scholar
Hill, R. J., Koch, D. L. & Ladd, A. J. C. 2001a The first effects of fluid inertia on flows in ordered and random arrays of spheres. J. Fluid Mech. 448, 213–241.Google Scholar
Hill, R. J., Koch, D. L. & Ladd, A. J. C. 2001b Moderate-Reynolds-number flows in ordered and random arrays of spheres. J. Fluid Mech. 448, 243–278.CrossRefGoogle Scholar
van der Hoef, M. A., Beetstra, R. & Kuipers, J. A. M. 2005 Lattice-Boltzmann simulations of low-Reynolds-number flow past mono- and bidisperse arrays of spheres: results for the permeability and drag force. J. Fluid Mech. 528, 233–254.CrossRefGoogle Scholar
Homann, H. & Bec, J. 2010 Finite-size effects in the dynamics of neutrally buoyant particles in turbulent flow. J. Fluid Mech. 651, 81–91.CrossRefGoogle Scholar
Johnson, A. A. & Tezduyar, T. E. 1997 3D simulation of fluid–particle interactions with the number of particles reaching 100. Comput. Meth. Appl. Mech. Engng 145 (3–4), 301–321.Google Scholar
Kashiwa, B. A. & Gaffney, E. S.2003 Design Basis for CFDLib. Tech. Rep. LA-UR-03-1295. Los Alamos National Lab.Google Scholar
Kenning, V. M. & Crowe, C. T. 1997 On the effect of particles on carrier phase turbulence modulation in gas–particle flows. Intl J. Multiphase Flow 23 (2), 403–408.Google Scholar
Kidanemariam, A. G., Chan-Braun, C., Doychev, T. & Uhlmann, M. 2013 Direct numerical simulation of horizontal open channel flow with finite-size, heavy particles at low solid volume fraction. New J. Phys. 15 (2), 025031.Google Scholar
Kim, J. & Moin, P. 1985 Application of a fractional-step method to incompressible Navier–Stokes equations. J. Comput. Phys. 59, 308–323.Google Scholar
Koch, D. L. 1990 Kinetic theory for a monodisperse gas–solid suspension. Phys. Fluids A 2(10), 1711–1723.Google Scholar
Koch, D. L. & Sangani, A. S. 1999 Particle pressure and marginal stability limits for a homogeneous monodisperse gas-fluidized bed: kinetic theory and numerical simulations. J. Fluid Mech. 400, 229–263.Google Scholar
Lucci, F., Ferrante, A. & Elghobashi, S. 2010 Modulation of isotropic turbulence by particles of Taylor length-scale size. J. Fluid Mech. 650, 5–55.Google Scholar
Lucci, F., Ferrante, A. & Elghobashi, S. 2011 Is Stokes number an appropriate indicator for turbulence modulation by particles of Taylor-length-scale size? Phys. Fluids 23 (2), 025101.CrossRefGoogle Scholar
Mashayek, F. & Taulbee, D. B. 2002 Turbulent gas–solid flows, part I: direct numerical simulations and Reynolds stress closures. Numer. Heat Transfer Part B: Fundam. 41 (1), 1–29.CrossRefGoogle Scholar
Miller, A. & Gidaspow, D. 1992 Dense, vertical gas–solid flow in a pipe. AIChE J. 38 (11), 1801–1815.Google Scholar
Mohd-Yusof, J.1996 Interaction of massive particles with turbulence. PhD thesis, Cornell University.Google Scholar
Moran, J. C. & Glicksman, L. R. 2003 Mean and fluctuating gas phase velocities inside a circulating fluidized bed. Chem. Engng Sci. 58, 1867–1878.Google Scholar
Naso, A. & Prosperetti, A. 2010 The interaction between a solid particle and a turbulent flow. New J. Phys. 12 (3), 033040.Google Scholar
Pai, M. G. & Subramaniam, S. 2009 A comprehensive probability density function formalism for multiphase flows. J. Fluid Mech. 628, 181–228.CrossRefGoogle Scholar
Peskin, C. S. 2002 The immersed boundary method. Acta Numerica 11, 479–517.Google Scholar
Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.Google Scholar
Prosperetti, A. & Oguz, H. N. 2001 Physalis: a new $o(N)$ method for the numerical simulation of disperse systems: potential flow of spheres. J. Comput. Phys. 167 (1), 196–216.CrossRefGoogle Scholar
Pruppacher, H. R., Le Clair, B. P. & Hamielec, A. E. 1970 Some relations between drag and flow pattern of viscous flow past a sphere and a cylinder at low and intermediate Reynolds numbers. J. Fluid Mech. 44 (4), 781–790.CrossRefGoogle Scholar
Reddy, R. K., Jin, S., Nandakumar, K., Minev, P. D. & Joshi, J. B. 2010 Direct numerical simulation of free falling sphere in creeping flow. Intl J. Comput. Fluid Dyn. 24 (3–4), 109–120.Google Scholar
Reddy, R. K., Sathe, M. J., Joshi, J. B., Nandakumar, K. & Evans, G. M. 2013 Recent developments in experimental (PIV) and numerical (DNS) investigation of solid–liquid fluidized beds. Chem. Engng Sci. 92, 1–12.Google Scholar
Rogallo, R. S.1981 Numerical experiments in homogeneous turbulence. NASA Tech. Rep. 81835.Google Scholar
Sangani, A. S., Mo, G., Tsao, H.-K. W. & Koch, D. L. 1996 Simple shear flows of dense gas–solid suspensions at finite Stokes numbers. J. Fluid Mech. 313, 309–341.CrossRefGoogle Scholar
Schiller, L. & Naumann, A. 1935 A Drag Coefficient Correlation. V.D.I. Zeitung.Google Scholar
Squires, K. D. & Eaton, J. K. 1991 Measurements of particle dispersion obtained from direct numerical simulations of isotropic turbulence. J. Fluid Mech. 226, 1–35.Google Scholar
Stoyan, D., Kendall, W. S. & Mecke, J. 1995 Stochastic Geometry and its Applications, 2nd edn. John Wiley and Sons.Google Scholar
Subramaniam, S. 2000 Statistical representation of a spray as a point process. Phys. Fluids 12 (10), 2413–2431.Google Scholar
Subramaniam, S., Mehrabadi, M., Horwitz, J. & Mani, A. 2014 Developing improved Lagrangian point particle models of gas–solid flow from particle-resolved direct numerical simulation. In Studying Turbulence Using Numerical Simulation Databases-XV, Proceedings of the CTR 2014 Summer Program, pp. 5–14. Center for Turbulence Research, Stanford University, CA.Google Scholar
Sun, J., Battaglia, F. & Subramaniam, S. 2007 Hybrid two-fluid DEM simulation of gas–solid fluidized beds. Trans. ASME J. Fluids Engng 129 (11), 1394–1403.CrossRefGoogle Scholar
Sundaram, S. & Collins, L. R. 1999 A numerical study of the modulation of isotropic turbulence by suspended particles. J. Fluid Mech. 379, 105–143.Google Scholar
Syamlal, M. & O’Brien, T. J.1987 A generalized drag correlation for multiparticle systems. Tech. Rep. Morgantown Energy Technology Center DOE Report.Google Scholar
Tenneti, S., Garg, R., Hrenya, C. M., Fox, R. O. & Subramaniam, S. 2010 Direct numerical simulation of gas–solid suspensions at moderate Reynolds number: quantifying the coupling between hydrodynamic forces and particle velocity fluctuations. Powder Technol. 203 (1), 57–69.Google Scholar
Tenneti, S., Garg, R. & Subramaniam, S. 2011 Drag law for monodisperse gas–solid systems using particle-resolved direct numerical simulation of flow past fixed assemblies of spheres. Intl J. Multiphase Flow 37 (9), 1072–1092.Google Scholar
Tenneti, S. & Subramaniam, S. 2014 Particle-resolved direct numerical simulation for gas–solid flow model development. Annu. Rev. Fluid Mech. 46 (1), 199–230.Google Scholar
Tenneti, S., Sun, B., Garg, R. & Subramaniam, S. 2013 Role of fluid heating in dense gas–solid flow as revealed by particle-resolved direct numerical simulation. Intl J. Heat Mass Transfer 58 (1–2), 471–479.Google Scholar
Torquato, S., Lu, B. & Rubinstein, J. 1990 Nearest-neighbor distribution functions in many-body systems. Phys. Rev. A 41, 2059–2075.CrossRefGoogle ScholarPubMed
Uhlmann, M. 2008 Interface-resolved direct numerical simulation of vertical particulate channel flow in the turbulent regime. Phys. Fluids 20 (5), 053305.Google Scholar
Wen, C. Y. & Yu, Y. H. 1966 Mechanics of fluidization. Chem. Engng Prog. Symp. Ser. 62, 100–111.Google Scholar
Wylie, J. J., Koch, D. L. & Ladd, A. J. C. 2003 Rheology of suspensions with high particle inertia and moderate fluid inertia. J. Fluid Mech. 480, 95–118.Google Scholar
Xu, Y. & Subramaniam, S. 2007 Consistent modeling of interphase turbulent kinetic energy transfer in particle-laden turbulent flows. Phys. Fluids 19 (8), 085101.CrossRefGoogle Scholar
Xu, Y. & Subramaniam, S. 2010 Effect of particle clusters on carrier flow turbulence: a direct numerical simulation study. Flow Turbul. Combust. 85, 735–761.Google Scholar
Yin, X. & Koch, D. L. 2007 Hindered settling velocity and microstructure in suspensions of spheres with moderate Reynolds number. Phys. Fluids 19, 093302.CrossRefGoogle Scholar
Yin, X., Zenk, J. R., Mitrano, P. P. & Hrenya, C. M. 2013 Impact of collisional versus viscous dissipation on flow instabilities in gas–solid systems. J. Fluid Mech. 727, R2.Google Scholar
Zhang, Z. & Prosperetti, A. 2005 A second-order method for three-dimensional particle simulation. J. Comput. Phys. 210 (1), 292–324.CrossRefGoogle Scholar
Zhang, Y. H. & Reese, J. M. 2003 Gas turbulence modulation in a two-fluid model for gas–solid flows. AIChE J. 49 (12), 3048–3065.Google Scholar
Zick, A. A. & Homsy, G. M. 1982 Stokes flow through periodic arrays of spheres. J. Fluid Mech. 115, 13–26.Google Scholar