Published online by Cambridge University Press: 06 November 2015
We study the buoyancy-driven spreading of a thin viscous film over a thin elastic membrane. Neglecting the effects of membrane bending and the membrane weight, we study the case of constant fluid injection and obtain a system of coupled partial differential equations to describe the shape of the air–liquid interface, and the deformation and radial tension of the stretched membrane. We obtain self-similar solutions to describe the dynamics. In particular, in the early-time period, the dynamics is dominated by buoyancy-driven spreading of the liquid film, and membrane stretching is a response to the buoyancy-controlled distribution of liquid weight; the location of the liquid front obeys the power-law form 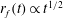 $r_{f}(t)\propto t^{1/2}$. However, in the late-time period, the system is quasi-steady, the air–liquid interface is flat, and membrane stretching, due to the liquid weight, causes the spreading of the liquid front; the location of the front obeys a different power-law form
$r_{f}(t)\propto t^{1/2}$. However, in the late-time period, the system is quasi-steady, the air–liquid interface is flat, and membrane stretching, due to the liquid weight, causes the spreading of the liquid front; the location of the front obeys a different power-law form  $r_{f}(t)\propto t^{1/4}$ before the edge effects of the membrane become significant. In addition, we report laboratory experiments for constant fluid injection using different viscous liquids and thin elastic membranes. Very good agreement is obtained between the theoretical predictions and experimental observations.
$r_{f}(t)\propto t^{1/4}$ before the edge effects of the membrane become significant. In addition, we report laboratory experiments for constant fluid injection using different viscous liquids and thin elastic membranes. Very good agreement is obtained between the theoretical predictions and experimental observations.