Hostname: page-component-669899f699-tzmfd Total loading time: 0 Render date: 2025-04-24T18:56:32.678Z Has data issue: false hasContentIssue false
  • English
  • Français

Progressive evolution of the viscous dissipation mechanism from the macroscale to the nanoscale

Published online by Cambridge University Press:  31 October 2024

Yi-Feng Wang ,
Zhi-Hui Cai ,
Yi-Bo Wang ,
Shu-Rong Gao ,
Yan-Ru Yang ,
Shao-Fei Zheng Open the ORCID record for Shao-Fei Zheng [Opens in a new window] ,
Duu-Jong Lee  and
Xiao-Dong Wang Open the ORCID record for Xiao-Dong Wang [Opens in a new window]
Yi-Feng Wang
Affiliation:
State Key Laboratory of Alternate Electrical Power System with Renewable Energy Sources, North China Electric Power University, Beijing 102206, PR China Research Center of Engineering Thermophysics, North China Electric Power University, Beijing 102206, PR China
Zhi-Hui Cai
Affiliation:
State Key Laboratory of Alternate Electrical Power System with Renewable Energy Sources, North China Electric Power University, Beijing 102206, PR China Research Center of Engineering Thermophysics, North China Electric Power University, Beijing 102206, PR China
Yi-Bo Wang
Affiliation:
State Key Laboratory of Alternate Electrical Power System with Renewable Energy Sources, North China Electric Power University, Beijing 102206, PR China Research Center of Engineering Thermophysics, North China Electric Power University, Beijing 102206, PR China
Shu-Rong Gao
Affiliation:
State Key Laboratory of Alternate Electrical Power System with Renewable Energy Sources, North China Electric Power University, Beijing 102206, PR China Research Center of Engineering Thermophysics, North China Electric Power University, Beijing 102206, PR China
Yan-Ru Yang
Affiliation:
State Key Laboratory of Alternate Electrical Power System with Renewable Energy Sources, North China Electric Power University, Beijing 102206, PR China Research Center of Engineering Thermophysics, North China Electric Power University, Beijing 102206, PR China
Shao-Fei Zheng*
Affiliation:
State Key Laboratory of Alternate Electrical Power System with Renewable Energy Sources, North China Electric Power University, Beijing 102206, PR China Research Center of Engineering Thermophysics, North China Electric Power University, Beijing 102206, PR China
Duu-Jong Lee*
Affiliation:
Department of Mechanical Engineering, City University of Hong Kong, Kowloon Tong 999077, Hong Kong Department of Chemical Engineering & Materials Science, Yuan Ze University, Chung-Li 32003, Taiwan
Xiao-Dong Wang*
Affiliation:
State Key Laboratory of Alternate Electrical Power System with Renewable Energy Sources, North China Electric Power University, Beijing 102206, PR China Research Center of Engineering Thermophysics, North China Electric Power University, Beijing 102206, PR China
*
†Email addresses for correspondence: [email protected], [email protected], [email protected]
†Email addresses for correspondence: [email protected], [email protected], [email protected]
†Email addresses for correspondence: [email protected], [email protected], [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

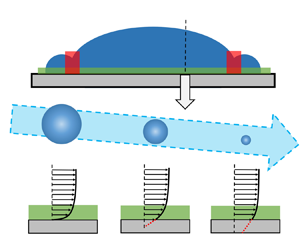
Recent studies of viscous dissipation mechanisms in impacting droplets have revealed distinct behaviours between the macroscale and nanoscale. However, the transition of these mechanisms from the macroscale to the nanoscale remains unexplored due to limited research at the microscale. This work addresses the gap using the many-body dissipative particle dynamics (MDPD) method. While the MDPD method omits specific atomic details, it retains crucial mesoscopic effects, making it suitable for investigating the impact dynamics at the microscale. Through the analysis of velocity contours within impacting droplets, the research identifies three primary contributors to viscous dissipation during spreading: boundary-layer viscous dissipation from shear flow; rim geometric head loss; and bulk viscous dissipation caused by droplet deformation. This prompts a re-evaluation of viscous dissipation mechanisms at both the macroscale and nanoscale. It reveals that the same three kinds of dissipation are present across all scales, differing only in their relative intensities at each scale. A model of the maximum spreading factor (βmax) incorporating all forms of viscous dissipation without adjustable parameters is developed to substantiate this insight. This model is validated against three distinct datasets representing the macroscale, microscale and nanoscale, encompassing a broad spectrum of Weber numbers, Ohnesorge numbers and contact angles. The satisfactory agreement between the model predictions and the data signifies a breakthrough in establishing a universal βmax model applicable across all scales. This model demonstrates the consistent nature of viscous dissipation mechanisms across different scales and underscores the importance of integrating microscale behaviours to understand macroscale and nanoscale phenomena.

JFM classification

Drops and Bubbles: Drops and Bubbles
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 998 , 10 November 2024 , A21
Check for updates
Copyright
© The Author(s), 2024. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Abolghasemibizaki, M., Dilmaghani, N., Mohammadi, R. & Castano, C.E. 2019 Viscous droplet impact on nonwettable textured surfaces. Langmuir 35, 10752–10761.CrossRefGoogle ScholarPubMed
Antonini, C., Amirfazli, A. & Marengo, M. 2012 Drop impact and wettability: from hydrophilic to superhydrophobic surfaces. Phys. Fluids 24, 102104.CrossRefGoogle Scholar
Attané, P., Girard, F. & Morin, V. 2007 An energy balance approach of the dynamics of drop impact on a solid surface. Phys. Fluids 19, 012101.CrossRefGoogle Scholar
Arienti, M., Pan, W., Li, X. & Karniadakis, G. 2011 Many-body dissipative particle dynamics simulation of liquid/vapor and liquid/solid interactions. J. Chem. Phys. 134, 204114.CrossRefGoogle ScholarPubMed
Bertola, V. 2009 An experimental study of bouncing Leidenfrost drops: comparison between Newtonian and viscoelastic liquids. Intl J. Heat Mass Transfer 52, 1786–1793.CrossRefGoogle Scholar
Bordbar, A., Taassob, A., Khojasteh, D., Marengo, M. & Kamali, R. 2018 Maximum spreading and rebound of a droplet impacting onto a spherical surface at low weber numbers. Langmuir 34, 5149–5158.CrossRefGoogle ScholarPubMed
Chandra, S. & Avedisian, C. 1991 On the collision of a droplet with a solid surface. Proc. R. Soc. A Math. Phys. Engng Sci. 432, 13–41.Google Scholar
Chen, H., Nie, Q. & Fang, H. 2020 Dynamic behavior of droplets on confined porous substrates: a many-body dissipative particle dynamics study. Phys. Fluids 32, 102003.CrossRefGoogle Scholar
Clanet, C., Béguin, C., Richard, D. & Quéré, D. 2004 Maximal deformation of an impacting drop. J. Fluid Mech. 517, 199–208.CrossRefGoogle Scholar
Du, B., Cheng, Y., Yang, S., Xu, W., Lan, Z., Wen, R. & Ma, X. 2020 Preferential vapor nucleation on hierarchical tapered nanowire bunches. Langmuir 37, 774–784.CrossRefGoogle ScholarPubMed
Du, J., Wang, X., Li, Y., Min, Q. & Wu, X. 2021 a Analytical consideration for the maximum spreading factor of liquid droplet impact on a smooth solid surface. Langmuir 37, 7582–7590.CrossRefGoogle ScholarPubMed
Du, J., Zhang, Y. & Min, Q. 2021 b Numerical investigations of the spreading and retraction dynamics of viscous droplets impact on solid surfaces. Colloids Surf. A 609, 125649.CrossRefGoogle Scholar
Eggers, J., Fontelos, M.A., Josserand, C. & Zaleski, S. 2010 Drop dynamics after impact on a solid wall: theory and simulations. Phys. Fluids 22, 062101.CrossRefGoogle Scholar
Gad-El-Hak, M. 1999 The fluid mechanics of microdevice – the Freeman scholar lecture. J. Fluids Engng 121, 5–33.CrossRefGoogle Scholar
Galliker, P., Schneider, J., Eghlidi, H., Kress, S., Sandoghdar, V. & Poulikakos, D. 2012 Direct printing of nanostructures by electrostatic autofocussing of ink nanodroplets. Nat. Commun. 3, 890.CrossRefGoogle ScholarPubMed
Ghoufi, A., Malfreyt, P. & Tildesley, D.J. 2016 Computer modelling of the surface tension of the gas–liquid and liquid–liquid interface. Chem. Soc. Rev. 45, 1387–1409.CrossRefGoogle ScholarPubMed
Glasscott, M.W., Pendergast, A.D., Goines, S., Bishop, A.R., Hoang, A.T., Renault, C. & Dick, J.E. 2019 Electrosynthesis of high-entropy metallic glass nanoparticles for designer, multi-functional electrocatalysis. Nat. Commun. 10, 2650.CrossRefGoogle ScholarPubMed
Groot, R.D. & Warren, P.B. 1997 Dissipative particle dynamics: bridging the gap between atomistic and mesoscopic simulation. J. Chem. Phys. 107, 4423–4435.CrossRefGoogle Scholar
Jamali, S., Boromand, A., Khani, S., Wagner, J., Yamanoi, M. & Maia, J. 2015 Generalized mapping of multi-body dissipative particle dynamics onto fluid compressibility and the Flory-Huggins theory. J. Chem. Phys. 142, 164902.CrossRefGoogle ScholarPubMed
Josserand, C. & Thoroddsen, S.T. 2016 Drop impact on a solid surface. Annu. Rev. Fluid Mech. 48, 365–391.CrossRefGoogle Scholar
Kobayashi, K., Konno, K., Yaguchi, H., Fujii, H., Sanada, T. & Watanabe, M. 2016 Early stage of nanodroplet impact on solid wall. Phys. Fluids 28, 032002.CrossRefGoogle Scholar
Koishi, T., Yasuoka, K. & Zeng, X.C. 2017 Molecular dynamics simulation of water nanodroplet bounce back from flat and nanopillared surface. Langmuir 33, 10184–10192.CrossRefGoogle ScholarPubMed
Kremer, J., Kilzer, A. & Petermann, M. 2018 Simultaneous measurement of surface tension and viscosity using freely decaying oscillations of acoustically levitated droplets. Rev. Sci. Instrum. 89, 015109.CrossRefGoogle ScholarPubMed
Laan, N., de Bruin, K.G., Bartolo, D., Josserand, C. & Bonn, D. 2014 Maximum diameter of impacting liquid droplets. Phys. Rev. Appl. 2, 044018.CrossRefGoogle Scholar
Lee, J.B., Derome, D., Dolatabadi, A. & Carmeliet, J. 2016 a Energy budget of liquid drop impact at maximum spreading: numerical simulations and experiments. Langmuir 32, 1279–1288.CrossRefGoogle ScholarPubMed
Lee, J.B., Laan, N., de Bruin, K.G., Skantzaris, G., Shahidzadeh, N., Derome, D., Carmeliet, J. & Bonn, D. 2016 b Universal rescaling of drop impact on smooth and rough surfaces. J. Fluid Mech. 786, R4.CrossRefGoogle Scholar
Li, B.X., Li, X.H. & Chen, M. 2017 Spreading and breakup of nanodroplet impinging on surface. Phys. Fluids 29, 012003.CrossRefGoogle Scholar
Li, X.H., Zhang, X.X. & Chen, M. 2015 Estimation of viscous dissipation in nanodroplet impact and spreading. Phys. Fluids 27, 052007.CrossRefGoogle Scholar
Li, Z., Bian, X., Tang, Y.H. & Karniadakis, G.E. 2018 A dissipative particle dynamics method for arbitrarily complex geometries. J. Comput. Phys. 355, 534–547.CrossRefGoogle Scholar
Li, Z., Hu, G.H., Wang, Z.L., Ma, Y.B. & Zhou, Z.W. 2013 Three dimensional flow structures in a moving droplet on substrate: a dissipative particle dynamics study. Phys. Fluids 25, 072103.CrossRefGoogle Scholar
Loth, E. 2008 Compressibility and rarefaction effects on drag of a spherical particle. AIAA J. 46, 2219–2228.CrossRefGoogle Scholar
Loth, E., Tyler Daspit, J., Jeong, M., Nagata, T. & Nonomura, T. 2021 Supersonic and hypersonic drag coefficients for a sphere. AIAA J. 59, 3261–3274.CrossRefGoogle Scholar
Madejski, J. 1976 Solidification of droplets on a cold surface. Intl J. Heat Mass Transfer 19, 1009–1013.CrossRefGoogle Scholar
Mao, T., Kuhn, D.C. & Tran, H. 1997 Spread and rebound of liquid droplets upon impact on flat surfaces. AIChE J. 43, 2169–2179.CrossRefGoogle Scholar
McBride, S.P. & Law, B.M. 2009 Viscosity-dependent liquid slip at molecularly smooth hydrophobic surfaces. Phys. Rev. E 80, 060601.CrossRefGoogle ScholarPubMed
Murtola, T., Bunker, A., Vattulainen, I., Deserno, M. & Karttunen, M. 2009 Multiscale modeling of emergent materials: biological and soft matter. Phys. Chem. Chem. Phys. 11, 1869–1892.CrossRefGoogle ScholarPubMed
Nie, Q.C., Zhong, Y.H. & Fang, H.S. 2019 Study of a nanodroplet breakup through many-body dissipative particle dynamics. Phys. Fluids 31, 042007.CrossRefGoogle Scholar
Pasandideh-Fard, M., Qiao, Y.M., Chandra, S. & Mostaghimi, J. 1996 Capillary effects during droplet impact on a solid surface. Phys. Fluids 8, 650–659.CrossRefGoogle Scholar
Srivastava, T. & Kondaraju, S. 2020 Analytical model for predicting maximum spread of droplet impinging on solid surfaces. Phys. Fluids 32, 092103.CrossRefGoogle Scholar
Stow, C.D. & Hadfield, M.G. 1981 An experimental investigation of fluid flow resulting from the impact of a water drop with an unyielding dry surface. Proc. R. Soc. A Math. Phys. Engng Sci. 373, 419–441.Google Scholar
Ukiwe, C. & Kwok, D.Y. 2005 On the maximum spreading diameter of impacting droplets on well-prepared solid surfaces. Langmuir 21, 666–673.CrossRefGoogle ScholarPubMed
Visser, C.W., Frommhold, P.E., Wildeman, S., Mettin, R., Lohse, D. & Sun, C. 2015 Dynamics of high-speed micro-drop impact: numerical simulations and experiments at frame-to-frame times below 100 ns. Soft Matt. 11, 1708–1722.CrossRefGoogle ScholarPubMed
Visser, C.W., Tagawa, Y., Sun, C. & Lohse, D. 2012 Microdroplet impact at very high velocity. Soft Matt. 8, 10732–10737.CrossRefGoogle Scholar
Wang, Y.-B., Wang, X.-D., Yang, Y.-R. & Chen, M. 2019 The maximum spreading factor for polymer nanodroplets impacting a hydrophobic solid surface. J. Phys. Chem. C 123, 12841–12850.CrossRefGoogle Scholar
Wang, Y.-B., Wang, Y.-F., Gao, S.-R., Yang, Y.-R., Wang, X.-D. & Chen, M. 2020 a Universal model for the maximum spreading factor of impacting nanodroplets: from hydrophilic to hydrophobic surfaces. Langmuir 36, 9306–9316.CrossRefGoogle ScholarPubMed
Wang, Y.-B., Wang, Y.-F., Wang, X., Zhang, B.-X., Yang, Y.-R., Lee, D.-J., Wang, X.-D. & Chen, M. 2021 b Splash of impacting nanodroplets on solid surfaces. Phys. Rev. Fluids 6, 094201.CrossRefGoogle Scholar
Wang, Y.-B., Wang, Y.-F., Yang, Y.-R., Wang, X.-D. & Chen, M. 2021 a Spreading time of impacting nanodroplets. J. Phys. Chem. B 125, 5630–5635.CrossRefGoogle ScholarPubMed
Wang, Y.-F., Wang, Y.-B., Cai, Z.-H., Ma, Q., Yang, Y.-R., Zheng, S.-F., Lee, D.-J. & Wang, X.D. 2024 Binary collision dynamics of equal-sized nanodroplets. J. Fluid Mech. 979, A25.CrossRefGoogle Scholar
Wang, Y.-F., Wang, Y.-B., He, X., Zhang, B.-X., Yang, Y.-R., Wang, X.-D. & Lee, D.-J. 2022 a Retraction dynamics of low-viscosity nanodroplets: from hydrophobic to hydrophilic surfaces. J. Mol. Liq. 355, 118963.CrossRefGoogle Scholar
Wang, Y.-F., Wang, Y.-B., He, X., Zhang, B.-X., Yang, Y.-R., Wang, X.-D. & Lee, D.-J. 2022 b Scaling laws of the maximum spreading factor for impact of nanodroplets on solid surfaces. J. Fluid Mech. 937, A12.CrossRefGoogle Scholar
Wang, Y.-F., Wang, Y.-B., Xie, F.-F., Liu, J.-Y., Wang, S.-L., Yang, Y.-R., Gao, S.-R. & Wang, X.-D. 2020 b Spreading and retraction kinetics for impact of nanodroplets on hydrophobic surfaces. Phys. Fluids 32, 092005.CrossRefGoogle Scholar
Wang, Y.-F., Wang, Y.-B., Zhang, C.-L., He, X., Yang, Y.-R., Zheng, S.-F., Lee, D.-J. & Wang, X.-D. 2023 Retraction and bouncing dynamics of nanodroplets upon impact on superhydrophobic surfaces. Phys. Fluids 35, 032012.CrossRefGoogle Scholar
Warren, P.B. 2001 Hydrodynamic bubble coarsening in off-critical vapor-liquid phase separation. Phys. Rev. Lett. 87, 225702.CrossRefGoogle ScholarPubMed
Warren, P.B. 2003 Vapor-liquid coexistence in many-body dissipative particle dynamics. Phys. Rev. E 68, 066702.CrossRefGoogle ScholarPubMed
Wildeman, S., Visser, C.W., Sun, C. & Lohse, D. 2016 On the spreading of impacting drops. J. Fluid Mech. 805, 636–655.CrossRefGoogle Scholar
Worthington, A.M. 1876 On the forms assumed by drops of liquids falling vertically on a horizontal plate. Pro. R. Soc. Lond. 25, 261–271.Google Scholar
Xie, F.F., Lv, S.H., Yang, Y.R. & Wang, X.D. 2020 Contact time of a bouncing nanodroplet. J. Phys. Chem. Lett. 11, 2818–2823.CrossRefGoogle ScholarPubMed
Yamada, T., Yuan, J. & Sunden, B.A. 2015 Application of many-body dissipative particle dynamics to determine liquid characteristics. Intl J. Numer. Meth. Heat Fluid Flow 25, 1619–1637.CrossRefGoogle Scholar
Yang, J.C., Qi, T.Y., Han, T.Y., Zhang, J. & Ni, M.J. 2018 Elliptical spreading characteristics of a liquid metal droplet impact on a glass surface under a horizontal magnetic field. Phys. Fluids 30, 012101.CrossRefGoogle Scholar
Yarin, A.L. & Weiss, D.A. 1995 Impact of drops on solid surfaces: self-similar capillary waves, and splashing as a new type of kinematic discontinuity. J. Fluid Mech. 283, 141–173.CrossRefGoogle Scholar
Zarin, N.A. 1970 Measurement of non-continuum and turbulence effects on subsonic sphere drag. NASA No. NASA-CR-1585.Google Scholar
Zhang, R., Farokhirad, S., Lee, T. & Koplik, J. 2014 Multiscale liquid drop impact on wettable and textured surfaces. Phys. Fluids 26, 082003.CrossRefGoogle Scholar
Zhao, J., Chen, S., Zhang, K. & Liu, Y. 2021 A review of many-body dissipative particle dynamics (MDPD): theoretical models and its applications. Phys. Fluids 33, 112002.CrossRefGoogle Scholar
Supplementary material: File

Wang et al. supplementary material

Wang et al. supplementary material
Download Wang et al. supplementary material(File)
File 463.7 KB