Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Li, Sheng
Liu, Haihu
Zhang, Jinggang
Jiang, Fei
and
Xi, Guang
2021.
Modeling of three-phase displacement in three-dimensional irregular geometries using a lattice Boltzmann method.
Physics of Fluids,
Vol. 33,
Issue. 12,
Zhang, Jinggang
Liu, Haihu
Wei, Bei
Hou, Jian
and
Jiang, Fei
2021.
Pore-Scale Modeling of Two-Phase Flows with Soluble Surfactants in Porous Media.
Energy & Fuels,
Vol. 35,
Issue. 23,
p.
19374.
Namaee-Ghasemi, Arman
Ayatollahi, Shahab
and
Mahani, Hassan
2021.
Pore-Scale Simulation of the Interplay between Wettability, Capillary Number, and Salt Dispersion on the Efficiency of Oil Mobilization by Low-Salinity Waterflooding.
SPE Journal,
Vol. 26,
Issue. 06,
p.
4000.
Liu, W.
Liu, Y. Y.
Yang, L. M.
Liu, Z. J.
Yuan, Z. Y.
Shu, C.
and
Teo, C. J.
2021.
Coupling improved discrete velocity method and G13-based gas kinetic flux solver: A hybrid method and its application for non-equilibrium flows.
Physics of Fluids,
Vol. 33,
Issue. 9,
He, Zhennan
Liang, Fachun
and
Meng, Jia
2022.
Pore-scale study of the effect of bifurcated fracture on spontaneous imbibition in heterogeneous porous media.
Physics of Fluids,
Vol. 34,
Issue. 7,
Liu, Fanli
and
Wang, Moran
2022.
Trapping patterns during capillary displacements in disordered media.
Journal of Fluid Mechanics,
Vol. 933,
Issue. ,
Li, Sheng
Liu, Haihu
Wu, Rui
Cai, Jianchao
Xi, Guang
and
Jiang, Fei
2022.
Prediction of spontaneous imbibition with gravity in porous media micromodels.
Journal of Fluid Mechanics,
Vol. 952,
Issue. ,
Wang, Zhongzheng
Pereira, Jean-Michel
Sauret, Emilie
and
Gan, Yixiang
2022.
Emergence of unstable invasion during imbibition in regular porous media.
Journal of Fluid Mechanics,
Vol. 941,
Issue. ,
Liu, Fanli
and
Wang, Moran
2022.
Phase diagram for preferential flow in dual permeable media.
Journal of Fluid Mechanics,
Vol. 948,
Issue. ,
Lasseux, Didier
and
Valdés-Parada, Francisco J.
2022.
A macroscopic model for immiscible two-phase flow in porous media.
Journal of Fluid Mechanics,
Vol. 944,
Issue. ,
Zhong, Huiying
He, Yuanyuan
Zhao, Xin
and
Peng, Xiaojuan
2022.
Theoretical Study on the Micro-Flow Mechanism of Polymer Flooding in a Double Heterogeneous Oil Layer.
Energies,
Vol. 15,
Issue. 9,
p.
3236.
Lei, Wenhai
Li, Qiangqiang
Yang, Hai-En
Wu, Tian-Jiang
Wei, Jiong
and
Wang, Moran
2022.
Preferential flow control in heterogeneous porous media by concentration-manipulated rheology of microgel particle suspension.
Journal of Petroleum Science and Engineering,
Vol. 212,
Issue. ,
p.
110275.
He, Zhennan
Liang, Fachun
and
Meng, Jia
2023.
Effects of injection directions and boundary exchange times on adaptive pumping in heterogeneous porous media: Pore-scale simulation.
Science of The Total Environment,
Vol. 867,
Issue. ,
p.
161427.
Gu, Qingqing
Zhang, Jinggang
Liu, Haihu
and
Wu, Lei
2023.
Numerical study of droplet behavior passing through a constricted square channel.
Physics of Fluids,
Vol. 35,
Issue. 7,
Shan, Fang
Chai, Zhenhua
Shi, Baochang
Xiao, Junfeng
and
Wang, Changli
2023.
Directional transport of a droplet on biomimetic ratchet structure.
Physics of Fluids,
Vol. 35,
Issue. 11,
Shi, Haidong
Zhu, Qingyuan
Chen, Zhangxin
Li, Jing
Feng, Dong
Zhang, Shengting
Ye, Jiawei
and
Wu, Keliu
2023.
Pore-scale modeling of water–gas flow in heterogeneous porous media.
Physics of Fluids,
Vol. 35,
Issue. 7,
Lei, Wenhai
Gong, Wenbo
and
Wang, Moran
2023.
Wettability effect on displacement in disordered media under preferential flow conditions.
Journal of Fluid Mechanics,
Vol. 975,
Issue. ,
Zhang, Jinggang
Cui, Haihang
Liu, Haihu
Chen, Li
Zhang, Xitong
and
Li, Chenlu
2023.
The Surfactant Role on a Droplet Passing through a Constricted Microchannel in a Pressure-Driven Flow: A Lattice Boltzmann Study.
Langmuir,
Vol. 39,
Issue. 38,
p.
13735.
Liang, Fachun
He, Zhennan
Meng, Jia
Zhao, Jingwen
and
Yu, Chao
2023.
Effects of microfracture parameters on adaptive pumping in fractured porous media: Pore-scale simulation.
Energy,
Vol. 263,
Issue. ,
p.
125950.
Fei, Linlin
Qin, Feifei
Zhao, Jianlin
Derome, Dominique
and
Carmeliet, Jan
2023.
Lattice Boltzmann modelling of isothermal two-component evaporation in porous media.
Journal of Fluid Mechanics,
Vol. 955,
Issue. ,
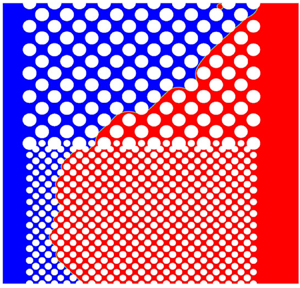
 $\lambda$ and capillary number
$\lambda$ and capillary number  $Ca_m$ which creatively incorporates the influence of channel width and length. Through the finite difference solution of the mathematical model, a
$Ca_m$ which creatively incorporates the influence of channel width and length. Through the finite difference solution of the mathematical model, a  $\lambda$–
$\lambda$– $Ca_m$ phase diagram is established to characterise the imbibition preference in the pore doublet. We then investigate the imbibition process in a dual-permeability pore network using a well-established lattice Boltzmann method, focusing on the competition between the viscous and capillary forces. Like in the pore doublet, the preferential imbibition occurs in high-permeability zone at high
$Ca_m$ phase diagram is established to characterise the imbibition preference in the pore doublet. We then investigate the imbibition process in a dual-permeability pore network using a well-established lattice Boltzmann method, focusing on the competition between the viscous and capillary forces. Like in the pore doublet, the preferential imbibition occurs in high-permeability zone at high  $Ca_{m}$ but in low-permeability zone at low
$Ca_{m}$ but in low-permeability zone at low  $Ca_{m}$. When
$Ca_{m}$. When  $Ca_m$ is not sufficiently high, an oblique advancing pattern is observed which is attributed to non-trivial interfacial tension. Using the newly defined capillary number, the critical
$Ca_m$ is not sufficiently high, an oblique advancing pattern is observed which is attributed to non-trivial interfacial tension. Using the newly defined capillary number, the critical  $Ca_{m}$ curve on which the breakthrough simultaneously occurs in both permeability zones is found to match well with that from the pore doublet and it is the optimal condition for maximising the imbibition efficiency in the entire pore network.
$Ca_{m}$ curve on which the breakthrough simultaneously occurs in both permeability zones is found to match well with that from the pore doublet and it is the optimal condition for maximising the imbibition efficiency in the entire pore network.