Hostname: page-component-5cf477f64f-rdph2 Total loading time: 0 Render date: 2025-04-07T22:24:56.023Z Has data issue: false hasContentIssue false
  • English
  • Français

Power-law exponent in the transition period of decay in grid turbulence

Published online by Cambridge University Press:  18 August 2015

L. Djenidi ,
Md. Kamruzzaman  and
R. A. Antonia
L. Djenidi*
Affiliation:
Discipline of Mechanical Engineering, School of Engineering, University of Newcastle, Newcastle, 2308 NSW, Australia
Md. Kamruzzaman
Affiliation:
Discipline of Mechanical Engineering, School of Engineering, University of Newcastle, Newcastle, 2308 NSW, Australia
R. A. Antonia
Affiliation:
Discipline of Mechanical Engineering, School of Engineering, University of Newcastle, Newcastle, 2308 NSW, Australia
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Hot-wire measurements are carried out in grid-generated turbulence at moderate to low Taylor microscale Reynolds number $Re_{{\it\lambda}}$ to assess the appropriateness of the commonly used power-law decay for the mean turbulent kinetic energy (e.g. 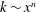$k\sim x^{n}$, with $n\leqslant -1$). It is found that in the region outside the initial and final periods of decay, which we designate a transition region, a power law with a constant exponent $n$ cannot describe adequately the decay of turbulence from its initial to final stages. One is forced to use a family of power laws of the form 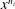$x^{n_{i}}$, where 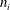$n_{i}$ is a different constant over a portion 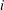$i$ of the decay time during the decay period. Accordingly, it is currently not possible to determine whether any grid-generated turbulence reported in the literature decays according to Saffman or Batchelor because the reported data fall in the transition period where $n$ differs from its initial and final values. It is suggested that a power law of the form 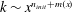$k\sim x^{n_{init}+m(x)}$, where 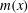$m(x)$ is a continuous function of $x$, could be used to describe the decay from the initial period to the final stage. The present results, which corroborate the numerical simulations of decaying homogeneous isotropic turbulence of Orlandi & Antonia (J. Turbul., vol. 5, 2004, doi:10.1088/1468-5248/5/1/009) and Meldi & Sagaut (J. Turbul., vol. 14, 2013, pp. 24–53), show that the values of $n$ reported in the literature, and which fall in the transition region, have been mistakenly assigned to the initial stage of decay.

JFM classification

Turbulent Flows: Homogeneous turbulence Turbulent Flows: Isotropic turbulence Turbulent Flows: Turbulent Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 779 , 25 September 2015 , pp. 544 - 555
Check for updates
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Batchelor, G. K. & Townsend, A. A. 1947 Decay of vorticity in isotropic turbulence. Proc. R. Soc. Lond. A 190, 534–550.Google Scholar
Batchelor, G. K. & Townsend, A. A. 1948 Decay of turbulence in the final period. Proc. R. Soc. Lond. A 194, 527–543.Google Scholar
Batchelor, G. K. 1948 Energy decay and self-preserving correlation functions in isotropic turbulence. Quart. Appl. Math. 6, 97–116.CrossRefGoogle Scholar
Batchelor, G. K. 1949 The role of big eddies in homogeneous turbulence. Proc. R. Soc. Lond. A 195, 513–532.Google Scholar
Bekritskaya, S. I. & Pavel’ev, A. A. 1974 On the power law of decay of grid turbulence. Izv. Akad. Nauk SSSR Mekh. Zhidk. Gaza 4, 170–172; (Engl. Trans.).Google Scholar
Comte-Bellot, G. & Corrsin, S. 1966 The use of a contraction to improve the isotopy of grid-generated turbulence. J. Fluid Mech. 25, 657–682.Google Scholar
Comte-Bellot, G. & Corrsin, S. 1971 Simple Eulerian time correlation of full- and narrow-band velocity signals in grid-generated, ‘isotropic’ turbulence. J. Fluid Mech. 48, 273–337.CrossRefGoogle Scholar
Corrsin, S. 1963 Turbulence: Experimental Methods. vol. 8. Springer.Google Scholar
Djenidi, L. & Antonia, R. A. 2014 Transport equation for the mean transport energy dissipation rate in low- $R_{{\it\lambda}}$ grid turbulence. J. Fluid Mech. 747, 288–315.Google Scholar
Djenidi, L. & Antonia, R. A. 2015 A general self-preservation analysis for decaying homogeneous isotropic turbulence. J. Fluid Mech. 773, 345–365.CrossRefGoogle Scholar
Gad-el-Hak, M. & Corrsin, S. 1974 Measurements of the nearly isotropic turbulence behind a uniform jet grid. J. Fluid Mech. 62, 115–143.Google Scholar
Goto, S. & Vassilocos, J. C. 2015 Energy dissipation and flux laws for unsteady turbulence. Phys. Lett. A 379 (16–17), 1144–1148.Google Scholar
Grant, H. L. & Nisbet, I. C. T 1957 The inhomogeneity of grid turbulence. J. Fluid Mech. 2, 263–272.CrossRefGoogle Scholar
Hearst, R. J. & Lavoie, P. 2014 Decay of turbulence generated by a square-fractal-element grid. J. Fluid Mech. 741, 567–584.Google Scholar
Huang, M.-J. & Leonard, A. 1994 Power-law decay of homogeneous turbulence at low Reynolds numbers. Phys. Fluids 480, 129–160.Google Scholar
Isaza, J. C., Salazar, R. & Warhaft, Z. 2014 On grid-generated turbulence in the near- and far field regions. J. Fluid Mech. 753, 402–426.Google Scholar
Kang, H. S., Chester, S. & Meneveau, C. 2003 Decaying turbulence in an active-grid-generated flow and comparisons with large eddy simulation. J. Fluid Mech. 480, 129–160.Google Scholar
Kistler, A. L. & Vrebalovich, T. 1966 Grid turbulence at large Reynolds numbers. J. Fluid Mech. 26, 37–47.CrossRefGoogle Scholar
Kolmogorov, A. 1941a Dissipation of energy in the locally isotropic turbulence. Dokl. Akad. Nauk SSSR 32, 16–18.Google Scholar
Kolmogorov, A. 1941b On the degeneration (decay) of isotropic turbulence in an incompressible viscous fluid. Dokl. Akad. Nauk SSSR 31, 538–540.Google Scholar
Krogstad, P.-Å. & Davidson, P. A. 2010 Is grid turbulence Saffman turbulence? J. Fluid Mech. 642, 373–394.Google Scholar
Larssen, J. V. & Davenport, W. J. 2001 On the generation of large-scale homogeneous turbulence. Exp. Fluids 50, 1207–1223.Google Scholar
Lavoie, P.2006 Effects of initial conditions in decaying grid turbulence. PhD thesis, University of Newcastle, Australia.Google Scholar
Lavoie, P., Djenidi, L. & Antonia, R. A. 2007 Effects of initial conditions in decaying turbulence generated by passive grids. J. Fluid Mech. 585, 395–420.Google Scholar
Lee, S. K., Benaissa, A., Djenidi, L., Lavoie, P. & Antonia, R. A. 2012 Scaling range of velocity and passive scalar spectra in grid turbulence. Phys. Fluids 24, 075101.Google Scholar
Lee, S. K., Djenidi, L., Antonia, R. A. & Danaila, L. 2013 On the destruction coefficients for slightly heated decaying grid turbulence. Intl J. Heat Fluid Flow 43, 129–136.Google Scholar
Ling, S. C. & Huang, T. T. 1970 Decay of weak turbulence. Phys. Fluids 13, 2912–2914.Google Scholar
Meldi, M. & Sagaut, P. 2013 Further insights into self-similarity and self-preservation in freely decaying isotropic turbulence. J. Turbul. 14, 24–53.Google Scholar
Mohamed, M. S. & LaRue, J. C. 1990 The decay power law in grid-generated turbulence. J. Fluid Mech. 219, 195–214.Google Scholar
Mydlarski, L. & Warhaft, Z. 1996 On the onset of high-Reynolds number grid-generated wind tunnel turbulence. J. Fluid Mech. 320, 331–368.Google Scholar
Mydlarski, L. & Warhaft, Z. 1998 Passive scalar statistics in high-Peclet-number grid turbulence. J. Fluid Mech. 358, 135–175.CrossRefGoogle Scholar
Orlandi, P. & Antonia, R. A. 2004 Dependence of a passive scalar in decaying isotropic turbulence on the Reynolds and Schmidt number using the EDQNM model. J. Turbul. 5, doi:10.1088/1468-5248/5/1/009.CrossRefGoogle Scholar
Saffman, P. G. 1967 Note on decay of homogeneous turbulence. Phys. Fluids 10, 1349.CrossRefGoogle Scholar
Schedvin, J., Stegen, G. R. & Gibson, C. H. 1974 Universal similarity at high grid Reynolds numbers. J. Fluid Mech. 65, 561–579.Google Scholar
Sinhuber, M., Bodenschatz, E. & Bewley, G. P. 2015 Decay of turbulence at high Reynolds numbers. Phys. Rev. Lett. 114, 034501.Google Scholar
Tan, H. S. & Ling, S. C. 1963 Final stage decay of grid produced turbulence. Phys. Fluids 6, 1693–1699.Google Scholar
Tavoularis, S., Bennett, J. C. & Corrsin, S. 1978 Velocity-derivative skewness in small Reynolds number, nearly isotropic turbulence. J. Fluid Mech. 88, 63–69.Google Scholar
Townsend, A. A. 1956 The Structure of Turbulent Shear Flow. Cambridge University Press.Google Scholar
Taylor, G. I. 1935 Statistical theory of turbulence. Proc. R. Soc. Lond. A 151, 412–478.Google Scholar
Valente, P. C. & Vassilicos, J. C. 2011 The decay of turbulence generated by a class of multiscale grids. J. Fluid Mech. 687, 300–430.Google Scholar
Zhou, T. & Antonia, R. A. 2000 Reynolds number dependence of the small-scale structure of grid turbulence. J. Fluid Mech. 406, 81–107.CrossRefGoogle Scholar