Hostname: page-component-586b7cd67f-2brh9 Total loading time: 0 Render date: 2024-11-24T16:57:18.280Z Has data issue: false hasContentIssue false
  • English
  • Français

Particle image velocimetry study of fractal-generated turbulence

Published online by Cambridge University Press:  12 September 2012

R. Gomes-Fernandes ,
B. Ganapathisubramani  and
J. C. Vassilicos
R. Gomes-Fernandes*
Affiliation:
Department of Aeronautics, Imperial College London, London SW7 2AZ, UK
B. Ganapathisubramani
Affiliation:
Aerodynamics & Flight Mechanics Research Group, University of Southampton, Southampton SO17 1BJ, UK
J. C. Vassilicos
Affiliation:
Department of Aeronautics, Imperial College London, London SW7 2AZ, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

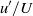An experimental investigation involving space-filling fractal square grids is presented. The flow is documented using particle image velocimetry (PIV) in a water tunnel as opposed to previous experiments which mostly used hot-wire anemometry in wind tunnels. The experimental facility has non-negligible incoming free-stream turbulence (with 2.8 % and 4.4 % in the streamwise () and spanwise (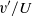) directions, respectively) which presents a challenge in terms of comparison with previous wind tunnel results. An attempt to characterize the effects of the incoming free stream turbulence on the grid-generated turbulent flow is made and an improved wake-interaction length scale is proposed which enables the comparison of the present results with previous ones for both fractal square and regular grids. This length scale also proves to be a good estimator of the turbulence intensity peak location. Furthermore, a new turbulence intensity normalization capable of collapsing 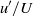for various grids in various facilities is proposed. Comparison with previous experiments indicates good agreement in turbulence intensities, Taylor microscale, as well as various other quantities, if the improved wake-interaction length scale is used. Global and local isotropy of fractal-generated turbulence is assessed using the velocity gradients of the two-component (2C) two-dimensional (2D) PIV and compared with regular grid results. Finally, the PIV data appear to confirm the new dissipation behaviour previously observed in hot-wire measurements.

JFM classification

Turbulent Flows: Turbulence control Turbulent Flows: Turbulence theory Wakes/Jets: Wakes
Type
Papers
Information
Journal of Fluid Mechanics , Volume 711 , 25 November 2012 , pp. 306 - 336
Copyright
Copyright © Cambridge University Press 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Adrian, R. J. & Westerweel, J. 2011 Particle Image Velocimetry. Cambridge University Press.Google Scholar
2. Aly, A., Abou, E.-A., Chong, A., Nicolleau, F. & Beck, S. 2010 Experimental study of the pressure drop after fractal-shaped orifices in turbulent pipe flows. Exp. Therm. Fluid Sci. 34 (1), 104–111.Google Scholar
3. Anderson, W. & Meneveau, C. 2011 Dynamic roughness model for large-eddy simulation of turbulent flow over multiscale, fractal-like rough surfaces. J. Fluid Mech. 679, 288–314.CrossRefGoogle Scholar
4. Antonia, R. A., Zhou, T. & Romano, G. P. 2002 Small-scale turbulence characteristics of two-dimensional bluff body wakes. J. Fluid Mech. 459, 67–92.CrossRefGoogle Scholar
5. Bai, K., Meneveau, C. & Katz, J. 2012 Near-wake turbulent flow structure and mixing length downstream of a fractal tree. Boundary-Layer Meteorol. 143, 285–308.CrossRefGoogle Scholar
6. Batchelor, G. K. 1953 The Theory of Homogeneous Turbulence. Cambridge University Press.Google Scholar
7. Bearman, P. W. & Trueman, D. M. 1972 An investigation of the flow around rectangular cylinders. Aeronaut. Q. 23, 229–237.CrossRefGoogle Scholar
8. Biferale, L., Cencini, M., Lanotte, A. S., Sbragaglia, M. & Toschi, 2004 Anomalous scaling and universality in hydrodynamic systems with power-law forcing. New J. Phys. 6, 37.CrossRefGoogle Scholar
9. Browne, L. W. B., Antonia, R. A. & Shah, D. A. 1987 Turbulent energy dissipation in a wake. J. Fluid Mech. 179, 307–326.CrossRefGoogle Scholar
10. Burattini, P., Lavoie, P. & Antonia, R. A. 2005 On the normalized turbulent energy dissipation rate. Phys. Fluids 17, 098103.CrossRefGoogle Scholar
11. Buxton, O. R. H. 2011Fine scale features of turbulent shear flows. PhD thesis, Imperial College London.Google Scholar
12. Cardesa-Dueñas, J. I., Nickels, T. B. & Dawson, J. R. 2012 2D PIV measurements in the near field of grid turbulence using stitched fields from multiple cameras. Exp. Fluids 52, 1611–1627.CrossRefGoogle Scholar
13. Cheskidov, A. & Doering, C. R. 2007 Energy dissipation in fractal-forced flow. J. Math. Phys. 48, 065208.CrossRefGoogle Scholar
14. Chester, S. & Meneveau, C. 2007 Renormalized numerical simulation of flow over planar and non-planar fractal trees. Environ. Fluid Mech. 7, 280–301.CrossRefGoogle Scholar
15. Chester, S., Meneveau, C. & Parlange, M. B. 2007 Modeling turbulent flow over fractal trees with renormalized numerical simulation. J. Comput. Phys. 225 (1), 427–448.CrossRefGoogle Scholar
16. Dallas, V., Vassilicos, J. C. & Hewitt, G. F. 2009 Stagnation point von Karman coefficient. Phys. Rev. E 80, 046306.CrossRefGoogle Scholar
17. Discetti, S., Ziskin, I. B., Adrian, R. J. & Prestridge, K. 2011 PIV study of fractal grid turbulence. In 9th International Symposium on Particle Image Velocimetry – PIV’11, Kobe, Japan. Visualization Society of Japan.Google Scholar
18. Doering, C. R. & Foias, C. 2002 Energy dissipation in body-forced turbulence. J. Fluid Mech. 467, 289–306.CrossRefGoogle Scholar
19. Eames, I., Jonsson, C. & Johnson, P. B. 2011 The growth of a cylinder wake in turbulent flow. J. Turbul. 12, 1–16.CrossRefGoogle Scholar
20. Geipel, P., Goh, K. H. H. & Lindstedt, R. P. 2010 Fractal-generated turbulence in opposed jet flows. Flow Turbul. Combust. 85 (3–4), 397–419.CrossRefGoogle Scholar
21. George, W. K. 1992 The decay of homogeneous turbulence. Phys. Fluids A 4, 1492.CrossRefGoogle Scholar
22. George, W. K. & Hussein, H. J. 1991 Locally axisymmetric turbulence. J. Fluid Mech. 233, 1–23.CrossRefGoogle Scholar
23. Groth, J. & Johansson, A. V. 1988 Turbulence reduction by screens. J. Fluid Mech. 197, 139–155.CrossRefGoogle Scholar
24. Hopfinger, E. J. & Toly, J. A. 1976 Spatially decaying turbulence and its relation to mixing across density interfaces. J. Fluid Mech. 78, 155–175.CrossRefGoogle Scholar
25. Hurst, D. & Vassilicos, J. C. 2007 Scalings and decay of fractal-generated turbulence. Phys. Fluids 19, 035103.CrossRefGoogle Scholar
26. Kang, H. K., Dennis, D. & Meneveau, C. 2011 Flow over fractals: drag forces and near wakes. Fractals 19, 387–399.CrossRefGoogle Scholar
27. Keylock, C. J., Nishimura, K., Nemoto, M. & Ito, Y. 2012 The flow structure in the wake of a fractal fence and the absence of an inertial regime. Environ. Fluid Mech. 12, 227–250.CrossRefGoogle Scholar
28. Kinzel, M., Wolf, M., Holzner, M., Lüthi, B., Tropea, C. & Kinzelbach, W. 2011 Simultaneous two-scale 3D-PTV measurements in turbulence under the influence of system rotation. Exp. Fluids 51 (1), 75–82.CrossRefGoogle Scholar
29. Kuczaj, A. K. & Geurts, B. J. 2006 Mixing in manipulated turbulence. J. Turbul. 7, 1–35.CrossRefGoogle Scholar
30. Kuczaj, A. K., Geurts, B. J. & McComb, W. D. 2006 Non local modulation of the energy cascade in broadband-forced turbulence. Phys. Rev. E 74, 016306.CrossRefGoogle Scholar
31. Laizet, S., Fortuné, V., Lamballais, V. & Vassilicos, J. C. 2012 Low Mach number prediction of the acoustic signature of fractal-generated turbulence. Intl J. Heat Fluid Flow 35, 25–32.CrossRefGoogle Scholar
32. Laizet, S., Lamballais, E. & Vassilicos, J. C. 2010 A numerical strategy to combine high-order schemes, complex geometry and parallel computing for high resolution dns of fractal generated turbulence. Comput. Fluids 39 (3), 471–484.CrossRefGoogle Scholar
33. Laizet, S. & Vassilicos, J. C. 2011 DNS of fractal-generated turbulence. Flow Turbul. Combust. 87, 673–705.CrossRefGoogle Scholar
34. Laizet, S. & Vassilicos, J. C. 2012 The fractal space-scale unfolding mechanism for energy-efficient turbulent mixing. Phys. Rev. Lett. (submitted).Google ScholarPubMed
35. Mazellier, N. & Vassilicos, J. C. 2008 The turbulence dissipation constant is not universal because of its universal dependence on large-scale flow topology. Phys. Fluids 20, 014102.CrossRefGoogle Scholar
36. Mazellier, N. & Vassilicos, J. C. 2010 Turbulence without Richardson–Kolmogorov cascade. Phys. Fluids 22, 075101.CrossRefGoogle Scholar
37. Mazzi, B., Okkels, F. & Vassilicos, J. C. 2002 A shell-model approach to fractal-induced turbulence. Eur. J. Mech. (B/Fluids) 28 (2), 231–241.Google Scholar
38. Mazzi, B. & Vassilicos, J. C. 2004 Fractal-generated turbulence. J. Fluid Mech. 502, 65–87.CrossRefGoogle Scholar
39. Nagata, K., Suzuki, H., Sakai, H., Hayase, Y. & Kubo, T. 2008a Direct numerical simulation of turbulence characteristics generated by fractal grids. Intl Rev. Phys. 5, 400–409.Google Scholar
40. Nagata, K., Suzuki, H., Sakai, H., Hayase, Y. & Kubo, T. 2008b Direct numerical simulation of turbulent mixing in grid-generated turbulence. Phys. Scr. 132, 014054.CrossRefGoogle Scholar
41. Nakamura, Y. & Tomonari, Y. 1976 The effect of turbulence on the drags of rectangular prisms. Trans. Japan Soc. Aeronaut. Space Sci. 19, 81–86.Google Scholar
42. Nedic, J., Ganapathisubramani, B., Vassilicos, J. C., Boree, J., Brizzi, L. E. & Spohn, A. 2012 Aero-acoustic performance of fractal spoilers. AIAA J. (in press).CrossRefGoogle Scholar
43. Nicolleau, F., Salim, S. & Nowakowski, A. F. 2011 Experimental study of a turbulent pipe flow through a fractal plate. J. Turbul. 12, 1–20.CrossRefGoogle Scholar
44. Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.CrossRefGoogle Scholar
45. Queiros-Conde, D. & Vassilicos, J. C. 2001 Turbulent wakes of 3-D fractal grids. In Intermittency in Turbulent Flows and Other Dynamical Systems (ed. Vassilicos, J. C. ). Cambridge University Press.Google Scholar
46. Saarenrinne, P. & Piirto, M. 2000 Turbulent kinetic energy dissipation rate estimation from PIV vector fields. Exp. Fluids 29, S300–S307.CrossRefGoogle Scholar
47. Seoud, R. E. & Vassilicos, J. C. 2007 Dissipation and decay of fractal-generated turbulence. Phys. Fluids 19, 105108.CrossRefGoogle Scholar
48. Soloff, S. M., Adrian, R. J. & Liu, Z.-C. 1997 Distortion compensation for generalized stereoscopic particle image velocimetry. Meas. Sci. Technol. 8, 1441–1454.CrossRefGoogle Scholar
49. Staicu, A., Mazzi, B., Vassilicos, J. C. & van de Water, W. 2003 Turbulent wakes of fractal objects. Phys. Rev. E 67 (6), 066306.CrossRefGoogle ScholarPubMed
50. Stresing, R., Peinke, J., Seoud, R. E. & Vassilicos, J. C. 2010 Defining a new class of turbulent flows. Phys. Rev. Lett. 104, 194501.CrossRefGoogle ScholarPubMed
51. Suzuki, H., Nagata, K., Sakai, Y. & Ukai, R. 2010 High-Schmidt-number scalar transfer in regular and fractal grid turbulence. Phys. Scr. T142, 014069.CrossRefGoogle Scholar
52. Symes, C. R. & Fink, L. E. 1977 Effects of external turbulence upon the flow past cylinders. In Structure and Mechanisms of Turbulence I (ed. Fiedler, H. ), pp. 86–102. Springer.Google Scholar
53. Tanaka, T. & Eaton, J. K. 2007 A correction method for measuring turbulence kinetic energy dissipation rate by PIV. Exp. Fluids 42, 893–902.CrossRefGoogle Scholar
54. Taylor, G. I. 1935 Statistical theory of turbulence. Proc. R. Soc. Lond. A 151, 421–478.CrossRefGoogle Scholar
55. Tennekes, H. & Lumley, J. L. 1972 A First Course in Turbulence. MIT.CrossRefGoogle Scholar
56. Townsend, A. A. 1956 The Structure of Turbulent Shear Flows. Cambridge University Press.Google Scholar
57. Tsinober, A., Kit, E. & Dracos, T. 1992 Experimental investigation of the field of velocity gradients in turbulent flows. J. Fluid Mech. 242, 169–192.CrossRefGoogle Scholar
58. Valente, P. C. & Vassilicos, J. C. 2011a The decay of turbulence generated by a class of multi-scale grids. J. Fluid Mech. 687, 300–340.CrossRefGoogle Scholar
59. Valente, P. C. & Vassilicos, J. C. 2011b Comment on dissipation and decay of fractal-generated turbulence [Phys. Fluids 19, 105108 (2007)]. Phys. Fluids 23, 119101.CrossRefGoogle Scholar
60. Valente, P. C. & Vassilicos, J. C. 2012a Dependence of decaying homogeneous isotropic turbulence on inflow conditions. Phys. Lett. A 376, 510–514.CrossRefGoogle Scholar
61. Valente, P. C. & Vassilicos, J. C. 2012b Universal dissipation scaling for non-equilibrium turbulence. Phys. Rev. Lett. 108, 214503.CrossRefGoogle Scholar
62. Wygnanski, I., Champagne, F. & Marasli, B. 1986 On the large-scale structures in two-dimensional, small-deficit, turbulent wakes. J. Fluid Mech. 168, 31–71.CrossRefGoogle Scholar
63. Zheng, H. W., Nicolleau, F. & Qin, N. 2012 Detached eddy simulation for turbulent flows in a pipe with a snowflake fractal orifice. In New Approaches in Modeling Multiphase Flows and Dispersion in Turbulence, Fractal Methods and Synthetic Turbulence (ed. Nicolleau, F. C. G. A., Cambon, C., Redondo, J.-M., Vassilicos, J. C., Reeks, M. & Nowakowski, A. F. ). Springer.Google Scholar