Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Posa, A.
Broglia, R.
and
Balaras, E.
2022.
Recovery in the wake of in-line axial-flow rotors.
Physics of Fluids,
Vol. 34,
Issue. 4,
Li, Zhaobin
Liu, Xiaohao
and
Yang, Xiaolei
2022.
Review of Turbine Parameterization Models for Large-Eddy Simulation of Wind Turbine Wakes.
Energies,
Vol. 15,
Issue. 18,
p.
6533.
Jin, Yaqing
Cheng, Shyuan
and
Chamorro, Leonardo P.
2022.
On the impact of layout in the dynamics of wind turbine arrays under passive oscillations.
Journal of Renewable and Sustainable Energy,
Vol. 14,
Issue. 3,
Xin, Zhiqiang
Liu, Songyang
Cai, Zhiming
Liao, Shenghai
and
Huang, Guoqing
2022.
Numerical Study on the Yaw Control for Two Wind Turbines under Different Spacings.
Applied Sciences,
Vol. 12,
Issue. 14,
p.
7098.
Ramos‐García, Néstor
González Horcas, Sergio
Pegalajar‐Jurado, Antonio
Kontos, Stavros
and
Bredmose, Henrik
2022.
Investigation of the floating IEA wind 15‐MW RWT using vortex methods Part II: Wake impact on downstream turbines under turbulent inflow.
Wind Energy,
Vol. 25,
Issue. 8,
p.
1434.
Angelou, Nikolas
Mann, Jakob
and
Dubreuil-Boisclair, Camille
2023.
Revealing inflow and wake conditions of a 6 MW floating turbine.
Wind Energy Science,
Vol. 8,
Issue. 10,
p.
1511.
Raibaudo, Cédric
Gilloteaux, Jean-Christophe
and
Perret, Laurent
2023.
Realistic turbulent inflow conditions for estimating the performance of a floating wind turbine.
Wind Energy Science,
Vol. 8,
Issue. 11,
p.
1711.
Yang, Lin
Liao, Kangping
Ma, Qingwei
Ma, Gang
and
Sun, Hanbing
2023.
Investigation of wake characteristics of floating offshore wind turbine with control strategy using actuator curve embedding method.
Renewable Energy,
Vol. 218,
Issue. ,
p.
119255.
Zhang, Fengshun
Yang, Xiaolei
and
He, Guowei
2023.
Multiscale analysis of a very long wind turbine wake in an atmospheric boundary layer.
Physical Review Fluids,
Vol. 8,
Issue. 10,
Li, Yunliang
Li, Zhaobin
Zhou, Zhideng
and
Yang, Xiaolei
2023.
Large-Eddy Simulation of Wind Turbine Wakes in Forest Terrain.
Sustainability,
Vol. 15,
Issue. 6,
p.
5139.
Gambuzza, Stefano
and
Ganapathisubramani, Bharathram
2023.
The influence of free stream turbulence on the development of a wind turbine wake.
Journal of Fluid Mechanics,
Vol. 963,
Issue. ,
Wu, Chutian
Wang, Shizhao
Zhang, Xin-Lei
and
He, Guowei
2023.
Explainability analysis of neural network-based turbulence modeling for transonic axial compressor rotor flows.
Aerospace Science and Technology,
Vol. 141,
Issue. ,
p.
108542.
Bossuyt, Juliaan
Ferčák, Ondřej
Sadek, Zein
Meneveau, Charles
Gayme, Dennice F.
and
Cal, Raúl Bayoán
2023.
Floating wind farm experiments through scaling for wake characterization, power extraction, and turbine dynamics.
Physical Review Fluids,
Vol. 8,
Issue. 12,
Zhang, Zhihao
Kuang, Limin
Han, Zhaolong
Zhou, Dai
Zhao, Yongsheng
Bao, Yan
Duan, Lei
Tu, Jiahuang
Chen, Yaoran
and
Chen, Mingsheng
2023.
Comparative analysis of bent and basic winglets on performance improvement of horizontal axis wind turbines.
Energy,
Vol. 281,
Issue. ,
p.
128252.
Messmer, Thomas
Peinke, Joachim
and
Hölling, Michael
2024.
Wind tunnel investigation on the recovery and dynamics of the wake of a floating offshore wind turbine subjected to low inflow turbulence.
Journal of Physics: Conference Series,
Vol. 2767,
Issue. 9,
p.
092083.
Kang, Yujoo
and
Lee, Sang
2024.
Wake effect on floating offshore wind turbine fatigue load.
Physics of Fluids,
Vol. 36,
Issue. 12,
Schliffke, Benyamin
Conan, Boris
and
Aubrun, Sandrine
2024.
Floating wind turbine motion signature in the far-wake spectral content – a wind tunnel experiment.
Wind Energy Science,
Vol. 9,
Issue. 3,
p.
519.
Liu, Songyang
Xin, Zhiqiang
Wang, Lei
and
Cai, Zhiming
2024.
Fluid-structure interaction simulation of dynamic response and wake of floating offshore wind turbine considering tower shadow effect.
Acta Mechanica Sinica,
Vol. 40,
Issue. 8,
Du, Bowen
Ge, Mingwei
Li, Xintao
and
Liu, Yongqian
2024.
A momentum-conserving wake superposition method for wind-farm flows under pressure gradient.
Journal of Fluid Mechanics,
Vol. 999,
Issue. ,
Taruffi, Federico
Combette, Robin
and
Viré, Axelle
2024.
Experimental and CFD analysis of a floating offshore wind turbine under imposed motions.
Journal of Physics: Conference Series,
Vol. 2767,
Issue. 6,
p.
062010.
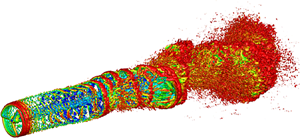
 $St = fD/U_\infty \in (0.2,0.6)$ (where
$St = fD/U_\infty \in (0.2,0.6)$ (where  $f$ is the motion frequency,
$f$ is the motion frequency,  $D$ is the rotor diameter, and
$D$ is the rotor diameter, and  $U_\infty$ is the incoming wind speed), which overlap with the Strouhal numbers of wake meandering induced by the shear layer instability, can lead to wake meandering with amplitudes being one order of magnitude larger than the FOWT motion for the most unstable frequency. For high inflow turbulence intensity, on the other hand, the onset of wake meandering is dominated by the inflow turbulence. The probability density function of the spanwise instantaneous wake centres is observed being non-Gaussian and closely related to that of the side-to-side motion. This complements the existing wake meandering mechanisms, that the side-to-side motion of an FOWT can be a novel origin for the onset of wake meandering. It is also found that LSA can predict the least stable frequencies and the amplification factor with acceptable accuracy for motion amplitude
$U_\infty$ is the incoming wind speed), which overlap with the Strouhal numbers of wake meandering induced by the shear layer instability, can lead to wake meandering with amplitudes being one order of magnitude larger than the FOWT motion for the most unstable frequency. For high inflow turbulence intensity, on the other hand, the onset of wake meandering is dominated by the inflow turbulence. The probability density function of the spanwise instantaneous wake centres is observed being non-Gaussian and closely related to that of the side-to-side motion. This complements the existing wake meandering mechanisms, that the side-to-side motion of an FOWT can be a novel origin for the onset of wake meandering. It is also found that LSA can predict the least stable frequencies and the amplification factor with acceptable accuracy for motion amplitude  $0.01D$. Effects of nonlinearity are observed when motion amplitude increases to
$0.01D$. Effects of nonlinearity are observed when motion amplitude increases to  $0.04D$, for which the most unstable turbine oscillations shift slightly to lower frequencies and the amplification factor decreases.
$0.04D$, for which the most unstable turbine oscillations shift slightly to lower frequencies and the amplification factor decreases.


