Hostname: page-component-586b7cd67f-2plfb Total loading time: 0 Render date: 2024-11-28T15:45:14.778Z Has data issue: false hasContentIssue false
  • English
  • Français

On the universality of anomalous scaling exponents of structure functions in turbulent flows

Published online by Cambridge University Press:  05 January 2018

E.-W. Saw Open the ORCID record for E.-W. Saw [Opens in a new window] ,
P. Debue ,
D. Kuzzay ,
F. Daviaud  and
B. Dubrulle
E.-W. Saw
Affiliation:
SPEC/IRAMIS/DSM, CEA, CNRS, University Paris-Saclay, CEA Saclay, 91191 Gif-sur-Yvette, France School of Atmospheric Sciences, Sun Yat-Sen University, Guangzhou, China
P. Debue
Affiliation:
SPEC/IRAMIS/DSM, CEA, CNRS, University Paris-Saclay, CEA Saclay, 91191 Gif-sur-Yvette, France
D. Kuzzay
Affiliation:
SPEC/IRAMIS/DSM, CEA, CNRS, University Paris-Saclay, CEA Saclay, 91191 Gif-sur-Yvette, France
F. Daviaud
Affiliation:
SPEC/IRAMIS/DSM, CEA, CNRS, University Paris-Saclay, CEA Saclay, 91191 Gif-sur-Yvette, France
B. Dubrulle*
Affiliation:
SPEC/IRAMIS/DSM, CEA, CNRS, University Paris-Saclay, CEA Saclay, 91191 Gif-sur-Yvette, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

All previous experiments in open turbulent flows (e.g. downstream of grids, jets and the atmospheric boundary layer) have produced quantitatively consistent values for the scaling exponents of velocity structure functions (Anselmet et al., J. Fluid Mech., vol. 140, 1984, pp. 63–89; Stolovitzky et al., Phys. Rev. E, vol. 48 (5), 1993, R3217; Arneodo et al., Europhys. Lett., vol. 34 (6), 1996, p. 411). 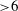The only measurement of scaling exponents at high order (${>}6$) in closed turbulent flow (von Kármán swirling flow) using Taylor’s frozen flow hypothesis, however, produced scaling exponents that are significantly smaller, suggesting that the universality of these exponents is broken with respect to change of large scale geometry of the flow. Here, we report measurements of longitudinal structure functions of velocity in a von Kármán set-up without the use of the Taylor hypothesis. The measurements are made using stereo particle image velocimetry at four different ranges of spatial scales, in order to observe a combined inertial subrange spanning approximately one and a half orders of magnitude. We found scaling exponents (up to ninth order) that are consistent with values from open turbulent flows, suggesting that they might be in fact universal.

JFM classification

Turbulent Flows: Intermittency Turbulent Flows: Turbulence theory Turbulent Flows: Turbulent Flows
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 837 , 25 February 2018 , pp. 657 - 669
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Anselmet, F., Gagne, Y., Hopfinger, E. J. & Antonia, R. A. 1984 High-order velocity structure functions in turbulent shear flows. J. Fluid Mech. 140, 63–89.Google Scholar
Arneodo, A. E., Baudet, C., Belin, F., Benzi, R., Castaing, B., Chabaud, B., Chavarria, R., Ciliberto, S., Camussi, R., Chilla, F. & Dubrulle, B. 1996 Structure functions in turbulence, in various flow configurations, at Reynolds number between 30 and 5000 using extended self-similarity. Europhys. Lett. 34 (6), 411–416.Google Scholar
Belin, F., Tabeling, P. & Willaime, H. 1996 Exponents of the structure functions in a low temperature helium experiment. Physica D 93 (1), 52–63.Google Scholar
Benzi, R., Ciliberto, S., Tripiccione, R., Baudet, C., Massaioli, F. & Succi, S. 1993 Extended self-similarity in turbulent flows. Phys. Rev. E 48 (1), R29.Google Scholar
Biferale, L. & Procaccia, I. 2005 Anisotropy in turbulent flows and in turbulent transport. Phys. Rep. 414.2, 43–164.Google Scholar
Dhruva, B., Tsuji, Y. & Sreenivasan, K. R. 1997 Transverse structure functions in high-Reynolds-number turbulence. Phys. Rev. E 56 (5), R4928.Google Scholar
Gotoh, T., Fukayama, D. & Nakano, T. 2002 Velocity field statistics in homogeneous steady turbulence obtained using a high-resolution direct numerical simulation. Phys. Fluids 14 (3), 1065–1081.Google Scholar
Huisman, S. G., Lohse, D. & Sun, C. 2013 Statistics of turbulent fluctuations in counter-rotating Taylor–Couette flows. Phys. Rev. E 88.6, 063001.Google Scholar
Ishihara, T., Gotoh, T. & Kaneda, Y. 2009 Study of high-Reynolds number isotropic turbulence by direct numerical simulation. Annu. Rev. Fluid Mech. 41, 165–180.Google Scholar
Iyer, K. P., Sreenivasan, K. R. & Yeung, P. K. 2017 Reynolds number scaling of velocity increments in isotropic turbulence. Phys. Rev. E 95 (2), 021101.Google Scholar
Kolmogorov, A. N. 1941a The local structure of turbulence in incompressible viscous fluid for very large Reynolds numbers. Dokl. Akad. Nauk SSSR 30 (4), 299–303.Google Scholar
Kolmogorov, A. N. 1941b Dissipation of energy in locally isotropic turbulence. Dokl. Akad. Nauk SSSR 32 (1), 16–18.Google Scholar
Kolmogorov, A. N. 1962 A refinement of previous hypotheses concerning the local structure of turbulence in a viscous incompressible fluid at high Reynolds number. J. Fluid Mech. 13 (1), 82–85.Google Scholar
Kuzzay, D., Faranda, D. & Dubrulle, B. 2015 Global vs local energy dissipation: the energy cycle of the turbulent von Kármán flow. Phys. Fluids 27, 075105.Google Scholar
Lewis, G. S. & Swinney, H. L. 1999 Velocity structure functions, scaling, and transitions in high-Reynolds-number Couette–Taylor flow. Phys. Rev. E 5, 5457.Google Scholar
Maurer, J., Tabeling, P. & Zocchi, G. 1994 Statistics of turbulence between two counterrotating disks in low-temperature helium gas. Europhys. Lett. 26 (1), 31–36.Google Scholar
Pinton, J.-F. & Labbé, R. 1994 Correction to the Taylor hypothesis in swirling flows. J. Phys. II France 4, 1461–1468.Google Scholar
Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.Google Scholar
Saw, E. W., Kuzzay, D., Faranda, D., Guittonneau, A., Daviaud, F., Wiertel-Gasquet, C. & Dubrulle, B. 2016 Experimental characterization of extreme events of inertial dissipation in a turbulent swirling flow. Nat. Commun. 7, 12466.Google Scholar
She, Z. S. & Leveque, E. 1994 Universal scaling laws in fully developed turbulence. Phys. Rev. Lett. 72 (3), 336.Google Scholar
Shen, X. & Warhaft, Z. 2002 Longitudinal and transverse structure functions in sheared and unsheared wind-tunnel turbulence. Phys. Fluids 14 (1), 370–381.Google Scholar
Sirovich, L., Smith, L. & Yakhot, V. 1994 Energy spectrum of homogeneous and isotropic turbulence in far dissipation range. Phys. Rev. Lett. 72 (3), 344–347.Google Scholar
Sreenivasan, K. R. & Antonia, R. A. 1997 The phenomenology of small-scale turbulence. Annu. Rev. Fluid Mech. 29.1, 435–472.Google Scholar
Stolovitzky, G., Sreenivasan, K. R. & Juneja, A. 1993 Scaling functions and scaling exponents in turbulence. Phys. Rev. E 48 (5), R3217.Google Scholar
Zocchi, G., Tabeling, P., Maurer, J. & Willaime, H. 1994 Measurement of the scaling of the dissipation at high Reynolds numbers. Phys. Rev. E 50 (5), 3693.Google Scholar