Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Du, Shanxiao
Hong, Jichao
Zhang, Hongxin
Zhao, Qinghai
Zhang, Tiezhu
Xu, Xiaoming
and
Jiang, Xiaotian
2021.
Research on Cavitation of the Rotating-Sleeve Distributing Flow System Considering Different Cam Groove Profiles.
Energies,
Vol. 14,
Issue. 8,
p.
2139.
Liao, Yingqian
Martins, Joaquim R.R.A.
and
Young, Yin Lu
2021.
3-D high-fidelity hydrostructural optimization of cavitation-free composite lifting surfaces.
Composite Structures,
Vol. 268,
Issue. ,
p.
113937.
Ge, Muye
Svennberg, Urban
and
Bensow, Rickard E.
2021.
Improved Prediction of Sheet Cavitation Inception Using Bridged Transition Sensitive Turbulence Model and Cavitation Model.
Journal of Marine Science and Engineering,
Vol. 9,
Issue. 12,
p.
1343.
Chen, Jie
Hu, Changli
Zhang, Mengjie
Huang, Biao
and
Zhang, Hanzhe
2021.
The influence of micro vortex generator on inception cavitation.
Physics of Fluids,
Vol. 33,
Issue. 10,
Wang, Ziyang
Cheng, Huaiyu
and
Ji, Bin
2021.
Euler–Lagrange study of cavitating turbulent flow around a hydrofoil.
Physics of Fluids,
Vol. 33,
Issue. 11,
Chen, Jie
Hu, Changli
Zhang, Mengjie
Huang, Biao
and
Zhang, Hanzhe
2022.
Experimental investigation into passive control effect of micro vortex generator at various cavitation conditions.
Ocean Engineering,
Vol. 260,
Issue. ,
p.
111734.
Rokhsar Talabazar, Farzad
Sheibani Aghdam, Araz
Jafarpour, Mohammad
Grishenkov, Dmitry
Koşar, Ali
and
Ghorbani, Morteza
2022.
Chemical effects in “hydrodynamic cavitation on a chip”: The role of cavitating flow patterns.
Chemical Engineering Journal,
Vol. 445,
Issue. ,
p.
136734.
Gluzman, Igal
and
Thomas, Flint O.
2022.
Characterization of bubble dynamics in the nozzle flow of aviation fuels via computer vision tools.
International Journal of Multiphase Flow,
Vol. 154,
Issue. ,
p.
104133.
Brandner, Paul A.
Venning, James A.
and
Pearce, Bryce W.
2022.
Nucleation effects on cavitation about a sphere.
Journal of Fluid Mechanics,
Vol. 946,
Issue. ,
Li, Zehao
Zuo, Zhigang
and
Qian, Zhongdong
2022.
A Venturi tube design for studying travelling bubble cavitation.
Journal of Physics: Conference Series,
Vol. 2217,
Issue. 1,
p.
012023.
Agarwal, Karuna
Ram, Omri
Lu, Yuhui
and
Katz, Joseph
2023.
On the pressure field, nuclei dynamics and their relation to cavitation inception in a turbulent shear layer.
Journal of Fluid Mechanics,
Vol. 966,
Issue. ,
Wang, Ziyang
Cheng, Huaiyu
Bensow, Rickard E.
Peng, Xiaoxing
and
Ji, Bin
2023.
Numerical assessment of cavitation erosion risk on the Delft twisted hydrofoil using a hybrid Eulerian-Lagrangian strategy.
International Journal of Mechanical Sciences,
Vol. 259,
Issue. ,
p.
108618.
Li, Zehao
Zuo, Zhigang
and
Qian, Zhongdong
2023.
Diffusion-driven periodic cavitation bubbling from a Harvey-type crevice in shear flows.
Physics of Fluids,
Vol. 35,
Issue. 10,
Rokhsar Talabazar, Farzad
Maleki, Mohammadamin
Sheibani Aghdam, Araz
Grishenkov, Dmitry
Ghorbani, Morteza
and
Koşar, Ali
2023.
Cavitation inception and evolution in cavitation on a chip devices at low upstream pressures.
Physics of Fluids,
Vol. 35,
Issue. 1,
Chen, Jie
Guo, Dongwei
Zhang, Jiakun
Wang, Yong
Liu, Taotao
Wu, Xian
and
Hu, Changli
2024.
Combined experimental and computational investigation of the influence of micro vortex generator on incipient cavitation mode.
Physics of Fluids,
Vol. 36,
Issue. 12,
Decaix, Jean
2024.
New proposals for coupling cavitation models with laminar/turbulent transition models: application to a cavitation sheet over a NACA 16 012.
Journal of Hydrodynamics,
Vol. 36,
Issue. 4,
p.
725.
Ge, Mingming
Apte, Dhruv
Wang, Chuan
Zhang, Guangjian
Zhang, Xinlei
and
Coutier-Delgosha, Olivier
2024.
Slip velocity and field information of two-phase cavitating flows.
Physics of Fluids,
Vol. 36,
Issue. 9,
Feng, Xianren
Liu, Yunqiao
and
Wang, Benlong
2024.
An improved laminar–turbulent transition cavitation model using the IDDES method.
Ocean Engineering,
Vol. 297,
Issue. ,
p.
117182.
Qin, Xinzhen
Chen, Yihong
Feng, Xianren
Shao, Xueming
and
Deng, Jian
2025.
A numerical investigation concerning wall-nucleation effects of the inception of sheet cavitation.
International Journal of Multiphase Flow,
Vol. 185,
Issue. ,
p.
105142.
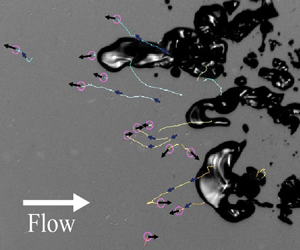
 $(50\text {--}60\ \mathrm {\mu }\textrm {m})$ low-momentum zones form close to the wall. Microbubbles trapped in these regions are generated initially from the collapse of intermittent attachment of travelling bubble cavitation. These bubbles migrate slowly upstream for a few milliseconds either under the influence of the adverse pressure gradients when the flow remains attached or carried by the recirculating flow when the boundary layer is separated. Their speed is only 2 %–4 % of the free-stream velocity, and their trajectories are erratic, indicating near-dynamic equilibrium. Owing to the low local pressure, their diameter increases by two to four times by non-condensable gas diffusion, from
$(50\text {--}60\ \mathrm {\mu }\textrm {m})$ low-momentum zones form close to the wall. Microbubbles trapped in these regions are generated initially from the collapse of intermittent attachment of travelling bubble cavitation. These bubbles migrate slowly upstream for a few milliseconds either under the influence of the adverse pressure gradients when the flow remains attached or carried by the recirculating flow when the boundary layer is separated. Their speed is only 2 %–4 % of the free-stream velocity, and their trajectories are erratic, indicating near-dynamic equilibrium. Owing to the low local pressure, their diameter increases by two to four times by non-condensable gas diffusion, from  $10$ to
$10$ to  $30\ \mathrm {\mu }\textrm {m}$ to the thickness of the low-momentum zone. At that time, either they are swept downstream by the free-stream flow or they become nuclei for new attached cavitation events. When the new patches collapse, new microbubbles form and the process repeats itself frequently, and independently of the free-stream nuclei. These phenomena do not occur when the adverse pressure gradients are too mild to create low-momentum zones with sufficient thickness to facilitate the slow upstream migration and growth.
$30\ \mathrm {\mu }\textrm {m}$ to the thickness of the low-momentum zone. At that time, either they are swept downstream by the free-stream flow or they become nuclei for new attached cavitation events. When the new patches collapse, new microbubbles form and the process repeats itself frequently, and independently of the free-stream nuclei. These phenomena do not occur when the adverse pressure gradients are too mild to create low-momentum zones with sufficient thickness to facilitate the slow upstream migration and growth.