Hostname: page-component-586b7cd67f-dlnhk Total loading time: 0 Render date: 2024-11-25T14:42:20.775Z Has data issue: false hasContentIssue false
  • English
  • Français

On Knudsen-minimum effect and temperature bimodality in a dilute granular Poiseuille flow

Published online by Cambridge University Press:  06 October 2015

Meheboob Alam ,
Achal Mahajan  and
Deepthi Shivanna
Meheboob Alam*
Affiliation:
Engineering Mechanics Unit, Jawaharlal Nehru Center for Advanced Scientific Research, Jakkur P.O., Bangalore 560064, India
Achal Mahajan
Affiliation:
Engineering Mechanics Unit, Jawaharlal Nehru Center for Advanced Scientific Research, Jakkur P.O., Bangalore 560064, India
Deepthi Shivanna
Affiliation:
Engineering Mechanics Unit, Jawaharlal Nehru Center for Advanced Scientific Research, Jakkur P.O., Bangalore 560064, India
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The numerical simulation of gravity-driven flow of smooth inelastic hard disks through a channel, dubbed ‘granular’ Poiseuille flow, is conducted using event-driven techniques. We find that the variation of the mass-flow rate (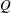$Q$) with Knudsen number (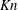$Kn$) can be non-monotonic in the elastic limit (i.e. the restitution coefficient 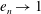$e_{n}\rightarrow 1$) in channels with very smooth walls. The Knudsen-minimum effect (i.e. the minimum flow rate occurring at 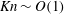$Kn\sim O(1)$ for the Poiseuille flow of a molecular gas) is found to be absent in a granular gas with 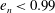$e_{n}<0.99$, irrespective of the value of the wall roughness. Another rarefaction phenomenon, the bimodality of the temperature profile, with a local minimum ($T_{\mathit{min}}$) at the channel centerline and two symmetric maxima (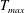$T_{\mathit{max}}$) away from the centerline, is also studied. We show that the inelastic dissipation is responsible for the onset of temperature bimodality (i.e. the ‘excess’ temperature, 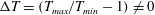${\rm\Delta}T=(T_{\mathit{max}}/T_{\mathit{min}}-1)\neq 0$) near the continuum limit ($Kn\sim 0$), but the rarefaction being its origin (as in the molecular gas) holds beyond 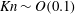$Kn\sim O(0.1)$. The dependence of the excess temperature ${\rm\Delta}T$ on the restitution coefficient is compared with the predictions of a kinetic model, with reasonable agreement in the appropriate limit. The competition between dissipation and rarefaction seems to be responsible for the observed dependence of both the mass-flow rate and the temperature bimodality on 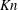$Kn$ and 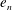$e_{n}$ in this flow. The validity of the Navier–Stokes-order hydrodynamics for granular Poiseuille flow is discussed with reference to the prediction of bimodal temperature profiles and related surrogates.

JFM classification

Granular media: Granular media Rarefied Gas Flow: Molecular dynamics Rarefied Gas Flow: Rarefied Gas Flow
Type
Papers
Information
Journal of Fluid Mechanics , Volume 782 , 10 November 2015 , pp. 99 - 126
Check for updates
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alam, M. & Chikkadi, V. 2010 Velocity distribution function and correlations in a granular Poiseuille flow. J. Fluid Mech. 653, 175–219.Google Scholar
Alam, M., Chikkadi, V. & Gupta, V. K. 2009 Density waves and the effect of wall roughness in granular Poiseuille flow: simulation and linear stability. Eur. Phys. J. Special Topics 179, 69–90.Google Scholar
Alam, M. & Luding, S. 2003 First normal stress difference and crystallization in a dense sheared granular fluid. Phys. Fluids 15, 2298–2312.Google Scholar
Alam, M. & Luding, S. 2005a Energy nonequipartition, rheology and microstructure in sheared bidisperse granular mixtures. Phys. Fluids 17, 063303.Google Scholar
Alam, M. & Luding, S. 2005b Non-Newtonian granular fluid: simulation and theory. In Powders and Grains (ed. Garcia-Rojo, R., Herrmann, H. J. & McNamara, S.), pp. 1141–1144. A. A. Balkema.Google Scholar
Aoki, K., Takata, S. & Nakanishi, T. 2002 Poiseuille-type flow of a rarefied gas between two parallel plates driven by a uniform external force. Phys. Rev. E 65, 026315.Google Scholar
Bird, G. A. 1994 Molecular Gas Dynamics and the Direct Simulation of Gas Flows. Oxford University Press.Google Scholar
Burnett, D. 1935 The distribution of velocities in a slightly non-uniform gas. Proc. Lond. Math. Soc. 39, 385–430.Google Scholar
Chapman, S. & Cowling, T. G. 1970 The Mathematical Theory for Non-uniform Gases. Cambridge University Press.Google Scholar
Cercignani, C. 2000 Rarefied Gas Dynamics. Cambridge University Press.Google Scholar
Cercignani, C. & Daneri, A. 1963 Flow of a rarefied gas between two parallel plates. J. Appl. Phys. 34, 3509–3513.Google Scholar
Chikkadi, V. & Alam, M. 2009 Slip velocity and stresses in granular Poiseuille flow via event driven simulation. Phys. Rev. E 80, 021303.Google Scholar
Forterre, Y. & Pouliquen, O. 2008 Flows of dense granular media. Annu. Rev. Fluid Mech. 40, 1–24.Google Scholar
Galvin, J., Hrenya, C. & Wildman, R. 2007 On the role of Knudsen layer in rapid granular flows. J. Fluid Mech. 585, 73–92.Google Scholar
Gayen, B. & Alam, M. 2008 Orientation correlation and velocity distributions in uniform shear flow of a dilute granular gas. Phys. Rev. Lett. 100, 068002.Google Scholar
Goldhirsch, I. 2003 Rapid granular flows. Annu. Rev. Fluid Mech. 35, 267–293.Google Scholar
Grad, H. 1949 On the kinetic theory of rarefied gases. Commun. Pure Appl. Maths. 2, 331–407.Google Scholar
Khain, E., Meerson, B. & Sasorov, P. V. 2008 Knudsen temperature jump and the Navier–Stokes hydrodynamics of granular gases driven by thermal wall. Phys. Rev. E 78, 041303.Google Scholar
Knudsen, M. 1909 Die Gesetze der Molekularstromung und der inneren Reibungsstromung der Gase durch Rohren. Ann. Phys. 28, 75–130.CrossRefGoogle Scholar
Kogan, M. N. 1969 Rarefied Gas Dynamics. Plenum.Google Scholar
Liss, E., Conway, S. L. & Glasser, B. J. 2002 Density waves in gravity-driven granular flow through a channel. Phys. Fluids 14, 3309–3328.Google Scholar
Lubachevsky, B. 1991 How to simulate billiards and similar systems. J. Comput. Phys. 94, 255–283.Google Scholar
Luding, S. & McNamara, S. 1998 How to handle the inelastic collapse of a dissipative hard-sphere gas with the TC model. Granul. Matt. 1, 113–128.Google Scholar
Mansour, M., Baras, F. & Garcia, A. L. 1997 On the validity of hydrodynamics in plane Poiseuille flows. Physica A 240, 255–267.Google Scholar
Pöschel, T. & Luding, S. 2001 Granular Gases. Springer.Google Scholar
Rao, K. K. & Nott, P. R. 2008 Introduction to Granular Flows. Cambridge University Press.Google Scholar
Rongali, R. & Alam, M. 2014 Higher-order effects on orientational correlation and relaxation dynamics in homogeneous cooling of a rough granular gas. Phys. Rev. E 89, 062201.Google Scholar
Rongali, R. & Alam, M.2015 Bulk hydrodynamics of Poiseuille flow of a heated granular gas. Preprint.Google Scholar
Saha, S. & Alam, M. 2014 Non-Newtonian stress, collisional dissipation and heat flux in the shear flow of inelastic disks: a reduction via Grad’s moment method. J. Fluid Mech. 757, 251–296.Google Scholar
Sela, N. & Goldhirsch, I. 1998 Hydrodynamic equations for rapid shear flows of smooth, inelastic spheres, to Burnett order. J. Fluid Mech. 361, 41–68.Google Scholar
Tij, M. & Santos, A. 1994 Perturbation analysis of a stationary non-equilibrium flow generated by external force. J. Stat. Phys. 76, 1399–1414.Google Scholar
Tij, M. & Santos, A. 2004 Poiseuille flow in a heated granular gas. J. Stat. Phys. 117, 901–928.Google Scholar
Torrilhon, M. & Struchtrup, H. 2004 Regularized 13-moment equations: shock structure calculations and comparison to Burnett models. J. Fluid Mech. 513, 171–198.Google Scholar
Vega Reyes, F., Santos, A. & Garzo, V. 2010 Non-Newtonian granular hydrodynamics. What do the inelastic simple shear flow and the elastic Fourier flow have in common? Phys. Rev. Lett. 104, 028001.Google Scholar
Vijayakumar, K. C. & Alam, M. 2007 Velocity distribution and the effect of wall roughness in granular Poiseuille flow. Phys. Rev. E 75, 051306.Google Scholar