Hostname: page-component-586b7cd67f-r5fsc Total loading time: 0 Render date: 2024-11-28T11:04:33.519Z Has data issue: false hasContentIssue false
  • English
  • Français

Numerical investigation on body-wake flow interaction over rod–airfoil configuration

Published online by Cambridge University Press:  14 August 2015

Yi Jiang ,
Mei-Liang Mao ,
Xiao-Gang Deng  and
Hua-Yong Liu
Yi Jiang*
Affiliation:
State Key Laboratory of Aerodynamics, China Aerodynamics Research and Development Center, PO Box 211, Mianyang 621000, PR China Computational Aerodynamics Institute, China Aerodynamics Research and Development Center, PO Box 211, Mianyang 621000, PR China
Mei-Liang Mao
Affiliation:
State Key Laboratory of Aerodynamics, China Aerodynamics Research and Development Center, PO Box 211, Mianyang 621000, PR China Computational Aerodynamics Institute, China Aerodynamics Research and Development Center, PO Box 211, Mianyang 621000, PR China
Xiao-Gang Deng
Affiliation:
National University of Defense Technology, Changsha, Hunan 410073, PR China
Hua-Yong Liu
Affiliation:
State Key Laboratory of Aerodynamics, China Aerodynamics Research and Development Center, PO Box 211, Mianyang 621000, PR China
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Numerical investigations of body-wake interactions were carried out by simulating the flow over a rod–airfoil configuration using high-order implicit large eddy simulation (HILES) for the incoming velocity 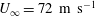$U_{\infty }=72~\text{m}~\text{s}^{-1}$ and a Reynolds number based on the airfoil chord 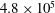$4.8\times 10^{5}$. The flow over five different rod–airfoil configurations with different distances of $L/d=2$, 4, 6, 8 and 10, respectively, were calculated for the analysis of body-wake interaction phenomena. Various fundamental mechanisms dictating the intricate flow phenomena including force varying regulation, flow structures and flow patterns in the interaction region, turbulent fluctuations and their suppression, noise radiation and fluid resonant oscillation, have been studied systematically. Due to the airfoil downstream, a relatively higher base pressure is exerted on the surface of the cylinder upstream, and the pressure fluctuation on the surface of the rod–airfoil configuration with $L/d=2$ is significantly suppressed, resulting in a reduction of the fluctuating lift. Following the distance between the cylinder and airfoil strongly decreases, Kármán-street shedding is suppressed due to the blocking effect. The flow in this interaction region has two opposite tendencies: the influence of the airfoil on the steady flow is to accelerate it and the counter-rotating vortices connecting with the leading edge of the airfoil tend to slow the flow down. There may be two flow patterns associated with the interference region, i.e. the Kármán-street suppressing mode and the Kármán-street shedding mode. The primary vortex shedding behind the cylinder upstream, and the shedding wake impingement onto the airfoil downstream, play a dominant role in the production of turbulent fluctuations. When primary vortex shedding is suppressed, the intensity of impingement is weakened, resulting in a significant suppression of the turbulent fluctuations. Due to these factors, a special broadband noise without a manifestly distinguishable peak is radiated by the rod–airfoil configuration with $L/d=2$. The fluid resonant oscillation within the flow interaction between the turbulent wake and the bodies was further investigated by adopting a feedback model, which confirmed that the effect of fluid resonant oscillation becomes stronger when 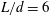$L/d=6$ and 10. The results obtained in this study provide physical insight into the understanding of the mechanisms relevant to the body-wake interaction.

JFM classification

Acoustics: Aeroacoustics Aerodynamics: Flow-structure interactions Turbulent Flows: Turbulence simulation
Type
Papers
Information
Journal of Fluid Mechanics , Volume 779 , 25 September 2015 , pp. 1 - 35
Check for updates
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Achenbach, E. 1968 Distribution of local pressure and skin friction around a circular cylinder in cross-flow up to $\mathit{Re}=5\times 10^{6}$ . J. Fluid Mech. 34, 625–639.Google Scholar
Agrawal, B. R. & Sharma, A.2014 Aerodynamic noise prediction for a rod–airfoil configuration using large eddy simulations. AIAA Paper 2014-3295.CrossRefGoogle Scholar
Apelt, C. J. & West, G. S. 1975 The effects of wake splitter plates on bluff-body flow in the range $10^{4}<\mathit{Re}<5\times 10^{4}$ . Part 2. J. Fluid Mech. 71, 145–160.CrossRefGoogle Scholar
Boris, J. P., Grinstein, F. F., Oran, E. S. & Kolbe, R. L. 1992 New insights into large eddy simulation. Fluid Dyn. Res. 10, 199–228.CrossRefGoogle Scholar
Boudet, J., Grosjean, N. & Jacob, M. C. 2005 Wake-airfoil interaction as broadband noise source: a large-eddy simulation study. Intl J. Aeroacoust. 4 (1), 93–116.CrossRefGoogle Scholar
Caraeni, M., Dai, Y. & Caraeni, D.2007 Acoustic investigation of rod airfoil configuration with DES and FWH. AIAA Paper 2007-4016.CrossRefGoogle Scholar
Carazo, A., Roger, M. & Omais, M.2011 Analytical prediction of wake-interaction noise in counter-rotating open rotors. AIAA Paper 2011-2758.Google Scholar
Casalino, D., Jacob, M. C. & Roger, M. 2003 Prediction of rod airfoil interaction noise using the FWH analogy. AIAA J. 41 (2), 182–191.Google Scholar
Creschner, B., Thiele, F., Casalino, D. & Jacob, M. C.2004 Influence of turbulence modelling on the broadband noise simulation for complex flows. AIAA Paper 2004-2926.Google Scholar
Daniel, J. B. 2006 Analysis of sponge zones for computational fluid mechanics. J. Comput. Phys. 212, 681–702.Google Scholar
Daude, F., Berland, J., Emmert, T., Lafon, P., Crouzet, F. & Bailly, C. 2012 A high-order finite-difference algorithm for direct computation of aerodynamic sound. Comput. Fluids 61, 46–63.CrossRefGoogle Scholar
Deng, X. G., Jiang, Y., Mao, M. L., Liu, H. Y., Li, S. & Tu, G. H. 2015 A family of hybrid cell-edge and cell-node dissipative compact schemes satisfying geometric conservation law. Comput. Fluids 116, 29–45.Google Scholar
Deng, X. G., Jiang, Y., Mao, M. L., Liu, H. Y. & Tu, G. H. 2013b Developing hybrid cell-edge and cell-node dissipative compact scheme for complex geometry flows. Sci. China Technol. Sci. 56, 2361–2369.CrossRefGoogle Scholar
Deng, X. G., Maekawa, H. & Shen, Q.1996 A class of high-order dissipative compact schemes. AIAA Paper 1996-1972.CrossRefGoogle Scholar
Deng, X. G., Mao, M. L., Tu, G. H., Liu, H. Y. & Zhang, H. X. 2011 Geometric conservation law and applications to high-order finite difference schemes with stationary grids. J. Comput. Phys. 230, 1100–1115.CrossRefGoogle Scholar
Deng, X. G., Min, Y. B., Mao, M. L., Liu, H. Y., Tu, G. H. & Zhang, H. X. 2013a Further studies on geometric conservation law and applications to high-order finite difference schemes with stationary grids. J. Comput. Phys. 239, 90–111.Google Scholar
Drikakis, D., Hahn, M., Mosedale, A. & Thornber, B. 2009 Large eddy simulation using high-resolution and high-order methods. Phil. Trans. R. Soc. Lond. A 367, 2985–2997.Google Scholar
Fitzpatrick, J. A. 2003 Flow/acoustic interactions of two cylinders in cross-flow. J. Fluids Struct. 17, 97–113.CrossRefGoogle Scholar
Gallardo, J. P., Andersson, H. I. & Pettersen, B. 2014 Turbulent wake behind a curved circular cylinder. J. Fluid Mech. 742, 192–229.Google Scholar
Gerolymos, G. A. & Vallet, I.2007 Influence of temporal integration and spatial discretization on hybrid RSM-VLES computations. AIAA Paper 2007-4094.Google Scholar
Gerrard, J. H. 1961 An experimental investigation of the oscillating lift and drag of a circular cylinder shedding turbulent vortices. J. Fluid Mech. 11, 244–256.Google Scholar
Giret, J. C., Sengissen, A., Moreau, S., Sanjosé, M. & Jouhaud, J. C. 2015 Noise source analysis of a rod–airfoil configuration using unstructured large eddy simulation. AIAA J. 53 (4), 1062–1077.Google Scholar
Gordnier, R. E. & Visbal, M. R.1993 Numerical simulation of delta-wing roll. AIAA Paper 1993-0554.Google Scholar
Greschner, B., Thiele, F., Jacob, M. C. & Casalino, D. 2008 Prediction of sound generated by a rod–airfoil configuration using EASM DES and the generalised Lighthill/FW-H analogy. Comput. Fluids 37, 402–413.CrossRefGoogle Scholar
Hahn, M., Drikakis, D., Youngs, D. L. & Williams, R. J. R. 2011 Richtmyer–Meshkov turbulent mixing arising from an inclined material interface with realistic surface perturbations and reshocked flow. Phys. Fluids 23, 046101.CrossRefGoogle Scholar
Hutcheson, F. V. & Brooks, T. F. 2012 Noise radiation from single and multiple rod configurations. Intl J. Aeroacoust. 11, 291–334.Google Scholar
Jacob, M. C., Boudet, J., Casalino, D. & Michard, M. 2005 A rod–airfoil experiment as benchmark for broadband noise modeling. J. Theor. Comput. Fluid Dyn. 19 (3), 171–196.Google Scholar
Jeong, J. & Hussain, F. 1995 On the identification of a vortex. J. Fluid Mech. 285, 69–94.Google Scholar
Jiang, M., Li, X. D. & Zhou, J. J. 2011 Experimental and numerical investigation on sound generation from airfoil-flow interaction. Appl. Math. Mech. 32 (6), 765–776.Google Scholar
Jiang, Y., Mao, M. L., Deng, X. G. & Liu, H. Y. 2013 Effect of surface conservation law on large eddy simulation based on seventh-order dissipative compact scheme. Appl. Mech. Mater. 419, 30–37.Google Scholar
Jiang, Y., Mao, M. L., Deng, X. G. & Liu, H. Y. 2014a Large eddy simulation on curvilinear meshes using seventh-order dissipative compact scheme. Comput. Fluids 104, 73–84.Google Scholar
Jiang, Y., Mao, M. L., Deng, X. G. & Liu, H. Y. 2015 Extending seventh-order dissipative compact scheme satisfying geometric conservation law to large eddy simulation on curvilinear grids. Adv. Appl. Maths Mech. 7 (4), 407–429.CrossRefGoogle Scholar
Jiang, Y., Mao, M. L., Deng, X. G., Liu, H. Y. & Yan, Zh. G. 2014b Numerical prediction of jet noise from nozzle using seventh-order dissipative compact scheme satisfying geometric conservation law. Appl. Mech. Mater. 574, 259–270.Google Scholar
Jiang, G. & Shu, C. 1996 Efficient implementation of weighted ENO. J. Comput. Phys. 181, 202–228.Google Scholar
John, M. H. & Jameson, A.2002 An implicit–explicit hybrid scheme for calculating complex unsteady flows. AIAA Paper 2002-0714.Google Scholar
King, W. F. N. & Pfizenmaier, E. 2009 An experimental study of sound generated by flows around cylinders of different cross-section. J. Sound Vib. 328, 318–337.CrossRefGoogle Scholar
Lele, S. K. 1992 Compact finite difference schemes with spectral-like resolution. J. Comput. Phys. 103, 16–42.CrossRefGoogle Scholar
Li, Y., Wang, X. N., Chen, Zh. W. & Li, Zh. Ch. 2014 Experimental study of vortex-structure interaction noise radiated from rod–airfoil configurations. J. Fluids Struct. 51, 313–325.CrossRefGoogle Scholar
Ljungkrona, L., Norberg, Ch. & Sunden, B. 1991 Free-stream turbulence and tube spacing effects on surface pressure fluctuations for two tubes in an in-line arrangement. J. Fluids Struct. 5, 701–727.Google Scholar
Lyrintzis, A. S. 2003 Surface integral methods in computational aeroacoustics – from the (CFD) near-field to the (acoustic) far-field. Intl J. Aeroacoust. 2 (2), 95–128.Google Scholar
Magagnato, F., Sorgüven, E. & Gabi, M.2003 Far field noise prediction by large eddy simulation and Ffowcs-Williams Hawkings analogy. AIAA Paper 2003-3206.Google Scholar
Mahir, N. & Rockwell, D. 1996 Vortex shedding from a forced system of two cylinders. Part I: tandem arrangement. J. Fluids Struct. 9, 473–489.Google Scholar
Mansy, H., Yang, P. M. & Williams, D. R. 1994 Quantitative measurements of three-dimensional structures in the wake of a circular cylinder. J. Fluid Mech. 270, 277–296.Google Scholar
Mao, M. L., Jiang, Y., Deng, X. G. & Liu, H. Y. 2016 Noise prediction in subsonic flow using seventh-order dissipative compact scheme on curvilinear mesh. Adv. Appl. Maths Mech. doi:10.4208/aamm.2014.m459.Google Scholar
Mochizuki, M., Kiya, M., Suzuki, T. & Arai, T. 1994 Vortex-shedding sound generated by two circular cylinders arranged in tandem. Trans. JSME B 60 (578), 3223–3229.Google Scholar
Munekata, M., Kawahara, K., Udo, T., Yoshikawa, H. & Ohba, H. 2006 An experimental study on aerodynamic sound generated from wake interference of circular cylinder and airfoil vane in tandem. J. Therm. Sci. 15 (4), 342–348.CrossRefGoogle Scholar
Munekata, M., Koshiishi, R., Yoshikawa, H. & Ohba, H. 2008 An experimental study on aerodynamic sound generated from wake interaction of circular cylinder and airfoil with attack angle in tandem. J. Therm. Sci. 17 (3), 212–217.Google Scholar
Oertel, H. & Affiliation, J. 1990 Wakes behind blunt bodies. Annu. Rev. Fluid Mech. 22, 539–564.Google Scholar
Owen, J. C. & Bearman, P. W. 2001 Passive control of VIV with drag reduction. J. Fluids Struct. 15, 597–605.Google Scholar
Pirozzoli, S., Grasso, F. & Gatski, T. B. 2004 Direct numerical simulation and analysis of a spatially evolving supersonic turbulent boundary layer at $M=2.25$ . Phys. Fluids 16, 530–545.Google Scholar
Poinsot, T. & Lele, S. K. 1992 Boundary conditions for direct simulations of compressible viscous flows. J. Comput. Phys. 101, 104–129.Google Scholar
Rizzetta, D. P., Visbal, M. R. & Morgan, P. E. 2008 A high-order compact finite-difference scheme for large-eddy simulation of active flow control. Prog. Aerosp. Sci. 44, 397–426.Google Scholar
Roger, M. & Carazo, A.2010 Blade-geometry considerations in analytical gust-airfoil interaction noise models. AIAA Paper 2010-3799.Google Scholar
Schell, A.2013 Validation of a direct noise calculation and a hybrid computational aeroacoustics approach in the acoustic far field of a rod–airfoil configuration. AIAA Paper 2013-2122.Google Scholar
Schlinker, R. H., Fink, M. R. & Amiet, R. K.1976 Vortex noise from non-rotating cylinders and airfoils. AIAA Paper 1976-81.Google Scholar
Szepessy, S. & Bearman, P. W. 1992 Aspect ratio and end plate effects on vortex shedding from a circular cylinder. J. Fluid Mech. 234, 191–217.Google Scholar
Tam, C. K. W. & Webb, J. C. 1993 Dispersion-relation-preserving finite difference schemes for computational acoustics. J. Comput. Phys. 107, 262–281.Google Scholar
Thornber, B. & Drikakis, D. 2008 Implicit large eddy simulation of a deep cavity using high-resolution methods. AIAA J. 46, 2634–2645.Google Scholar
Van Leer, B. 1977 Towards the ultimate conservative difference scheme. IV. A new approach to numerical convection. J. Comput. Phys. 23, 276–299.Google Scholar
Visbal, M. R. & Gaitonde, D. V. 2002 On the use of higher-order finite-difference schemes on curvilinear and deforming meshes. J. Comput. Phys. 181, 155–185.Google Scholar
Visbal, M. R. & Rizzetta, D. P. 2002 Large-eddy simulation on curvilinear grids using compact differencing and filtering schemes. Trans. ASME J. Fluids Engng 124, 836–847.Google Scholar
Wu, J. Z., Lu, X. Y. & Zhuang, L. X. 2007 Integral force acting on a body due to local flow structures. J. Fluid Mech. 576, 265–286.Google Scholar
Xu, C. Y., Chen, L. W. & Lu, X. Y. 2010 Large-eddy simulation of the compressible flow past a wavy cylinder. J. Fluid Mech. 665, 238–273.Google Scholar
Zdravkovich, M. M. 1977 Review of flow interference between two circular cylinders in various arrangements. Trans. ASME J. Fluids Engng 99, 618–633.Google Scholar
Zdravkovich, M. M. 1997 Flow Around Circular Cylinder, Vol. 1 Fundamentals. Oxford University Press.Google Scholar
Zdravkovich, M. M. 2003 Flow Around Circular Cylinder, Vol. 2 Fundamentals. Oxford University Press.Google Scholar