Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-20T16:55:35.544Z Has data issue: false hasContentIssue false
  • English
  • Français

Numerical investigation of the generation and growth of coherent flow structures in a triggered turbulent spot

Published online by Cambridge University Press:  23 October 2014

Joshua R. Brinkerhoff  and
Metin I. Yaras
Joshua R. Brinkerhoff
Affiliation:
Department of Mechanical and Aerospace Engineering, Carleton University, Ottawa, ON K1S 5B6, Canada
Metin I. Yaras*
Affiliation:
Department of Mechanical and Aerospace Engineering, Carleton University, Ottawa, ON K1S 5B6, Canada
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Multiple mechanisms for the regeneration of hairpin-like coherent flow structures in transitional and turbulent boundary layers have been proposed in the published literature, but a complete understanding of the typical topologies of coherent structures observed in the literature has not yet been achieved. To contribute to this understanding, a numerical study is performed of a turbulent spot triggered in a zero-pressure-gradient laminar boundary layer by a pulsed, transverse jet. Two direct numerical simulations (DNS) capture the growth of the spot into a mature turbulent region containing a large number of coherent vortical flow structures. The boundary-layer Reynolds number based on the test-surface streamwise length is 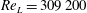$\mathit{Re}_{L}=309\,200$. The internal structure of the spot is characterized by densely spaced packets of hairpin vortices. Lateral growth of the spot occurs as new hairpin vortices form along the spanwise edges of the spot. The formation of these hairpin vortices is attributed to unstable shear layers that develop in the streamwise–spanwise plane due to the wall-normal motions induced by the streamwise oriented legs of hairpin vortices within the spot. Results are presented that highlight the mechanism by which the instability of such shear layers forms wavepackets of hairpin vortices; how the formation of these vortices produces a flow environment that promotes the creation of new hairpin vortices; and how the newly created hairpin vortices impact the production of turbulence kinetic energy in the flow region surrounding the spot. A quantitative description of the hairpin-vortex regeneration mechanism based on the transport of the instantaneous vorticity vector is presented to illustrate how the velocity and vorticity fields interact with the local strain rates to promote the growth of coherent vortical structures. The simulation results also shed light on a mechanism that seems to have a dominant influence on the formation of the calmed region in the wake of the turbulent spot.

JFM classification

Instability: Transition to turbulence Turbulent Flows: Turbulence simulation Turbulent Flows: Turbulent Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 759 , 25 November 2014 , pp. 257 - 294
Check for updates
Copyright
© 2014 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Adrian, R. J., Meinhart, C. D. & Tomkins, C. D. 2000 Vortex organization in the outer region of the turbulent boundary layer. J. Fluid Mech. 422, 1–54.Google Scholar
Azih, C., Brinkerhoff, J. R. & Yaras, M. I. 2012 Direct numerical simulation of convective heat transfer in a zero-pressure-gradient boundary layer with supercritical water. J. Therm. Sci. 21, 49–59.Google Scholar
Brinkerhoff, J. R. & Yaras, M. I. 2011 Interaction of viscous and inviscid instability modes in separation-bubble transition. Phys. Fluids 23, 124102.CrossRefGoogle Scholar
Brinkerhoff, J. R. & Yaras, M. I. 2012 Direct numerical simulation of a square jet ejected transversely into an accelerating, laminar main flow. Flow Turbul. Combust. 89, 519–546.CrossRefGoogle Scholar
Cantwell, B., Coles, D. & Dimotakis, P. 1978 Structure and entrainment in the plane of symmetry of a turbulent spot. J. Fluid Mech. 87, 641–672.CrossRefGoogle Scholar
Choi, H. & Moin, P. 1994 Effects of the computational time step on numerical solutions of turbulent flow. J. Comput. Phys. 113, 1–4.CrossRefGoogle Scholar
Chong, T. P. & Zhong, S.2003 Development of turbulent wedges in favourable pressure gradients. AIAA Paper 2003-4245.Google Scholar
Chong, T. P. & Zhong, S. 2005 On the three-dimensional structure of turbulent spots. Trans. ASME J. Turbomach. 127, 545–552.CrossRefGoogle Scholar
Djenidi, L. & Antonia, R. A. 1993 LDA measurements in low Reynolds number turbulent boundary layer. Exp. Fluids 14, 280–288.Google Scholar
D’Ovidio, A., Harkins, J. A. & Gostelow, J. P.2001a Turbulent spots in strong adverse pressure gradients. Part 1. Spot behavior. ASME Paper 2001-GT-0194.Google Scholar
D’Ovidio, A., Harkins, J. A. & Gostelow, J. P.2001b Turbulent spots in strong adverse pressure gradients. Part 2. Spot propagation and spreading rates. ASME Paper 2001-GT-0406.CrossRefGoogle Scholar
Emmons, H. W. 1951 The laminar–turbulent transition in a boundary layer. Part 1. J. Aeronaut. Sci. 18, 490–498.Google Scholar
Fiedler, H. E. 1988 Coherent structures in turbulent flows. Prog. Aerosp. Sci. 25, 231–269.Google Scholar
Fric, T. F. & Roshko, A. 1994 Vortical structure in the wake of a transverse jet. J. Fluid Mech. 279, 1–47.Google Scholar
Gad-El-Hak, M., Blackwelder, R. F. & Riley, J. J. 1981 On the growth of turbulent regions in laminar boundary layers. J. Fluid Mech. 110, 73–95.Google Scholar
Gostelow, J. P., Melwani, N. & Walker, G. J. 1996 Effects of streamwise pressure gradients on turbulent spot development. Trans. ASME J. Turbomach. 118, 737–743.Google Scholar
Guo, H., Lian, Q. X., Li, Y. & Wang, H. W. 2004 A visual study on complex flow structures and flow breakdown in a boundary layer transition. Exp. Fluids 37, 311–322.Google Scholar
Henningson, D. S. & Alfredsson, P. H. 1987 The wave structure of turbulent spots in plane Poiseuille flow. J. Fluid Mech. 178, 405–421.Google Scholar
Henningson, D. S., Spalart, P. & Kim, J. 1987 Numerical simulations of turbulent spots in plane Poiseuille and boundary-layer flow. Phys. Fluids 30, 2914–2917.CrossRefGoogle Scholar
Honkan, A. & Andreopoulos, Y. 1997 Vorticity, strain-rate and dissipation characteristics in the near-wall region of turbulent boundary layers. J. Fluid Mech. 350, 29–96.Google Scholar
Jeong, J. & Hussain, F. 1995 On the identification of a vortex. J. Fluid Mech. 285, 69–94.CrossRefGoogle Scholar
Johnson, M. W. 2001 On the flow structure within a turbulent spot. Intl J. Heat Fluid Flow 22, 409–416.Google Scholar
Katz, Y., Seifert, A. & Wygnanski, I. 1990 On the evolution of the turbulent spot in a laminar boundary layer with a favourable pressure gradient. J. Fluid Mech. 221, 1–22.Google Scholar
Kim, H. T., Kline, S. J. & Reynolds, W. C. 1971 The production of turbulence near a smooth wall in a turbulent boundary layer. J. Fluid Mech. 50, 133–160.Google Scholar
Krishnan, L. & Sandham, N. D. 2007 Strong interaction of a turbulent spot with a shock-induced separation bubble. Phys. Fluids 19 (1), 016102.Google Scholar
Makita, H. & Nishizawa, A. 2001 Characteristics of internal vortical structures in a merged turbulent spot. J. Turbul. 2, 012.Google Scholar
McAuliffe, B. R. & Yaras, M. I. 2010 Transition mechanisms in separation bubbles under low- and elevated-freestream turbulence. Trans. ASME J. Turbomach. 132, 011004.CrossRefGoogle Scholar
Narasimha, R. 1985 The laminar–turbulent transition zone in the boundary layer. Prog. Aerosp. Sci. 22, 29–80.CrossRefGoogle Scholar
Ovchinnikov, V., Choudhari, M. M. & Piomelli, U. 2008 Numerical simulations of boundary-layer bypass transition due to high-amplitude free-stream turbulence. J. Fluid Mech. 613, 135–169.Google Scholar
Perry, A. E., Lim, T. T. & Teh, E. W. 1981 A visual study of turbulent spots. J. Fluid Mech. 104, 387–405.Google Scholar
Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.CrossRefGoogle Scholar
Roberts, S. K. & Yaras, M. I. 2005 Modeling transition in separated and attached boundary layers. Trans. ASME J. Turbomach. 127, 402–411.Google Scholar
Robinson, S. K. 1991 Coherent motions in the turbulent boundary layer. Annu. Rev. Fluid Mech. 23, 601–639.Google Scholar
Sabatino, D. R. & Smith, C. R. 2008 Turbulent spot flow topology and mechanisms for surface heat transfer. J. Fluid Mech. 612, 81–105.Google Scholar
Sankaran, R. & Antonia, R. A. 1988 Influence of a favourable pressure gradient on the growth of a turbulent spot. AIAA J. 26, 885–887.CrossRefGoogle Scholar
Schlichting, H. 1968 Boundary Layer Theory. McGraw-Hill.Google Scholar
Schoppa, W. & Hussain, F. 1997 Genesis and dynamics of coherent structures in near-wall turbulence: a new look. In Advances in Fluid Mechanics (ed. Panton, R. L.), vol. 15, pp. 385–422. Computational Mechanics Publications.Google Scholar
Schröder, A., Geisler, R., Elsinga, G. E., Scarano, F. & Dierksheide, U. 2008 Investigation of a turbulent spot and a tripped turbulent boundary layer flow using time-resolved tomographic PIV. Exp. Fluids 44, 305–316.Google Scholar
Schröder, A. & Kompenhans, J. 2004 Investigation of a turbulent spot using multi-plane stereo particle image velocimetry. Exp. Fluids 36, 82–90.Google Scholar
Schubauer, G. B. & Klebanoff, P. S.1956 Contributions on the mechanics of boundary layer transition. National Advisory Committee for Aeronautics Report 1289.Google Scholar
Singer, B. A. 1996 Characteristics of a young turbulent spot. Phys. Fluids 8, 509–521.Google Scholar
Singer, B. A. & Joslin, R. D. 1994 Metamorphosis of a hairpin vortex into a young turbulent spot. Phys. Fluids 6, 3724–3736.CrossRefGoogle Scholar
Smith, C. R., Walker, J. D. A., Haidari, A. H. & Sobrun, U. 1991 On the dynamics of near-wall turbulence. Phil. Trans. Phys. Sci. Engng 336, 131–175.Google Scholar
Spalart, P. R. 1988 Direct simulation of a turbulent boundary layer up to $Re_{{\it\theta}}=1410$ . J. Fluid Mech. 187, 61–98.Google Scholar
Stanislas, M., Perret, L. & Foucaut, J.-M. 2008 Vortical structures in the turbulent boundary layer: a possible route to a universal representation. J. Fluid Mech. 602, 327–382.Google Scholar
Strand, J. S. & Goldstein, D. B. 2011 Direct numerical simulations of riblets to constrain the growth of turbulent spots. J. Fluid Mech. 668, 267–292.Google Scholar
Volino, R. J. & Simon, T. W. 1995 Bypass transition in boundary layers including curvature and favorable pressure gradient effects. Trans. ASME J. Turbomach. 117, 166–174.Google Scholar
Wu, X. 2010 Establishing the generality of three phenomena using a boundary layer with free-stream passing wakes. J. Fluid Mech. 664, 193–219.Google Scholar
Wu, X. & Moin, P. 2009 Direct numerical simulation of turbulence in a nominally zero-pressure-gradient flat-plate boundary layer. J. Fluid Mech. 630, 5–41.Google Scholar
Wygnanski, I., Sokolov, M. & Friedman, D. 1976 On a turbulent ‘spot’ in a laminar boundary layer. J. Fluid Mech. 78, 785–819.CrossRefGoogle Scholar
Yaras, M. I. 2006 An experimental study of artificially generated turbulent spots under strong favorable pressure gradients and freestream turbulence. Trans. ASME J. Fluids Engng 129, 563–572.Google Scholar