Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-30T16:33:06.617Z Has data issue: false hasContentIssue false
  • English
  • Français

Numerical investigation of a jet from a blunt body opposing a supersonic flow

Published online by Cambridge University Press:  30 August 2011

Li-Wei Chen ,
Guo-Lei Wang  and
Xi-Yun Lu
Li-Wei Chen
Affiliation:
Department of Modern Mechanics, University of Science and Technology of China, Hefei, Anhui 230026, China
Guo-Lei Wang
Affiliation:
Department of Modern Mechanics, University of Science and Technology of China, Hefei, Anhui 230026, China
Xi-Yun Lu*
Affiliation:
Department of Modern Mechanics, University of Science and Technology of China, Hefei, Anhui 230026, China Modern Mechanics Division, E-Institutes of Shanghai Universities, Shanghai 200072, China
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Numerical investigation of a sonic jet from a blunt body opposing a supersonic flow with a free stream Mach number 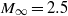was carried out using large-eddy simulation for two total pressure ratios of the jet to the free stream, i.e. 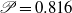and 1.633. Results have been validated carefully against experimental data. Various fundamental mechanisms dictating the flow phenomena, including shock/jet interaction, shock/shear-layer interaction, turbulent shear-layer evolution and coherent structures, have been studied systematically. Based on the analysis of the flow structures and features, two typical flow states, i.e. unstable and stable states corresponding to the two values of 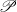, are identified and the behaviours relevant to the flow states are discussed. Small-scale vortical structures mainly occur in the jet column, and large-scale vortices develop gradually in a recirculation region when the jet terminates through a Mach disk and reverses its orientation as a conical free shear layer. The turbulent fluctuations are enhanced by the rapid deviation of the shear layer and the interaction with shock waves. Moreover, the coherent structures of the flow motion are analysed using the proper orthogonal decomposition technique. It is found that the dominant mode in the cross-section plane exhibits an antisymmetric character for the unstable state and an axisymmetric one for the stable state, while statistical analysis of unsteady loads indicates that the side loads can be seen as a rotating vector uniformly distributed in the azimuthal direction. Further, we clarify a feedback mechanism whereby the unsteady motion is sustained by the upstream-propagating disturbance to the Mach disk through the recirculation subsonic region and downstream propagation in the conical shear layer. Feedback models are then proposed which can reasonably well predict the dominant frequencies of the two flow states. The results obtained in this study provide physical insight into the understanding of the mechanisms relevant to the opposing jet/supersonic flow interaction.

JFM classification

Turbulent Flows: Compressible turbulence Wakes/Jets: Jets Turbulent Flows: Turbulence simulation
Type
Papers
Information
Journal of Fluid Mechanics , Volume 684 , 10 October 2011 , pp. 85 - 110
Copyright
Copyright © Cambridge University Press 2011

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Amatucci, V. A., Dutton, J. C., Kuntz, D. W. & Addy, A. L. 1992 Two stream, supersonic, wake flowfield behind a thick base. Part 1. General features. AIAA J. 30, 2039–2046.CrossRefGoogle Scholar
2. Andreopoulos, Y., Agui, J. H. & Briassulis, G. 2000 Shock wave-turbulence interactions. Annu. Rev. Fluid Mech. 32, 309–345.CrossRefGoogle Scholar
3. Berkooz, G., Holmes, P. & Lumley, J. L. 1993 The proper orthogonal decomposition in the analysis of turbulent flows. Annu. Rev. Fluid Mech. 25, 539–575.Google Scholar
4. Bodony, D. J. & Lele, S. K. 2005 On using large-eddy simulation for the prediction of noise from cold and heated turbulent jets. Phys. Fluids 17, 085103.CrossRefGoogle Scholar
5. Bogey, C. & Bailly, C. 2006 Large eddy simulations of transitional round jets: influence of the Reynolds number on flow development and energy dissipation. Phys. Fluids 18, 065101.CrossRefGoogle Scholar
6. Bourdon, C. J. & Dutton, J. C. 2000 Shear layer flapping and interface convolution in a separated supersonic flow. AIAA J. 38, 1907–1915.CrossRefGoogle Scholar
7. Bradshaw, P. 1967 The turbulence structure of equilibrium boundary layers. J. Fluid Mech. 29, 625–645.CrossRefGoogle Scholar
8. Bradshaw, P. 1974 The effect of mean compression or dilatation on the turbulence structure for supersonic boundary layers. J. Fluid Mech. 63, 449–464.CrossRefGoogle Scholar
9. Chen, L.-W., Xu, C.-Y. & Lu, X.-Y. 2010a Large eddy simulation of opposing-jet-perturbed supersonic flow past a hemispherical noses. Mod. Phys. Lett. B 24, 1287–1290.CrossRefGoogle Scholar
10. Chen, L.-W., Xu, C.-Y. & Lu, X.-Y. 2010b Numerical investigation of the compressible flow past an aerofoil. J. Fluid Mech. 643, 97–126.CrossRefGoogle Scholar
11. Debiève, J.-F., Ardissone, J.-P. & Dussauge, J.-P. 2003 Shock motion and state of turbulence in a perturbed supersonic flow around a sphere. J. Turbul. 4, 1–15.CrossRefGoogle Scholar
12. Deck, S. & Nguyen, A. T. 2004 Unsteady side loads in a thrust-optimized contour nozzle at hysteresis regime. AIAA J. 42, 1878–1888.CrossRefGoogle Scholar
13. Deck, S. & Thorigny, P. 2007 Unsteadiness of an axisymmetric separating-reattaching flow: numerical investigation. Phys. Fluids 19, 065103.CrossRefGoogle Scholar
14. Ferrante, A., Matheou, G. & Dimotakis, P. E. 2011 LES of an inclined sonic jet into a turbulent crossflow at Mach 3.6. J. Turbul. 12, 1–32.CrossRefGoogle Scholar
15. Finley, P. J. 1966 The flow of a jet from a body opposing a supersonic free stream. J. Fluid Mech. 26, 337–368.CrossRefGoogle Scholar
16. Freund, J. B., Lele, S. K. & Moin, P. 2000 Compressibility effects in a turbulent annular mixing layer. Part 1. Turbulence and growth rate. J. Fluid Mech. 421, 229–267.CrossRefGoogle Scholar
17. Fujita, M. 1995 Axisymmetric oscillations of an opposing jet from a hemispherical nose. AIAA J. 33, 1850–1856.CrossRefGoogle Scholar
18. Fujita, M. 2002 Three-dimensional oscillations of a supersonic opposing jet flow around a hemispherical nose. J. Japan Soc. Aeronaut. Space Sci. 50, 373–379.Google Scholar
19. Fujita, M. & Karashima, K. 1999 An experimental and computational study on self-excited oscillations in supersonic opposing jet flow. Trans. Japan Soc. Aeronaut. Space Sci. 42, 112–119.Google Scholar
20. Garnier, E., Sagaut, P. & Deville, M. 2002 Large eddy simulation of shock/homogeneous turbulence interaction. Comput. Fluids 31, 245–268.CrossRefGoogle Scholar
21. Génin, F. & Menon, S. 2010 Dynamics of sonic jet injection into supersonic crossflow. J. Turbul. 11, 1–30.CrossRefGoogle Scholar
22. Georgiadis, N. J., Rizzetta, D. P. & Fureby, C. 2010 Large-eddy simulation: current capabilities, recommended practices, and future research. AIAA J. 48, 1772–1784.CrossRefGoogle Scholar
23. Herrin, J. L. & Dutton, J. C. 1997 The turbulence structure of a reattaching axisymmetric compressible free shear layer. Phys. Fluids 9, 3502–3512.CrossRefGoogle Scholar
24. Hill, D. J., Pantano, C. & Pullin, D. I. 2006 Large-eddy simulation and multiscale modelling of a Richtmyer–Meshkov instability with reshock. J. Fluid Mech. 557, 29–61.CrossRefGoogle Scholar
25. Ho, C. M. & Nosseir, N. S. 1981 Dynamics of an impinging jet. Part 1. The feedback phenomenon. J. Fluid Mech. 105, 119–142.CrossRefGoogle Scholar
26. Hudy, L. M., Naguib, A. M. & Humphreys, W. M. 2003 Wall-pressure-array measurements beneath a separating/reattaching flow region. Phys. Fluids 15, 706–717.CrossRefGoogle Scholar
27. Jeong, J. & Hussain, F. 1995 On the identification of a vortex. J. Fluid Mech. 285, 69–94.CrossRefGoogle Scholar
28. Karashima, K. & Sato, K. 1975 An experimental study of an opposing jet. Bull. Inst. Space Aeronaut. Sci. Univ. Tokyo 11, 53–64.Google Scholar
29. Kawai, S. & Fujii, K. 2005 Computational study of supersonic base flow using hybrid turbulence methodology. AIAA J. 43, 1265–1275.CrossRefGoogle Scholar
30. Lu, X.-Y., Wang, S.-W., Sung, H. G., Hsieh, S. Y. & Yang, V. 2005 Large-eddy simulations of turbulent swirling flows injected into a dump chamber. J. Fluid Mech. 527, 171–195.CrossRefGoogle Scholar
31. Lumley, J. L. 1967 Rational approach to relations between motions of differing scales in turbulent flows. Phys. Fluids 10, 1405–1408.CrossRefGoogle Scholar
32. Matheou, G., Bonanos, A. M., Pantano, C. & Dimotakis, P. E. 2010 Large-eddy simulation of mixing in a recirculating shear flow. J. Fluid Mech. 646, 375–414.Google Scholar
33. Meyer, K. E., Pedersen, J. M. & Özcan, O. 2007 A turbulent jet in crossflow analysed with proper orthogonal decomposition. J. Fluid Mech. 583, 199–227.Google Scholar
34. Na, Y. & Moin, P. 1998 The structure of wall-pressure fluctuations in turbulent boundary layers with adverse pressure gradient and separation. J. Fluid Mech. 377, 347–373.CrossRefGoogle Scholar
35. Piomelli, U. 1999 Large-eddy simulation: achievements and challenges. Prog. Aerosp. Sci. 35, 335–362.CrossRefGoogle Scholar
36. Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.CrossRefGoogle Scholar
37. Pope, S. B. 2004 Ten questions concerning the large-eddy simulation of turbulent flows. New J. Phys. 6, 35.CrossRefGoogle Scholar
38. Rai, M. M. & Chakravarthy, S. 1993 Conservative high-order accurate finite difference method for curvilinear grids. AIAA Paper 93–3380.Google Scholar
39. Ribner, H. S. 1954Shock-turbulence interaction and the generation of noise. NACA Tech. Note 3255.Google Scholar
40. Robinet, J.-C. 2007 Bifurcations in shock wave/laminar boundary layer interaction: global instability approach. J. Fluid Mech. 579, 85–112.CrossRefGoogle Scholar
41. Robinson, S. K. 1991 Coherent motions in the turbulent boundary layers. Annu. Rev. Fluid Mech. 23, 601–639.Google Scholar
42. Rockwell, D. & Naudasher, E. 1979 Self-sustained oscillations of the impinging free shear layers. Annu. Rev. Fluid Mech. 11, 67–94.CrossRefGoogle Scholar
43. Shang, J. S. 2002 Plasma injection for hypersonic blunt-body drag reduction. AIAA J. 40, 1178–1186.CrossRefGoogle Scholar
44. Shang, J. S., Hayes, J., Wurtzler, K. & Strang, W. 2001 Jet-spike bifurcation in high-speed flows. AIAA J. 39, 1159–1165.CrossRefGoogle Scholar
45. Simon, F., Deck, S., Guillen, P., Sagaut, P. & Merlen, A. 2007 Numerical simulation of the compressible mixing layer past an axisymmetric trailing edge. J. Fluid Mech. 591, 215–253.CrossRefGoogle Scholar
46. Simpson, R. L., Ghodbane, M. & McGrath, B. E. 1987 Surface pressure fluctuations in a separating turbulent boundary layer. J. Fluid Mech. 177, 167–186.CrossRefGoogle Scholar
47. Tam, C. K. W. 1974 Discrete tones of isolated airfoils. J. Acoust. Soc. Am. 55, 1173–1177.CrossRefGoogle Scholar
48. Thomas, J. L. & Salas, M. D. 1986 Far-field boundary conditions for transonic lifting solutions to the Euler equations. AIAA J. 24, 1074–1080.CrossRefGoogle Scholar
49. Viti, V., Neel, R. & Schetz, J. A. 2009 Detailed flow physics of the supersonic jet interaction flow field. Phys. Fluids 21, 046101.CrossRefGoogle Scholar
50. Wang, S. W., Hsieh, S. Y. & Yang, V. 2005 Unsteady flow evolution in swirl injector with radial entry. I. Stationary conditions. Phys. Fluids 17, 045106.CrossRefGoogle Scholar
51. Warren, C. H. E. 1950 An experimental investigation of the effect of ejecting a coolant gas at the nose of a bluff body. J. Fluid Mech. 8, 400–417.CrossRefGoogle Scholar
52. Wu, J.-Z., Lu, X.-Y. & Zhuang, L.-X. 2007 Integral force acting on a body due to local flow structures. J. Fluid Mech. 576, 265–286.CrossRefGoogle Scholar
53. Xu, C.-Y., Chen, L.-W. & Lu, X.-Y. 2009 Numerical simulation of shock wave and turbulence interaction over a circular cylinder. Mod. Phys. Lett. B 23, 233–236.CrossRefGoogle Scholar
54. Xu, C.-Y., Chen, L.-W. & Lu, X.-Y. 2010 Large-eddy simulation of the compressible flow past a wavy cylinder. J. Fluid Mech. 665, 238–273.CrossRefGoogle Scholar
55. Yoda, M. & Fiedler, H. E. 1996 The round jet in a uniform counterflow: flow visualization and mean concentration measurements. Exp. Fluids 21, 427–436.CrossRefGoogle Scholar