Article contents
A novel subcritical transition to turbulence in Taylor–Couette flow with counter-rotating cylinders
Published online by Cambridge University Press: 02 April 2020
Abstract
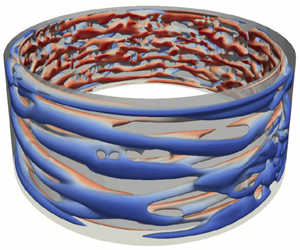
The transition to turbulence in Taylor–Couette flow often occurs via a sequence of supercritical bifurcations to progressively more complex, yet stable, flows. We describe a subcritical laminar–turbulent transition in the counter-rotating regime mediated by a transient intermediate state in a system with an axial aspect ratio of  $\unicode[STIX]{x1D6E4}=5.26$ and a radius ratio of
$\unicode[STIX]{x1D6E4}=5.26$ and a radius ratio of  $\unicode[STIX]{x1D702}=0.905$. In this regime, flow visualization experiments and numerical simulations indicate the intermediate state corresponds to an aperiodic flow featuring interpenetrating spirals. Furthermore, the reverse transition out of turbulence leads first to the same intermediate state, which is now stable, before returning to an azimuthally symmetric laminar flow. Time-resolved tomographic particle image velocimetry is used to characterize the experimental flows; these measurements compare favourably to direct numerical simulations with axial boundary conditions matching those of the experiments.
$\unicode[STIX]{x1D702}=0.905$. In this regime, flow visualization experiments and numerical simulations indicate the intermediate state corresponds to an aperiodic flow featuring interpenetrating spirals. Furthermore, the reverse transition out of turbulence leads first to the same intermediate state, which is now stable, before returning to an azimuthally symmetric laminar flow. Time-resolved tomographic particle image velocimetry is used to characterize the experimental flows; these measurements compare favourably to direct numerical simulations with axial boundary conditions matching those of the experiments.
JFM classification
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2020. Published by Cambridge University Press
References
- 11
- Cited by


