Hostname: page-component-586b7cd67f-dsjbd Total loading time: 0 Render date: 2024-11-25T03:28:13.170Z Has data issue: false hasContentIssue false
  • English
  • Français

A note on analytic solutions for entraining stratified gravity currents

Published online by Cambridge University Press:  11 December 2017

Marcus C. Horsley Open the ORCID record for Marcus C. Horsley [Opens in a new window]  and
Andrew W. Woods Open the ORCID record for Andrew W. Woods [Opens in a new window]
Marcus C. Horsley*
Affiliation:
BP Institute, University of Cambridge, Madingley Road, Cambridge CB3 0EZ, UK
Andrew W. Woods
Affiliation:
BP Institute, University of Cambridge, Madingley Road, Cambridge CB3 0EZ, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

High-Reynolds-number steady currents of relatively dense fluid propagating along a horizontal boundary become unstable and mix with the overlying fluid if the gradient Richardson number across the interface is less than 1/4. The process of entrainment produces a deepening mixing layer at the interface, which increases the gradient Richardson number of this layer and eventually may suppress further entrainment. The conservation of the vertically averaged buoyancy and momentum flux, as the current advances along the boundary, leads to two integral constraints relating the downstream flow with that upstream of the mixing zone. These constraints are equivalent to imposing a Froude number in the upstream flow. Using the ansatz that the dowstream velocity and buoyancy profiles in the current have a lower well-mixed region overlain by an interfacial layer of constant gradient, we can use these two constraints to quantify the total entrainment of ambient fluid into the flow as a function of the gradient Richardson number of the downstream flow. This leads to recognition that both subcritical and supercritical currents may develop downstream of the mixing zone. However, as the mixing increases and the interfacial layer gradually deepens, there is a critical point at which these two solution branches coincide. For each upstream Froude number, we can also determine the downstream flow with maximal entrainment. This maximal entrainment solution coincides with the convergence point of the supercritical and subcritical branches. We compare this with the entrainment predicted for those solutions with a gradient Richardson number of 1/4, which corresponds to the marginally stable case. As the upstream Froude number 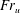$Fr_{u}$ increases, the maximum depth of the interfacial mixing layer gradually increases until eventually, for 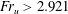$Fr_{u}>2.921$, the whole current may become modified through entrainment. We discuss the relevance of these results for mixing in gravity-driven flows.

JFM classification

Geophysical and Geological Flows: Gravity currents Geophysical and Geological Flows: Hydraulic control
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 836 , 10 February 2018 , pp. 260 - 276
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bursik, M. I. & Woods, A. W. 1996 The dynamics and thermodynamics of large ash flows. Bull. Volcanol. 58 (2), 175–193.CrossRefGoogle Scholar
Cenedese, C. & Adduce, C. 2010 A new parameterization for entrainment in overflows. J. Phys. Oceanogr. 40 (8), 1835–1850.Google Scholar
Cenedese, C., Nokes, R. & Hyatt, J.2016 Mixing in a density-driven current flowing over a rough bottom. In 20th Australasian Fluid Mechanics Conference, Perth, Australia, 2016. http://people.eng.unimelb.edu.au/imarusic/proceedings/20/610%20Paper.pdf.Google Scholar
Clarke, R. H. 1972 The morning glory: an atmospheric hydraulic jump. J. Appl. Meteorol. 11 (2), 304–311.Google Scholar
Ellison, T. H. & Turner, J. S. 1959 Turbulent entrainment in stratified flows. J. Fluid Mech. 6 (3), 423–448.Google Scholar
Fay, J. A. 1980 Gravitational spread and dilution of heavy vapor clouds. In Second International Symposium on Stratified Flows, Norwegian Institute of Technology, Trondheim, Norway (ed. Carstens, T. & McClimans, T.), pp. 24–27.Google Scholar
Garvine, R. W. & Monk, J. D. 1974 Frontal structure of a river plume. J. Geophys. Res. 79 (15), 2251–2259.Google Scholar
Hallworth, M. A., Phillips, J. C., Huppert, H. E. & Sparks, R. S. J. 1993 Entrainment in turbulent gravity currents. Nature 362 (6423), 829–831.Google Scholar
Hogg, A. J., Nasr-Azadani, M. M., Ungarish, M. & Meiburg, E. 2016 Sustained gravity currents in a channel. J. Fluid Mech. 798, 853–888.Google Scholar
Holland, D. M., Rosales, R. R., Stefanica, D. & Tabak, E. G. 2002 Internal hydraulic jumps and mixing in two-layer flows. J. Fluid Mech. 470, 63–83.Google Scholar
Ivey, G. N., Winters, K. B. & Koseff, J. R. 2008 Density stratification, turbulence, but how much mixing? Annu. Rev. Fluid Mech. 40, 169–184.CrossRefGoogle Scholar
Johnson, C. G. & Hogg, A. J. 2013 Entraining gravity currents. J. Fluid Mech. 731, 477–508.Google Scholar
Kneller, B. M., Nasr-Azadani, M. M., Radhakrishnan, S. & Meiburg, E. 2016 Long-range sediment transport in the world’s oceans by stably stratified turbidity currents. J.  Geophys. Res. 121, 8608–8620.Google Scholar
Liapidevskii, V. Y. 1994 Mixing and blocking effects in two-layer flow over an obstacle. In Fourth International Symposium on Stratified Flows, Grenoble, France, vol. 4, pp. 183–189.Google Scholar
Monti, P., Querzoli, G., Cenedese, A. & Piccinini, S. 2007 Mixing properties of a stably stratified parallel shear layer. Phys. Fluids 19 (8), 085104.Google Scholar
Samasiri, P. & Woods, A. W. 2015 Mixing in axisymmetric gravity currents. J. Fluid Mech. 782, R1.Google Scholar
Sher, D. & Woods, A. W. 2015 Gravity currents: entrainment, stratification and self-similarity. J. Fluid Mech. 784, 130–162.Google Scholar
Sher, D. & Woods, A. W. 2017 Mixing in continuous gravity currents. J. Fluid Mech. 818, doi:10.1017/jfm.2017.168.Google Scholar
Smyth, W. D. & Moum, J. N. 2012 Ocean mixing by Kelvin–Helmholtz instability. Oceanography 25, 140–149.Google Scholar
Strang, E. J. & Fernando, H. J. S. 2001 Entrainment and mixing in stratified shear flows. J. Fluid Mech. 428, 349–386.Google Scholar
Turner, J. S. 1979 Buoyancy Effects in Fluids. Cambridge University Press.Google Scholar
Xu, X., Chang, Y. S., Peters, H., Özgökmen, T. M. & Chassignet, E. P. 2006 Parameterization of gravity current entrainment for ocean circulation models using a high-order 3D nonhydrostatic spectral element model. Ocean Model. 14 (1), 19–44.Google Scholar