Hostname: page-component-586b7cd67f-t7czq Total loading time: 0 Render date: 2024-11-28T13:48:53.376Z Has data issue: false hasContentIssue false
  • English
  • Français

Multiple states in turbulent plane Couette flow with spanwise rotation

Published online by Cambridge University Press:  28 December 2017

Zhenhua Xia Open the ORCID record for Zhenhua Xia [Opens in a new window] ,
Yipeng Shi ,
Qingdong Cai ,
Minping Wan  and
Shiyi Chen
Zhenhua Xia*
Affiliation:
Department of Engineering Mechanics, Zhejiang University, Hangzhou 310027, China
Yipeng Shi
Affiliation:
State Key Laboratory for Turbulence and Complex Systems, Center for Applied Physics and Technology, College of Engineering, Peking University, Beijing 100871, China
Qingdong Cai
Affiliation:
State Key Laboratory for Turbulence and Complex Systems, Center for Applied Physics and Technology, College of Engineering, Peking University, Beijing 100871, China
Minping Wan
Affiliation:
Department of Mechanics and Aerospace Engineering, Southern University of Science and Technology, Shenzhen 518055, China
Shiyi Chen*
Affiliation:
Department of Engineering Mechanics, Zhejiang University, Hangzhou 310027, China State Key Laboratory for Turbulence and Complex Systems, Center for Applied Physics and Technology, College of Engineering, Peking University, Beijing 100871, China Department of Mechanics and Aerospace Engineering, Southern University of Science and Technology, Shenzhen 518055, China
*
†Email addresses for correspondence: [email protected], [email protected]
†Email addresses for correspondence: [email protected], [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Turbulence is ubiquitous in nature and engineering applications. Although Kolmogorov’s (C. R. Acad. Sci. URSS, vol. 30, 1941a, pp. 301–305; Dokl. Akad. Nauk URSS, vol. 30, 1941b, pp. 538–540) theory suggested a unique turbulent state for high Reynolds numbers, multiple states were reported for several flow problems, such as Rayleigh–Bénard convection and Taylor–Couette flows. In this paper, we report that multiple states also exist for turbulent plane Couette flow with spanwise rotation through direct numerical simulations at rotation number $Ro=0.2$ and Reynolds number 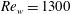$Re_{w}=1300$ based on the angular velocity in the spanwise direction and half of the wall velocity difference. With two different initial flow fields, our results show that the flow statistics, including the mean streamwise velocity and Reynolds stresses, show different profiles. These different flow statistics are closely related to the flow structures in the domain, where one state corresponds to two pairs of roll cells, and the other shows three pairs. The present result enriches the studies on multiple states in turbulence.

JFM classification

Turbulent Flows: Rotating turbulence Turbulent Flows: Turbulence simulation Turbulent Flows: Turbulent Flows
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 837 , 25 February 2018 , pp. 477 - 490
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahlers, G., Funfschilling, D. & Bodenschatz, E. 2011 Heat transport in turbulent Rayleigh–Bénard convection for Pr ≃ 0. 8 and Ra ≲ 1015 . J. Phys.: Conf. Ser. 318, 082001.Google Scholar
Alfredsson, P. H. & Tillmark, N. 2005 Instability, transition and turbulence in plane Couette flow with system rotation. In Laminar Turbulent Transition and Finite Amplitude Solutions (ed. Mullin, T. & Kerswell, R. R.), pp. 173–193. Springer.Google Scholar
Andereck, C. D., Liu, S. S. & Swinney, H. L. 1986 Flow regimes in a circular Couette system with independently rotating cylinders. J. Fluid Mech. 164, 155–183.Google Scholar
Barri, M. & Andersson, H. I. 2007 Anomalous turbulence in rapidly rotating plane Couette flow. In Advances in Turbulence XI (ed. Palma, J. M. L. M. & Silva Lopes, A.), pp. 100–102. Springer.Google Scholar
Barri, M. & Andersson, H. I. 2010 Computer experiments on rapidly rotating plane Couette flow. Commun. Comput. Phys. 7, 683–717.Google Scholar
Bech, K. H. & Andersson, H. I. 1996 Secondary flow in weakly rotating turbulent plane Couette flow. J. Fluid Mech. 317, 195–214.Google Scholar
Bech, K. H. & Andersson, H. I. 1997 Turbulent plane Couette flow subject to strong system rotation. J. Fluid Mech. 347, 289–314.Google Scholar
Brauckmann, H. J., Salewski, M. & Eckhardt, B. 2016 Momentum transport in Taylor–Couette flow with vanishing curvature. J. Fluid Mech. 790, 419–452.Google Scholar
Cortet, P. P., Chiffaudel, A., Daviaud, F. & Dubrulle, B. 2010 Experimental evidence of a phase transition in a closed turbulent flow. Phys. Rev. Lett. 105, 214501.Google Scholar
Eckhardt, B., Schneider, T. M., Hof, B. & Westerweel, J. 2007 Turbulence transition in pipe flow. Annu. Rev. Fluid Mech. 39, 447–468.Google Scholar
Faisst, H. & Eckhardt, B. 2000 Transition from the Couette–Taylor system to the plane Couette system. Phys. Rev. E 61, 7227–7230.Google Scholar
Gai, J., Xia, Z. H., Cai, Q. D. & Chen, S. Y. 2016 Turbulent statistics and flow structures in spanwise-rotating turbulent plane Couette flows. Phys. Rev. Fluids 1, 054401.Google Scholar
Grossmann, S. & Lohse, D. 2011 Multiple scaling in the ultimate regime of thermal convection. Phys. Fluids 23, 045108.Google Scholar
Grossmann, S., Lohse, D. & Sun, C. 2016 High-Reynolds number Taylor–Couette turbulence. Annu. Rev. Fluid Mech. 48, 53–80.Google Scholar
Hiwatashi, K., Alfredsson, P. H., Tillmark, N. & Nagata, M. 2007 Experimental observations of instabilities in rotating plane Couette flow. Phys. Fluids 19, 048103.Google Scholar
Hsieh, A. & Biringen, S. 2016 The minimal flow unit in complex turbulent flows. Phys. Fluids 28, 125102.Google Scholar
Huang, Y. H., Xia, Z. H., Wan, M. P. & Chen, S. Y.2017 Numerical investigations on turbulent spanwise rotating plane Couette flow at $Ro=0.02$ . Phys. Rev. Fluids (submitted).Google Scholar
Huisman, S. G.2010 Local velocity measurements in Twente turbulent Taylor–Couette. Master thesis, University of Twente.Google Scholar
Huisman, S. G., van der Veen, R. C. A., Sun, C. & Lohse, D. 2014 Multiple states in highly turbulent Taylor–Couette flow. Nat. Commun. 5, 3820.Google Scholar
Iyer, K. P., Bonaccorso, F., Biferale, L. & Toschi, F. 2017 Multiscale anisotropic fluctuations in sheared turbulence with multiple states. Phys. Rev. Fluids 2, 052602(R).Google Scholar
Jakirlić, S., Hanjalić, K. & Tropea, C. 2002 Modeling rotating and swirling turbulent flows: a perpetual challenge. AIAA J. 40, 1984–1996.Google Scholar
Jiménez, J. & Moin, P. 1991 The minimal flow units in near-wall turbulence. J. Fluid Mech. 225, 213–240.Google Scholar
Kachanov, Y. S. 1994 Physical mechanisms of laminar-boundary-layer transition. Annu. Rev. Fluid Mech. 26, 411–482.Google Scholar
Kasagi, N., Sumitani, Y., Suzuki, Y. & Iida, O. 1995 Kinematics of the quasi-coherent vortical structure in near-wall turbulence. Intl J. Heat Fluid Flow 16, 2–10.Google Scholar
Kawata, T. & Alfredsson, P. H. 2016a Experiments in rotating plane Couette flow: momentum transport by coherent roll-cell structure and zero-absolute-vorticity state. J. Fluid Mech. 791, 191–213.Google Scholar
Kawata, T. & Alfredsson, P. H. 2016b Turbulent rotating plane Couette flow: Reynolds and rotation number dependency of flow structure and momentum transport. Phys. Rev. Fluids 1, 034402.Google Scholar
Kolmogorov, A. N. 1941a The local structure of turbulence in incompressible viscous fluid for very large Reynolds numbers. C. R. Acad. Sci. URSS 30, 301–305.Google Scholar
Kolmogorov, A. N. 1941b On degeneration of isotropic turbulence in an incompressible viscous liquid. Dokl. Akad. Nauk URSS 30, 538–540.Google Scholar
Kristoffersen, R. & Andersson, H. I. 1993 Direct simulations of low-Reynolds-number turbulent flow in a rotating channel. J. Fluid Mech. 256, 163–197.Google Scholar
Kundu, P. k., Cohen, I. M., Dowling, D. R. & Tryggvason, G. 2015 Fluid Mechanics, 6th edn., p. 771. Academic.Google Scholar
Lee, C. B. & Wu, J. Z. 2008 Transition in wall-bounded flows. Appl. Mech. Rev. 61, 030802.Google Scholar
Lee, M. J. & Kim, J. 1991 The structure of turbulence in a simulated plane Couette flow. In Proceedings of Eighth Symposium on Turbulent Shear Flows, Munich, Germany (ed. Durst, F. et al. ), paper 5-3, Springer.Google Scholar
Martínez-Arias, B., Peixinho, J., Crumeyrolle, O. & Mutabazi, I. 2014 Effect of the number of vortices on the torque scaling in Taylor–Couette flow. J. Fluid Mech. 748, 756–767.Google Scholar
Moser, R. D. & Moin, P. 1987 The effects of curvature in wall-bounded turbulent flows. J. Fluid Mech. 175, 479–510.Google Scholar
Nagata, M. 1998 Tertiary solutions and their stability in rotating plane Couette flow. J. Fluid Mech. 358, 357–378.Google Scholar
van der Poel, E. P., Stevens, R. J. A. M. & Lohse, D. 2011 Connecting flow structures and heat flux in turbulent Rayleigh–Bénard convection. Phys. Rev. E 84, 045303.Google Scholar
Ravelet, F., Chiffaudel, A. & Daviaud, F. 2008 Supercritical transition to turbulence in an inertially driven von Kármán closed flow. J. Fluid Mech. 601, 339–364.Google Scholar
Ravelet, F., Marié, L., Chiffaudel, A. & Daviaud, F. 2004 Multistability and memory effect in a highly turbulent flow: experimental evidence for a global bifurcation. Phys. Rev. Lett. 93, 164501.Google Scholar
Reynolds, O. 1894 On the dynamical theory of incompressible viscous flows and the determination of the criterion. Phil. Trans. R. Soc. Lond. A 186, 123–161.Google Scholar
Salewski, M. & Eckhardt, B. 2015 Turbulent states in plane Couette flow with rotation. Phys. Fluids 27, 045109.Google Scholar
Stevens, R. J. A. M., Clercx, H. J. H. & Lohse, D. 2012 Breakdown of the large-scale circulation in 𝛾 = 1/2 rotating Rayleigh–Bénard flow. Phys. Rev. E 86, 056311.Google Scholar
Suryadi, A., Segalini, A. & Alfredsson, P. H. 2014 Zero absolute vorticity: insight from experiments in rotating laminar plane Couette flow. Phys. Rev. E 89, 033003.Google Scholar
Tillmark, N. & Alfredsson, P. H. 1996 Experiments on rotating plane Couette flow. In Advances in Turbulence VI (ed. Gavrilakis, S., Machiels, L. & Monkewitz, P. A.), pp. 391–394. Kluwer.Google Scholar
Tsukahara, T. 2011 Structures and turbulent statistics in a rotating plane Couette flow. J. Phys.: Conf. Ser. 318, 022024.Google Scholar
Tsukahara, T., Tillmark, N. & Alfredsson, P. H. 2010 Flow regimes in a plane Couette flow with system rotation. J. Fluid Mech. 648, 5–33.Google Scholar
Van der Veen, R. C. A., Huisman, S. G., Dung, O.-Y., Tang, H. L., Sun, C. & Lohse, D. 2016 Exploring the phase space of multiple states in highly turbulent Taylor–Couette flows. Phys. Rev. Fluids 1, 024401.Google Scholar
Weiss, S. & Ahlers, G. 2013 Effect of tilting on turbulent convection: cylindrical samples with aspect ratio 𝛤 = 0. 50. J. Fluid Mech. 715, 314–334.Google Scholar
Xi, H. D. & Xia, K. Q. 2008 Flow mode transitions in turbulent thermal convection. Phys. Fluids 20, 055104.Google Scholar
Zimmerman, D. S., Triana, S. A. & Lathrop, D. P. 2011 Bi-stability in turbulent, rotating spherical Couette flow. Phys. Fluids 23, 065104.Google Scholar

Xia et al. supplementary movie 1

Snapshots of instantaneous velocity vectors in cross-section at x=0 from Case R2_512

Download Xia et al. supplementary movie 1(Video)
Video 5.7 MB

Xia et al. supplementary movie 2

Snapshots of instantaneous velocity vectors in cross-section at x=0 from Case R3_512

Download Xia et al. supplementary movie 2(Video)
Video 5.1 MB