Hostname: page-component-586b7cd67f-l7hp2 Total loading time: 0 Render date: 2024-11-30T23:45:17.900Z Has data issue: false hasContentIssue false
  • English
  • Français

Modelling the decay of finite-span starting and stopping wall jets in an external stream

Published online by Cambridge University Press:  08 November 2022

Ben Steinfurth Open the ORCID record for Ben Steinfurth [Opens in a new window]  and
Julien Weiss Open the ORCID record for Julien Weiss [Opens in a new window]
Ben Steinfurth*
Affiliation:
Institute of Aeronautics and Astronautics, Technische Universität Berlin, Marchstr. 12–14, 10587 Berlin, Germany
Julien Weiss
Affiliation:
Institute of Aeronautics and Astronautics, Technische Universität Berlin, Marchstr. 12–14, 10587 Berlin, Germany
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

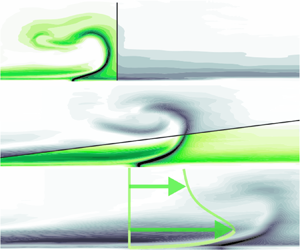
The flow under consideration is a wall jet that results from an inclined jet in cross-flow emitted into a zero-pressure-gradient turbulent boundary layer from a slot-like outlet of width $b=0.5\,\mathrm {mm}$ and span $L=20\,\mathrm {mm}$. Despite the finite jet span, the velocity decay and wall-normal spreading rate in the symmetry plane can be described with power laws almost identical to those for the two-dimensional flow determined by Zhou & Wygnanski (AIAA J., vol. 31, 1993, pp. 848–853). This is explained by the lack of significant lateral spreading found in the present configuration due to a self-amplifying inward-directed fluid motion, fundamentally differing from conditions found in the absence of an external stream. Regions with ‘approximately self-similar’ properties also exist in the case of unsteady velocity programmes where the fluid is ejected in a pulsatile fashion. Here, the wall jet is enclosed by a leading vortex structure and a deceleration wave, for which the time-dependent locations can be predicted by means of empirical constants. This yields models for the major properties inside the advancing and diffusing wall jet that only require knowledge regarding the velocity ratio, the ejected momentum flux and the kinematic viscosity, representing an extension to scaling laws for steady wall jets in still ambience established by Narasimha et al. (Aeronaut. Q., vol. 77, 1973, pp. 355–359).

JFM classification

Boundary Layers: Boundary layer control Vortex Flows: Vortex dynamics Wakes/Jets: Jets
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 951 , 25 November 2022 , A28
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Abrahamsson, H., Johansson, B. & Löfdahl, L. 1996 An investigation of the turbulence field in a three-dimensional wall jet. In Advances in Turbulence VI. Fluid Mechanics and its Applications (ed. S. Gavrilakis, L. Machiels & P.A. Monkewitz), vol. 36, pp. 417–420. Springer.Google Scholar
Akima, H. 1970 A new method of interpolation and smooth curve fitting based on local procedures. J. ACM 17 (4), 589–602.CrossRefGoogle Scholar
Artham, S., Zhang, Z. & Gnanamanickam, E.P. 2021 Inner–outer interactions in a forced plane wall jet. Exp. Fluids 62, 35.Google Scholar
Bakke, P. 1957 An experimental investigation of a wall jet. J. Fluid Mech. 2, 467–472.CrossRefGoogle Scholar
Bhatt, S. & Gnanamanickam, E. 2020 Linear and nonlinear mechanisms within a forced plane wall jet. Phys. Rev. Fluids 5 (7), 074604.CrossRefGoogle Scholar
Bradshaw, P. & Gee, M.T. 1962 Turbulent wall jets with and without an external stream. Aeronaut. Res. Counc. R&M 3252.Google Scholar
Charonko, J.J. & Vlachos, P.P. 2013 Estimation of uncertainty bounds for individual particle image velocimetry measurements from cross-correlation peak ratio. Meas. Sci. Technol. 24 (6), 065301.Google Scholar
Craft, T.J. & Launder, B.E. 2001 On the spreading mechanism of the three-dimensional turbulent wall jet. J. Fluid Mech. 435, 305–326.CrossRefGoogle Scholar
Davis, M.R. & Winarto, H. 1980 Jet diffusion from a circular nozzle above a solid plane. J. Fluid Mech. 730, 626–658.Google Scholar
Förthmann, E. 1934 Über turbulente Strahlausbreitung. Ing.-Arch. 5 (1), 42–54.Google Scholar
Gartshore, I.S. & Newman, B.G. 1969 The turbulent wall jet in an arbitrary pressure gradient. Aeronaut. Q. 20 (1), 25–56.CrossRefGoogle Scholar
George, W.K., Abrahamsson, H., Eriksson, J., Karlsson, R.I., Löfdahl, L. & Wosnik, M. 2000 A similarity theory for the turbulent plane wall jet without external stream. J. Fluid Mech. 425, 367–411.CrossRefGoogle Scholar
Glauert, M.B. 1956 The wall jet. J. Fluid Mech. 1, 625–643.Google Scholar
Gnanamanickam, E.P., Bhatt, S., Artham, S. & Zhang, Z. 2019 Large-scale motions in a plane wall jet. J. Fluid Mech. 877, 239–281.Google Scholar
Greenblatt, D. & Wygnanski, I.J. 2000 The control of flow separation by periodic excitation. Prog. Aerosp. Sci. 36 (7), 487–545.CrossRefGoogle Scholar
Gupta, A., Choudhary, H., Singh, A.K., Prabhakaran, T. & Dixit, S.A. 2020 Scaling mean velocity in two-dimensional turbulent wall jets. J. Fluid Mech. 891, A11.Google Scholar
Keane, R.D. & Adrian, R.J. 1992 Theory of cross-correlation analysis of PIV images. Appl. Sci. Res. 49, 191–215.Google Scholar
Kebede, W. 1982 Numerical computations of the 3-dimensional wall jet. MSc thesis, University of Manchester.Google Scholar
Kruka, V. & Eskinazi, S. 1964 The wall-jet in a moving stream. J. Fluid Mech. 20 (4), 555–579.CrossRefGoogle Scholar
Lai, J.C.S. & Lu, D. 1996 Effect of wall inclination on the mean flow and turbulence characteristics in a two-dimensional wall jet. Intl J. Heat Fluid Flow 17 (4), 377–385.CrossRefGoogle Scholar
Launder, B.E. & Rodi, W. 1979 The turbulent wall jet. Prog. Aerosp. Sci. 19, 81–128.Google Scholar
Launder, B.E. & Rodi, W. 1983 The turbulent wall jet - measurements and modeling. Annu. Rev. Fluid Mech. 15, 429–459.Google Scholar
Myers, G.E., Schauer, J.J. & Eustis, R.H. 1963 Plane turbulent wall jet flow development and friction factor. J. Fluid Engng 85 (1), 47–53.Google Scholar
Naqavi, I.Z., Tyacke, J.C. & Tucker, P.G. 2018 Direct numerical simulation of a wall jet: flow physics. J. Fluid Mech. 852, 507–542.CrossRefGoogle Scholar
Narasimha, R., Narayan, K.Y. & Parthasarathy, S.P. 1973 Parametric analysis of turbulent wall jets in still air. Aeronaut. Q. 77 (751), 355–359.Google Scholar
Newman, B.G., Patel, R.P., Savage, S.B. & Tjio, H.K. 1972 Three-dimensional wall jet originating from a circular orifice. Aeronaut. Q. 23 (3), 188–200.CrossRefGoogle Scholar
Pai, B.R. & Whitelaw, J.H. 1971 The prediction of wall temperature in the presence of film cooling. Intl J. Heat Mass Transfer 14 (3), 409–426.Google Scholar
Patel, R.P. 1962 Self preserving, two-dimensional turbulent jets and wall jets in a moving stream. PhD thesis. McGill University, Montreal.Google Scholar
Schwarz, W.H. & Cosart, W.P. 1961 The two-dimensional turbulent wall-jet. J. Fluid Mech. 10, 481–495.CrossRefGoogle Scholar
Seban, R.A. & Back, L.H. 1961 Velocity and temperature profiles in a wall jet. Intl J. Heat Mass Transfer 3, 255–265.CrossRefGoogle Scholar
Sforza, P.M. & Herbst, G. 1970 A study of three-dimensional, incompressible, turbulent wall jets. AIAA J. 8 (1), 276–282.CrossRefGoogle Scholar
Sigalla, A. 1958 Measurements of skin friction in a plane turbulent wall jet. Aeronaut. Q. 62, 873–877.Google Scholar
Steinfurth, B. & Weiss, J. 2020 Vortex rings produced by non-parallel planar starting jets. J. Fluid Mech. 903, A16.Google Scholar
Steinfurth, B. & Weiss, J. 2021 a Boundary-layer control by means of pulsed jets at different inclination angles. AIAA J. 59 (8), 3946–3959.CrossRefGoogle Scholar
Steinfurth, B. & Weiss, J. 2021 b Development of inclined pulsed jets in an adverse-pressure-gradient crossflow. AIAA Paper 2021-2837.Google Scholar
Steinfurth, B. & Weiss, J. 2021 c Velocity ratio effect on flow structures of non-parallel planar starting jets in cross-flow. J. Fluid Mech. 915, A11.Google Scholar
Swean, T.F., Ramberg, S.E., Plesniak, M.W. & Stewart, M.B. 1989 Turbulent surface jet in channel of limited depth. J. Hydraul. Engng 115 (12), 1587–1606.CrossRefGoogle Scholar
Thomas, F. 1963 Untersuchungen über die Grenzschicht an einer Wand stromabwärts von einem Ausblasespalt. In Abhandlungen der Braunschweigischen Wissenschaftlichen Gesellschaft (ed. H.J. Bogen), vol. 15, pp. 1–17. Vieweg.Google Scholar
Tollmien, W. 1926 Berechnung turbulenter Ausbreitungsvorgänge. Z. Angew. Math. Mech. 6 (6), 468–478.Google Scholar
Weiss, J., Jondeau, E., Giani, A., Charlot, B. & Combette, P. 2017 a Static and dynamic calibration of a MEMS calorimetric shear-stress sensor. Sensors Actuators A 265, 211–216.CrossRefGoogle Scholar
Weiss, J., Schwaab, Q., Boucetta, Y., Giani, A., Guigue, C., Combette, P. & Charlot, B. 2017 b Simulation and testing of a MEMS calorimetric shear-stress sensor. Sensors Actuators A 253, 210–217.CrossRefGoogle Scholar
Weiss, J., Steinfurth, B., Chamard, L., Giani, A. & Combette, P. 2022 Spectral proper orthogonal decomposition of unsteady wall shear stress under a turbulent separation bubble. AIAA J. 60 (4), 2150–2159.Google Scholar
Wille, R. & Fernholz, H. 1965 Report on the first European Mechanics Colloquium, on the Coanda effect. J. Fluid Mech. 24 (4), 801–819.CrossRefGoogle Scholar
Wygnanski, I., Katz, Y. & Horev, E. 1992 On the applicability of various scaling laws to the turbulent wall jet. J. Fluid Mech. 234, 669–690.Google Scholar
Zhou, M.D. & Wygnanski, I. 1993 Parameters governing the turbulent wall jet in an external stream. AIAA J. 31 (5), 848–853.CrossRefGoogle Scholar

Steinfurth and Weiss supplementary movie 1

Visualisation of the extraction of time-dependent propagation front location $x_p/b$; upper diagram: FTLE field with $x_p/b$ marked by red dashed vertical line, lower diagram: jet spreading rates for steady and starting wall jets with $x_p/b$ marked by red circle
Download Steinfurth and Weiss supplementary movie 1(Video)
Video 1.7 MB

Steinfurth and Weiss supplementary movie 2

Visualisation of the extraction of time-dependent deceleration wave location $x_d/b$; upper diagram: FTLE field with $x_d/b$ marked by red dashed vertical line, lower diagram: maximum jet velocities for steady and starting wall jets with $x_d/b$ marked by red circle

Download Steinfurth and Weiss supplementary movie 2(Video)
Video 759.5 KB