Hostname: page-component-586b7cd67f-2plfb Total loading time: 0 Render date: 2024-11-24T16:58:13.389Z Has data issue: false hasContentIssue false
  • English
  • Français

Miscible displacement flows in near-horizontal ducts at low Atwood number

Published online by Cambridge University Press:  27 February 2012

S. M. Taghavi ,
K. Alba ,
T. Seon ,
K. Wielage-Burchard ,
D. M. Martinez  and
I. A. Frigaard
S. M. Taghavi
Affiliation:
Department of Chemical and Biological Engineering, University of British Columbia, 2360 East Mall, Vancouver, British Columbia, V6T 1Z3, Canada
K. Alba
Affiliation:
Department of Mechanical Engineering, University of British Columbia, 2054-6250 Applied Science Lane, Vancouver, British Columbia, V6T 1Z4, Canada
T. Seon
Affiliation:
Université Pierre et Marie Curie, Institut d’Alembert, 4 place Jussieu, 75005 Paris, France
K. Wielage-Burchard
Affiliation:
Department of Mathematics, University of British Columbia, 1984 Mathematics Road, Vancouver, British Columbia, V6T 1Z2, Canada
D. M. Martinez
Affiliation:
Department of Chemical and Biological Engineering, University of British Columbia, 2360 East Mall, Vancouver, British Columbia, V6T 1Z3, Canada
I. A. Frigaard*
Affiliation:
Department of Mechanical Engineering, University of British Columbia, 2054-6250 Applied Science Lane, Vancouver, British Columbia, V6T 1Z4, Canada Department of Mathematics, University of British Columbia, 1984 Mathematics Road, Vancouver, British Columbia, V6T 1Z2, Canada
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We study buoyant displacement flows with two miscible fluids of equal viscosity in the regime of low Atwood number and in ducts that are inclined close to horizontal. Using a combination of experimental, computational and analytical methods, we characterize the transitions in the flow regimes between inertial- and viscous-dominated regimes, and as the displacement flow rate is gradually increased. 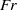Three dimensionless groups largely describe these flows: densimetric Froude number , Reynolds number 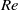and duct inclination . Our results show that the flow regimes collapse into regions in a two-dimensional 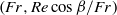plane. These regions are qualitatively similar between pipes and plane channels, although viscous effects are more extensive in pipes. In each regime, we are able to give a leading-order estimate for the velocity of the leading displacement front, which is effectively a measure of displacement efficiency.

JFM classification

Geophysical and Geological Flows: Gravity currents Low-Reynolds-number flows: Lubrication theory Multiphase and Particle-laden Flows: Multiphase flow
Type
Papers
Information
Journal of Fluid Mechanics , Volume 696 , 10 April 2012 , pp. 175 - 214
Copyright
Copyright © Cambridge University Press 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Alba, K., Laure, P. & Khayat, R. E. 2011 Transient two-layer thin-film flow inside a channel. Phys. Rev. E 84, 026320.CrossRefGoogle ScholarPubMed
2. Amaouche, M., Mehidi, N. & Amatousse, N. 2007 Linear stability of a two-layer film flow down an inclined channel: a second-order weighted residual approach. Phys. Fluids 19, 084106.CrossRefGoogle Scholar
3. Baird, M. H. I., Aravamudan, K., Rao, N. V. Rama, Chadam, J. & Peirce, A. P. 1992 Unsteady axial mixing by natural convection in vertical column. AIChE J. 38, 1825.CrossRefGoogle Scholar
4. Beckett, F. M., Mader, H. M., Phillips, J. C., Rust, A. C. & Witham, F. 2011 An experimental study of low Reynolds number exchange flow of two Newtonian fluids in a vertical pipe. J. Fluid Mech. 682, 652–670.CrossRefGoogle Scholar
5. Benjamin, T. J. 1968 Gravity currents and related phenomena. J. Fluid Mech. 31, 209–248.CrossRefGoogle Scholar
6. Birman, V. K., Battandier, B. A., Meiburg, E. & Linden, P. F. 2007 Lock-exchange flows in sloping channels. J. Fluid Mech. 577, 53–77.CrossRefGoogle Scholar
7. Birman, V. K., Martin, J. E., Meiburg, E. & Linden, P. F. 2005 The non-Boussinesq lock-exchange problem. Part 2. High-resolution simulations. J. Fluid Mech. 537, 125–144.CrossRefGoogle Scholar
8. Charru, F. & Hinch, E. J. 2000 Phase diagram of interfacial instabilities in a two-layer Couette flow and mechanism of the long wave instability. J. Fluid Mech. 414, 195–223.CrossRefGoogle Scholar
9. Chen, C.-Y. & Meiburg, E. 1996 Miscible displacements in capillary tubes. Part 2. Numerical simulations. J. Fluid Mech. 326, 57–90.CrossRefGoogle Scholar
10. Debacq, M., Fanguet, V., Hulin, J. P., Salin, D. & Perrin, B. 2001 Self similar concentration profiles in buoyant mixing of miscible fluids in a vertical tube. Phys. Fluids 13, 3097.CrossRefGoogle Scholar
11. Debacq, M., Hulin, J. P., Salin, D., Perrin, B. & Hinch, E. J. 2003 Buoyant mixing of miscible fluids of varying viscosities in vertical tube. Phys. Fluids 15, 3846.CrossRefGoogle Scholar
12. Didden, N. & Maxworthy, T. 1982 The viscous spreading of plane and axisymmetric gravity currents. J. Fluid Mech. 121, 27–42.CrossRefGoogle Scholar
13. Ern, P., Charru, F. & Luchini, P. 2003 Stability analysis of a shear flow with strongly stratified viscosity. J. Fluid Mech. 496, 295–312.CrossRefGoogle Scholar
14. Govindarajan, R. 2004 Effect of miscibility on the linear instability of two-fluid channel flow. Intl J. Multiphase Flow 30, 1177–1192.CrossRefGoogle Scholar
15. Goyal, N. & Meiburg, E. 2006 Miscible displacements in Hele-Shaw cells: two-dimensional base states and their linear stability. J. Fluid Mech. 558, 329–355.CrossRefGoogle Scholar
16. Goyal, N., Pichler, H. & Meiburg, E. 2007 Variable density, miscible displacements in a vertical Hele-Shaw cell: linear stability. J. Fluid Mech. 584, 357–372.CrossRefGoogle Scholar
17. Hallez, Y. & Magnaudet, J. 2008 Effects of channel geometry on buoyancy-driven mixing. Phys. Fluids 20, 053306.CrossRefGoogle Scholar
18. Hinch, E. J. 1984 A note on the mechanism of the instability at the interface between two shearing fluids. J. Fluid Mech. 114, 463–465.CrossRefGoogle Scholar
19. Hormozi, S., Wielage-Burchard, K. & Frigaard, I. A. 2011 Entry, start up and stability effects in visco-plastically lubricated pipe flows. J. Fluid Mech. 673, 432–467.CrossRefGoogle Scholar
20. Hoult, D. P 1972 Oil spreading in the sea. Annu. Rev. Fluid Mech. 4, 341–368.CrossRefGoogle Scholar
21. Huppert, H. E. & Hallworth, M. A. 2007 Bi-directional flows in constrained systems. J. Fluid Mech. 578, 95–112.CrossRefGoogle Scholar
22. John, M. O., Oliveira, R. M., Heussler, F. H. C. & Meiburg, E. 2011 Viscously unstable miscible displacements in horizontal Hele-Shaw cells: the effect of density variations. J. Fluid Mech., doi:10.1017/jfm.2012.18.CrossRefGoogle Scholar
23. Joseph, D. D. & Renardy, Y. Y. 1993 Fundamentals of Two-Fluid Dynamics. Part 2: Lubricated Transport, Drops and Miscible Liquids, Interdisciplinary Applied Mathematics Series , vol. 4. Springer.Google Scholar
24. Lajeunesse, E., Martin, J., Rakotomalala, N., Salin, D. & Yortsos, Y. 1999 Miscible displacement in a Hele-Shaw cell at high rates. J. Fluid Mech. 398, 299–319.CrossRefGoogle Scholar
25. Lajeunesse, E., Martin, J, Rakotomalala, N. & Salin, D. 1997 3D instability of miscible displacements in a Hele-Shaw cell. Phys. Rev. Lett. 79, 5254–5257.CrossRefGoogle Scholar
26. Mehidi, N. & Amatousse, N. 2009 Modélisation d’un écoulement coaxial en conduite circulaire de deux fluides visqueux. C. R. Mecanique 337, 112–118.Google Scholar
27. d’Olce, M. 2008 Instabilités de cisaillement dans lécoulement concentrique de deux fluides miscibles. PhD thesis, Universite Pierre et Marie Curie, Orsay, France.Google Scholar
28. d’Olce, M., Martin, J., Rakotomalala, N. & Salin, D. 2008 Pearl and mushroom instability patterns in two miscible fluids core annular flows. Phys. Fluids 20, 024104.CrossRefGoogle Scholar
29. d’Olce, M., Martin, J., Rakotomalala, N., Salin, D. & Talon, L. 2009 Convective/absolute instability in miscible core–annular flow. Part 1. Experiments. J. Fluid Mech. 618, 305–322.CrossRefGoogle Scholar
30. Oliveira, R. M. & Meiburg, E. 2011 Miscible displacements in Hele-Shaw cells: three-dimensional Navier–Stokes simulations. J. Fluid Mech. 687, 431–460.CrossRefGoogle Scholar
31. Petitjeans, P. & Maxworthy, T. 1996 Miscible displacements in capillary tubes. Part 1. Experiments. J. Fluid Mech. 326, 37–56.CrossRefGoogle Scholar
32. Rakotomalala, N., Salin, D. & Watzky, P. 1997 Miscible displacement between two parallel plates: BGK lattice gas simulations. J. Fluid Mech. 338, 277–297.CrossRefGoogle Scholar
33. Ranganathan, T. & Govindarajan, R. 2001 Stabilization and destabilization of channel flow by location of viscosity-stratified fluid layer. Phys. Fluids 13, 1–3.CrossRefGoogle Scholar
34. Ruyer-Quil, C. & Manneville, P. 2000 Improved modelling of flows down inclined planes. Eur. Phys. J. B 15, 357–369.Google Scholar
35. Sahu, K. C., Ding, H., Valluri, P. & Matar, O. K. 2009a Linear stability analysis and numerical simulation of miscible two-layer channel flow. Phys. Fluids 21, 042104.CrossRefGoogle Scholar
36. Sahu, K. C., Ding, H., Valluri, P. & Matar, O. K. 2009b Pressure-driven miscible two-fluid channel flow with density gradients. Phys. Fluids 21, 043603.CrossRefGoogle Scholar
37. Sahu, K. C. & Vanka, S. P. 2011 A multiphase lattice Boltzmann study of buoyancy-induced mixing in a tilted channel. Comput. Fluids 50, 199–215.CrossRefGoogle Scholar
38. Selvam, B., Merk, S., Govindarajan, R. & Meiburg, E. 2007 Stability of miscible core–annular flow with viscosity stratification. J. Fluid Mech. 492, 23–49.CrossRefGoogle Scholar
39. Selvam, B., Talon, L., Leshafft, L. & Meiburg, E. 2009 Convective/absolute instability in miscible core–annular flow. Part 2. Numerical simulations and nonlinear global modes. J. Fluid Mech. 618, 323–348.CrossRefGoogle Scholar
40. Seon, T., Hulin, J.-P., Salin, D., Perrin, B. & Hinch, E. J. 2004 Buoyant mixing of miscible fluids in tilted tubes. Phys. Fluids 16, L103–L106.CrossRefGoogle Scholar
41. Seon, T., Hulin, J.-P., Salin, D., Perrin, B. & Hinch, E. J. 2005 Buoyancy driven miscible front dynamics in tilted tubes. Phys. Fluids 17, 031702.CrossRefGoogle Scholar
42. Seon, T., Hulin, J.-P., Salin, D., Perrin, B. & Hinch, E. J. 2006 Laser-induced fluorescence measurements of buoyancy driven mixing in tilted tubes. Phys. Fluids 18, 041701.CrossRefGoogle Scholar
43. Seon, T., Znaien, J., Salin, D., Hulin, J.-P., Hinch, E. J. & Perrin, B. 2007a Front dynamics and macroscopic diffusion in buoyant mixing in a tilted tube. Phys. Fluids 19, 125105.CrossRefGoogle Scholar
44. Seon, T., Znaien, J., Salin, D., Hulin, J.-P., Hinch, E. J. & Perrin, B. 2007b Transient buoyancy-driven front dynamics in nearly horizontal tubes. Phys. Fluids 19, 123603.CrossRefGoogle Scholar
45. Shin, J. O., Dalziel, S. B. & Linden, P. F. 2004 Gravity currents produced by lock exchange. J. Fluid. Mech. 521, 1–34.CrossRefGoogle Scholar
46. Simpson, J. E. 1997 Gravity Currents in the Environment and the Laboratory, 2nd edn. Cambridge University Press.Google Scholar
47. Stevenson, D. S. & Blake, S. 1998 Modelling the dynamics and thermodynamics of volcanic degassing. Bull. Volcanol. 38 (4), 307–317.CrossRefGoogle Scholar
48. Taghavi, S. M. 2011 From displacement to mixing in a slightly inclined duct. PhD thesis, University of British Columbia, Vancouver, Canada.Google Scholar
49. Taghavi, S. M., Seon, T., Martinez, D. M. & Frigaard, I. A. 2009 Buoyancy-dominated displacement flows in near-horizontal channels: the viscous limit. J. Fluid. Mech. 639, 1–35.CrossRefGoogle Scholar
50. Taghavi, S. M., Seon, T., Martinez, D. M. & Frigaard, I. A. 2010 Influence of an imposed flow on the stability of a gravity current in a near horizontal duct. Phys. Fluids 22, 031702.CrossRefGoogle Scholar
51. Taghavi, S. M., Seon, T., Wielage-Burchard, K., Martinez, D. M. & Frigaard, I. A. 2011 Stationary residual layers in buoyant Newtonian displacement flows. Phys. Fluids 23, 044105.CrossRefGoogle Scholar
52. Yang, Z. & Yortsos, Y. C. 1997 Asymptotic solutions of miscible displacements in geometries of large aspect ratio. Phys. Fluids 9, 286–298.CrossRefGoogle Scholar
53. Yee, H. C., Warming, R. F. & Harten, A. 1985 Implicit total variation diminishing (TVD) schemes for steady-state calculations. J. Comput. Phys. 57, 327–360.CrossRefGoogle Scholar
54. Yih, C.-S. 1967 Instability due to viscosity stratification. J. Fluid Mech. 27, 337–352.CrossRefGoogle Scholar