Article contents
Microlayer dynamics of hydrodynamically interacting vapour bubbles in flow boiling
Published online by Cambridge University Press: 10 March 2023
Abstract
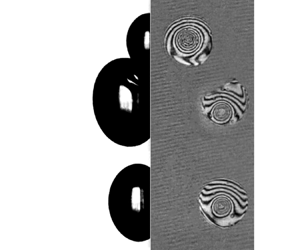
Nucleate boiling, a ubiquitous heat transfer mode, involves multiple vapour bubble nucleations on the heater surface and offers high heat transfer coefficients. The bubble growth process on a heating substrate involves the formation of microlayer, a thin liquid film trapped between the growing bubble and the heating substrate, and contributes to the bubble growth phenomenon through evaporation. Microlayer dynamics for a single bubble have been widely investigated in the pool and flow boiling conditions. However, the literature on multiple bubbles interactions and their associated microlayer information is scarce. Notably, in the case of flow boiling, where the microlayer dynamics are not symmetric due to the bubble's movement, the bubbles’ interaction and its influence on associated microlayer dynamics have never been reported. Therefore, microlayer and bubble dynamics in multiple interactions have been investigated experimentally using simultaneous application of thin-film interferometry and high-speed videography techniques in flow boiling with water as the working fluid. Our experimental investigation revealed that the secondary nucleation could cause a reduction in lift-off time and may assist or hinder the movement of the first bubble. The experimental results also demonstrated that the secondary nucleation could deplete the microlayer of the first bubble hydrodynamically even when the bubbles are far apart. Furthermore, it has been found that the microlayer depletion rate depends on the growth rate and the location of the secondary nucleation. Hence, this experimental study emphasises the need to consider the interaction of bubbles while modelling boiling flows to avoid overestimating the contribution of microlayer evaporation.
JFM classification
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2023. Published by Cambridge University Press
References
- 16
- Cited by



