Hostname: page-component-586b7cd67f-2plfb Total loading time: 0 Render date: 2024-11-24T08:40:45.187Z Has data issue: false hasContentIssue false
  • English
  • Français

Method of curved-shock characteristics with application to inverse design of supersonic flowfields

Published online by Cambridge University Press:  14 June 2021

Chongguang Shi Open the ORCID record for Chongguang Shi [Opens in a new window] ,
Chengxiang Zhu Open the ORCID record for Chengxiang Zhu [Opens in a new window] ,
Yancheng You Open the ORCID record for Yancheng You [Opens in a new window]  and
Guangsheng Zhu
Chongguang Shi
Affiliation:
School of Aerospace Engineering, Xiamen University, Xiamen, Fujian361005, PR China
Chengxiang Zhu
Affiliation:
School of Aerospace Engineering, Xiamen University, Xiamen, Fujian361005, PR China
Yancheng You*
Affiliation:
School of Aerospace Engineering, Xiamen University, Xiamen, Fujian361005, PR China
Guangsheng Zhu
Affiliation:
School of Aerospace Engineering, Xiamen University, Xiamen, Fujian361005, PR China
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

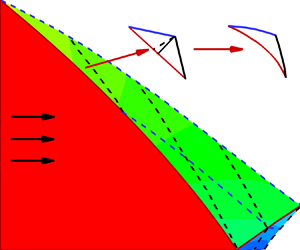
This paper describes the development of a method of curved-shock characteristics based on curved shock theory. The proposed method is applied to supersonic flowfield calculations and inverse design in planar/axisymmetric, external/internal and uniform/non-uniform flows. The main idea is to determine the gradients of the pressure and flow deflection angle in the streamline-characteristic coordinates. With the acquired derivatives, the flow parameters of the post-shock flowfield can be quickly identified. Compared with the method of characteristics, the gradient information enhances the computational efficiency and accuracy of the method of curved-shock characteristics. This makes the method of curved-shock characteristics more effective, accurate and robust than the conventional method of characteristics. Explicit equations in the form of gradients are derived along the streamlines and characteristics. Several supersonic flowfields are solved using the method of curved-shock characteristics, and the results show that the proposed method requires less computational resources, by an order of magnitude, than the method of characteristics while achieving superior accuracy. Additionally, the proposed method is applied to the inverse design of internal flows. A series of planar and axisymmetric flowfields with centre-bodies are solved under the condition that the shock curves are given. The accuracy and efficiency of the method of curved-shock characteristics make it a good candidate for the inverse design of planar/axisymmetric supersonic flowfields.

JFM classification

Aerodynamics: High-speed flow Compressible Flows: Shock waves Mathematical Foundations: Computational methods
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 920 , 10 August 2021 , A36
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Ben-Dor, G. 2007 Shock Wave Reflection Phenomena. Springer.Google Scholar
Chu, C.W. & Nadir, S. 1976 Unsteady two-dimensional subsonic, transonic, and supersonic flow calculations by the method of characteristics. Proc. Fifth Intl Conf. Numer. Meth. Fluid Dyn. 59, 145–152.Google Scholar
Crocco, L. 1937 Singolarita della corrente gassosa iperacustica nell'interno di una prora a diedro. Cong. Unione Mat. Ital. 15, 597–615.Google Scholar
Deiterding, R. 2011 Block-structured adaptive mesh refinement - theory, implementation and application. In European Series in Applied and Industrial Mathematics (ESAIM): Proceedings, vol. 34, pp. 97–150.Google Scholar
Emanuel, G. 1988 Shock wave derivatives. Phys. Fluids 31, 3625–3633.CrossRefGoogle Scholar
Emanuel, G. 2012 Shock Wave Dynamics: Derivatives and Related Topics. CRC Press.CrossRefGoogle Scholar
Emanuel, G. 2018 Analytical extension of curved shock theory. Shock Waves 28, 417–425.CrossRefGoogle Scholar
Filippi, A.A. & Skews, B.W. 2018 Streamlines behind curved shock waves in axisymmetric flow fields. Shock Waves 28, 785–793.CrossRefGoogle Scholar
Gerber, N. & Bartos, J.M. 1960 Calculation of flow-variable gradients behind curved shock waves. J. Aerosp. Sci. 27, 958–959.CrossRefGoogle Scholar
Griffith, W.C. 1981 Shock waves. J. Fluid Mech. 106, 81–101.CrossRefGoogle Scholar
Harrop, R. 1953 An introduction to the method of characteristics. Aircraft Engng Aerosp. Technol. 25, 277–285.CrossRefGoogle Scholar
Hoffman, J.D. 1973 Accuracy studies of the numerical method of characteristics for axisymmetric, steady supersonic flows. J. Comput. Phys. 11 (2), 210–239.CrossRefGoogle Scholar
Huang, T.L., Yue, L.J., Ma, S.H., Zhang, Q.F., Zhang, P. & Chang, X.Y. 2020 Numerical investigation on flow nonuniformity-induced hysteresis in scramjet isolator. Chin. J. Aeronaut. 33, 3176–3188.CrossRefGoogle Scholar
Kanwal, R.P. 1959 On curved shock waves in three-dimensional gas flows. Q. Appl. Maths 16, 361–372.CrossRefGoogle Scholar
Laurence, S., Parziale, N. & Deiterding, R. 2012 Dynamical separation of spherical bodies in supersonic flow. J. Fluid Mech. 713, 159–182.CrossRefGoogle Scholar
Li, Y.Q., Shi, C.G., Zheng, X.G. & You, Y.C. 2019 Dual waverider to integrate external and internal flows. J. Aircraft 57, 1–12.Google Scholar
Mach, E. 2013 The Science of Mechanics. Cambridge Library Collection - Physical Sciences. Cambridge University Press.CrossRefGoogle Scholar
Mölder, S. 1972 Polar streamline directions at the triple point of mach interaction of shock waves. Can. Aeronaut. Space Inst. Trans. 5, 88–89.Google Scholar
Mölder, S. 2016 Curved shock theory. Shock Waves 26 (4), 337.CrossRefGoogle Scholar
Mölder, S. 2017 Flow behind concave shock waves. Shock Waves 27 (5), 721–730.CrossRefGoogle Scholar
von Neumann, J. 1943 Oblique reflection of shock waves. In John von Neumann Collected Works, pp. 238–299. Pergamon Press.Google Scholar
Qin, L., Qilong, G., Dong, S., Pengxin, L. & Hanxin, Z. 2017 A fourth-order symmetric weno scheme with improved performance by new linear and nonlinear optimizations. J. Sci. Comput. 71, 109–143.Google Scholar
Rylov, A.I. 1990 On the impossibility of regular reflection of a steady-state shock wave from the axis of symmetry. J. Appl. Maths Mech. 54, 201–203.CrossRefGoogle Scholar
Shi, C.G., Han, W.Q., Deiterding, R., Zhu, C.X. & You, Y.C. 2020 Second-order curved shock theory. J. Fluid Mech. 891, A21.CrossRefGoogle Scholar
Tao, Y., Fan, X.Q. & Zhao, Y.L. 2014 Viscous effects of shock reflection hysteresis in steady supersonic flows. J. Fluid Mech. 759, 134–148.CrossRefGoogle Scholar
Thomas, T.Y. 1947 On curved shock waves. J. Math. Phys. 26 (14), 62–68.CrossRefGoogle Scholar
Thomas, T.Y. 1949 The consistency relations for shock waves. Stud. Appl. Maths 28, 62–90.Google Scholar
Truesdell, C. 1952 On curved shocks in steady plane flow of an ideal fluid. J. Aerosp. Sci. 19, 826–828.Google Scholar
Wang, Z. 2007 High-order methods for the Euler and Navier–Stokes equations on unstructured grids. Prog. Aerosp. Sci. 43, 1–41.CrossRefGoogle Scholar
You, Y.C. & Liang, D.W. 2009 Design concept of three-dimensional section controllable internal waverider hypersonic inlet. Sci. China Ser. E Technol. Sci. 52, 2017–2028.CrossRefGoogle Scholar
Zhang, K. 2020 Hypersonic Curved Compression Inlet and Its Inverse Design. Springer.CrossRefGoogle Scholar
Zheng, X.G., Hu, Z.C., Li, Y.Q., Zhu, C.X., You, Y.C. & Song, W.J. 2020 Local-turning osculating cones method for waverider design. AIAA J. 58, 1–15.Google Scholar