Hostname: page-component-586b7cd67f-g8jcs Total loading time: 0 Render date: 2024-11-24T11:29:25.516Z Has data issue: false hasContentIssue false
  • English
  • Français

Mechanism of reduction of aeroacoustic sound by porous material: comparative study of microscopic and macroscopic models

Published online by Cambridge University Press:  27 October 2021

Yasunori Sato  and
Yuji Hattori Open the ORCID record for Yuji Hattori [Opens in a new window]
Yasunori Sato
Affiliation:
Institute of Fluid Science, Tohoku University, Sendai980-8577, Japan Graduate School of Information Sciences, Tohoku University, Sendai980-8579, Japan
Yuji Hattori*
Affiliation:
Institute of Fluid Science, Tohoku University, Sendai980-8577, Japan
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

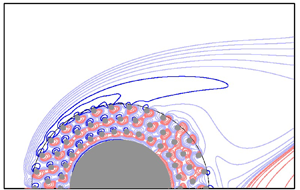
The effects of porous material on the aeroacoustic sound generated in a two-dimensional low-Reynolds-number flow ($Re=150$) past a circular cylinder are studied by direct numerical simulation in which the acoustic waves of small amplitudes are obtained directly as a solution to the compressible Navier–Stokes equations. Two models are introduced for the porous material: the microscopic model, in which the porous material is a collection of small cylinders, and the macroscopic model, in which the porous material is continuum characterized by permeability. The corrected volume penalization method is used to deal with the core cylinder, the small cylinders and the porous material. In the microscopic model, significant reduction of the aeroacoustic sound is found depending on the parameters; the maximum reduction of $24.4$ dB from the case of a bare cylinder is obtained. The results obtained for the modified macroscopic model are in good agreement with those obtained for the microscopic model converted by the theory of homogenization, which establishes that the microscopic and macroscopic models are consistent and valid. The detailed mechanism of sound reduction is elucidated. The presence of a fluid region between the porous material and the core cylinder is important for sound reduction. When the sound is strongly reduced, the pressure field behind the cylinder becomes nearly uniform with a high value to stabilize the shear layer in the wake; as a result, the vortex shedding behind the cylinder is delayed to the far wake to suppress the unsteady vortex motion near the cylinder, which is responsible for the aeroacoustic sound.

JFM classification

Acoustics: Aeroacoustics Acoustics: Noise control Low-Reynolds-number flows: Porous media
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 929 , 25 December 2021 , A34
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Ali, M.S.M., Zaki, S.A., Ismail, M.H., Muhamad, S. & Mahzan, M.I. 2013 Aeolian tones radiated from flow over bluff bodies. Open Mech. J. 7, 48–57.Google Scholar
Auriault, J.-L., Boutin, C. & Geindreau, C. 2009 Homogenization of Coupled Phenomena in Heterogeneous Media, Chap. 10. ISTE Ltd and John Wiley & Sons Inc.CrossRefGoogle Scholar
Barré, S., Bogey, C. & Bailly, C. 2008 Direct simulation of isolated elliptic vortices and of their radiated noise. Theor. Comput. Fluid Dyn. 22, 65–82.CrossRefGoogle Scholar
Berdichevsky, A.L. & Cai, Z. 1993 Preform permeability predictions by self-consistent method and finite element simulation. Polym. Compos. 14, 132–143.CrossRefGoogle Scholar
Blevins, R.D. 1990 Flow-Induced Vibration, Chap. 3. Van Nostrand Reinhold.Google Scholar
Boutin, C. 2000 Study of permeability by periodic and self-consistent homogenisation. Eur. J. Mech. (A/Solids) 19, 603–632.CrossRefGoogle Scholar
Breugem, W.-P., van Dijk, V. & Delfos, R. 2015 Flows through real porous media: X-ray computed tomography, experiments, and numerical simulations. Trans. ASME J. Fluids Engng 136, 040902.CrossRefGoogle Scholar
Cervera, F., Sanchis, L., Sánchez-Pérez, J.V., Martínez-Sala, R., Rubio, C., Meseguer, F., López, C., Caballero, D. & Sánchez-Dehesa, J. 2002 Refractive acoustic devices for airborne sound. Phys. Rev. Lett. 88, 023902.CrossRefGoogle ScholarPubMed
Colonius, T. & Lele, S.K. 2004 Computational aeroacoustics: progress on nonlinear problems of sound generation. Prog. Aerosp. Sci. 40, 345–416.CrossRefGoogle Scholar
Delfs, J., Faßmann, B., Lippitz, N., Lummer, M., Mößner, M., Müller, L., Rurkowska, K. & Uphoff, S. 2014 SFB 880: aeroacoustic research for low noise take-off and landing. CEAS Aeronaut. J. 5, 403–417.CrossRefGoogle Scholar
Fellah, Z.E.A. & Depollier, C. 2000 Transient acoustic wave propagation in rigid porous media: a time-domain approach. J. Acoust. Soc. Am. 107, 683–688.CrossRefGoogle ScholarPubMed
Ffowcs Williams, J.E. & Hawkings, D.L. 1969 Sound generation by turbulence and surfaces in arbitrary motion. Phil. Trans. R. Soc. Lond. A 264, 321–342.Google Scholar
Freund, J.B., Lele, S.K. & Moin, P. 2000 Direct numerical simulation of a Mach 1.92 turbulent jet and its sound field. AIAA J. 38, 3–31.CrossRefGoogle Scholar
Gao, K., van Dommelen, J.A.W., Goransson, P. & Geers, M.G.D. 2016 Computational homogenization of sound propagation in a deformable porous material including microscopic viscous-thermal effects. J. Sound Vib. 365, 119–133.CrossRefGoogle Scholar
Geyer, T. & Sarradj, E. 2016 Circular cylinders with soft porous cover for flow noise reduction. Exp. Fluids 57, 30.CrossRefGoogle Scholar
Geyer, T., Sarradj, E. & Fritzsche, C. 2010 Porous airfoils: noise reduction and boundary layer effects. Intl J. Aeroacoust. 9, 787–820.CrossRefGoogle Scholar
Giret, J.-C. & Sengissen, A. 2015 Noise source analysis of a rod-airfoil configuration using unstructured large-eddy simulation. AIAA J. 53, 1062–1077.CrossRefGoogle Scholar
Gloerfelt, X., Bailly, C. & Juvé, D. 2003 Direct computation of the noise radiated by a subsonic cavity flow and application of integral methods. J. Sound Vib. 266, 119–146.CrossRefGoogle Scholar
Hattori, Y. & Komatsu, R. 2017 Mechanism of aeroacoustic sound generation and reduction in a flow past oscillating and fixed cylinders. J. Fluid Mech. 832, 241–268.CrossRefGoogle Scholar
Hattori, Y. & Llewellyn Smith, S.G. 2002 Axisymmetric acoustic scattering by vortices. J. Fluid Mech. 473, 275–294.CrossRefGoogle Scholar
Herr, M., Rossignol, K.-S., Delfs, J., Mößner, M. & Lippitz, N. 2014 Specification of porous materials for low noise trailing edge applications. In 20th AIAA/CEAS Aeroacoustics Conference, Atlanta, GA, AIAA Paper 2014–3041.Google Scholar
Hwang, W.R. & Advani, S.G. 2010 Numerical simulations of Stokes–Brinkman equations for permeability prediction of dual scale fibrous porous media. Phys. Fluids 22, 113101.CrossRefGoogle Scholar
Inoue, O. & Hatakeyama, N. 2002 Sound generation by a two-dimensional circular cylinder in a uniform flow. J. Fluid Mech. 471, 285–314.CrossRefGoogle Scholar
Inoue, O. & Hattori, Y. 1999 Sound generation by shock-vortex interactions. J. Fluid Mech. 380, 81–116.CrossRefGoogle Scholar
Inoue, O., Hattori, Y. & Sasaki, T. 2000 Sound generation by coaxial collision of two vortex rings. J. Fluid Mech. 424, 327–365.CrossRefGoogle Scholar
Iwagami, S., Tabata, R., Kobayashi, T., Hattori, Y. & Takahashi, K. 2021 Numerical study on edge tone with compressible direct numerical simulation: sound intensity and jet motion. Intl J. Aeroacoust. 20, 283–316.CrossRefGoogle Scholar
Komatsu, R., Iwakami, W. & Hattori, Y. 2016 Direct numerical simulation of aeroacoustic sound by volume penalization method. Comput. Fluids 130, 24–36.CrossRefGoogle Scholar
Lele, S.K. 1992 Compact finite-difference schemes with spectral-like resolution. J. Comput. Phys. 103, 16–42.CrossRefGoogle Scholar
Liu, H., Azarpeyvand, M., Wei, J. & Qu, Z. 2015 Tandem cylinder aerodynamic sound control using porous coating. J. Sound Vib. 334, 190–201.CrossRefGoogle Scholar
Liu, Q. & Vasilyev, O.V. 2007 A Brinkman penalization method for compressible flows in complex geometries. J. Comput. Phys. 227, 946–966.CrossRefGoogle Scholar
Lopez Penha, D.J., Geurts, B.J., Stolz, S. & Nordlund, M. 2011 Computing the apparent permeability of an array of staggered square rods using volume-penalization. Comput. Fluids 51, 157–173.CrossRefGoogle Scholar
Matsumura, Y. & Jackson, T.L. 2014 Numerical simulation of fluid flow through random packs of polydisperse cylinders. Phys. Fluids 26, 123302.CrossRefGoogle Scholar
Matsumura, Y., Jenne, D. & Jackson, T.L. 2015 Numerical simulation of fluid flow through random packs of ellipses. Phys. Fluids 27, 023301.CrossRefGoogle Scholar
Mitchell, B.E., Lele, S.K. & Moin, P. 1995 Direct computation of the sound from a compressible co-rotating vortex pair. J. Fluid Mech. 285, 181–202.CrossRefGoogle Scholar
Mitchell, B.E., Lele, S.K. & Moin, P. 1999 Direct computation of the sound generated by vortex pairing in an axisymmetric jet. J. Fluid Mech. 383, 113–142.CrossRefGoogle Scholar
Müller, B. 2008 High order numerical simulation of aeolian tones. Comput. Fluids 37, 450–462.CrossRefGoogle Scholar
Naito, H. & Fukagata, K. 2012 Numerical simulation of flow around a circular cylinder having porous surface. Phys. Fluids 24, 117102.CrossRefGoogle Scholar
Nakashima, Y. 2008 Sound generation by head-on and oblique collisions of two vortex rings. Phys. Fluids 20, 056102.CrossRefGoogle Scholar
Nishimura, M. & Goto, T. 2010 Aerodynamic noise reduction by pile fabrics. Fluid Dyn. Res. 42, 015003.CrossRefGoogle Scholar
Perrot, C., Chevillotte, F. & Panneton, R. 2007 Dynamic viscous permeability of an opencell aluminum foam: computations versus experiments. J. Appl. Phys. 103, 024909.CrossRefGoogle Scholar
Poinsot, T.J. & Lele, S.K. 1992 Boundary-conditions for direct simulations of compressible viscous flows. J. Comput. Phys. 101, 104–129.CrossRefGoogle Scholar
Rajani, B.N., Kandasamy, A. & Majumdar, S. 2009 Numerical simulation of laminar flow past a circular cylinder. Appl. Math. Model. 33, 1228–1247.CrossRefGoogle Scholar
Sueki, T., Takaishi, T., Ikeda, M. & Arai, N. 2010 Application of porous material to reduce aerodynamic sound from bluff bodies. Fluid Dyn. Res. 42, 015004.CrossRefGoogle Scholar
Tsutahara, M., Kataoka, T., Shikata, K. & Takada, N. 2008 New model and scheme for compressible fluids of the finite difference lattice Boltzmann method and direct simulations of aerodynamic sound. Comput. Fluids 37, 79–89.CrossRefGoogle Scholar
Wang, M., Freund, J.B. & Lele, S.K. 2006 Computational prediction of flow-generated sound. Annu. Rev. Fluid Mech. 38, 483–512.CrossRefGoogle Scholar
Wong, H.Y. & Kokkalis, A. 1982 A comparative study of three aerodynamic devices for suppressing vortex-induced oscillation. J. Wind Engng Ind. Aerod. 10, 21–29.CrossRefGoogle Scholar
Xu, Y., Zheng, Z.C. & Wilson, D.K. 2010 Simulation of turbulent wind noise reduction by porous windscreens using high-order schemes. J. Comput. Acoust. 18, 321–334.CrossRefGoogle Scholar