Article contents
Marginally stable and turbulent boundary layers in low-curvature Taylor–Couette flow
Published online by Cambridge University Press: 15 February 2017
Abstract
Marginal stability arguments are used to describe the rotation number dependence of torque in Taylor–Couette (TC) flow for radius ratios 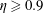 $\unicode[STIX]{x1D702}\geqslant 0.9$ and shear Reynolds number
$\unicode[STIX]{x1D702}\geqslant 0.9$ and shear Reynolds number 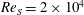 $\mathit{Re}_{S}=2\times 10^{4}$. With an approximate representation of the mean profile by piecewise linear functions, characterised by the boundary-layer thicknesses at the inner and outer cylinder and the angular momentum in the centre, profiles and torques are extracted from the requirement that the boundary layers represent marginally stable TC subsystems and that the torque at the inner and outer cylinder coincide. This model then explains the broad shoulder in the torque as a function of rotation number near
$\mathit{Re}_{S}=2\times 10^{4}$. With an approximate representation of the mean profile by piecewise linear functions, characterised by the boundary-layer thicknesses at the inner and outer cylinder and the angular momentum in the centre, profiles and torques are extracted from the requirement that the boundary layers represent marginally stable TC subsystems and that the torque at the inner and outer cylinder coincide. This model then explains the broad shoulder in the torque as a function of rotation number near 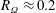 $R_{\unicode[STIX]{x1D6FA}}\approx 0.2$. For rotation numbers
$R_{\unicode[STIX]{x1D6FA}}\approx 0.2$. For rotation numbers 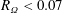 $R_{\unicode[STIX]{x1D6FA}}<0.07$ the TC stability conditions predict boundary layers in which the shear Reynolds numbers are very large. Assuming that the TC instability is bypassed by some shear instability, a second narrower maximum in torque appears, in very good agreement with numerical simulations. The results show that marginal stability theory, despite its shortcomings in other cases, can explain quantitatively the non-monotonic torque variation with rotation number for both the broad maximum as well as the narrow maximum.
$R_{\unicode[STIX]{x1D6FA}}<0.07$ the TC stability conditions predict boundary layers in which the shear Reynolds numbers are very large. Assuming that the TC instability is bypassed by some shear instability, a second narrower maximum in torque appears, in very good agreement with numerical simulations. The results show that marginal stability theory, despite its shortcomings in other cases, can explain quantitatively the non-monotonic torque variation with rotation number for both the broad maximum as well as the narrow maximum.
JFM classification
- Type
- Papers
- Information
- Copyright
- © 2017 Cambridge University Press
References
- 9
- Cited by



