Hostname: page-component-586b7cd67f-dsjbd Total loading time: 0 Render date: 2024-11-28T15:51:26.570Z Has data issue: false hasContentIssue false
  • English
  • Français

Longitudinal development of flow-separation lines on slender bodies in translation

Published online by Cambridge University Press:  05 January 2018

S.-K. Lee Open the ORCID record for S.-K. Lee [Opens in a new window]
S.-K. Lee*
Affiliation:
Defence Science and Technology Group, Melbourne, VIC 3207, Australia Australian Maritime College, University of Tasmania, Launceston, TAS 7250, Australia
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

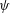This paper examines flow-separation lines on axisymmetric bodies with tapered tails, where the separating flow takes into account the effect of local body radius $r(x)$, incidence angle $\unicode[STIX]{x1D713}$ and the body-length Reynolds number $\mathit{Re}_{L}$. The flow is interpreted as a transient problem which relates the longitudinal distance $x$ to time 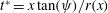$t^{\ast }=x\,\text{tan}(\unicode[STIX]{x1D713})/r(x)$, similar to the approach of Jeans & Holloway (J. Aircraft, vol. 47 (6), 2010, pp. 2177–2183) on scaling separation lines. The windward and leeward sides correspond to the body azimuth angles 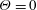$\unicode[STIX]{x1D6E9}=0$ and 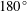$180^{\circ }$, respectively. From China-clay flow visualisation on axisymmetric bodies and from a literature review of slender-body flows, the present study shows three findings. (i) The time scale 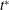$t^{\ast }$ provides a collapse of the separation-line data, 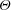$\unicode[STIX]{x1D6E9}$, for incidence angles between 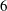$6$ and 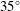$35^{\circ }$, where the data fall on a power law $\unicode[STIX]{x1D6E9}\sim (t^{\ast })^{k}$. (ii) The data suggest that the separation rate 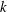$k$ is independent of the Reynolds number over the range 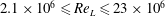$2.1\times 10^{6}\leqslant \mathit{Re}_{L}\leqslant 23\times 10^{6}$; for a primary separation $k_{1}\simeq -0.190$, and for a secondary separation 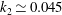$k_{2}\simeq 0.045$. (iii) The power-law curve fits trace the primary and secondary lines to a characteristic start time $t_{s}^{\ast }\simeq 1.5$.

JFM classification

Aerodynamics: Aerodynamics Wakes/Jets: Separated flows Wakes/Jets: Wakes/Jets
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 837 , 25 February 2018 , pp. 627 - 639
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Allen, H. J.1949 Estimation of the forces and moments acting on inclined bodies of revolution of high fineness ratio. Tech. Rep. NACA-RM-A9I26. National Advisory Committee for Aeronautics, Washington.Google Scholar
Ashok, A., Van Buren, T. & Smits, A. J. 2015 Asymmetries in the wake of a submarine model in pitch. J. Fluid Mech. 774, 416–442.Google Scholar
Chesnakas, C. J. & Simpson, R. L. 1997 Detailed investigation of the three-dimensional separation about a 6:1 prolate spheroid. AIAA J. 35 (6), 990–999.Google Scholar
Erm, L. P.2003 Calibration of the flow in the extended test section of the low-speed wind tunnel at DSTO. Tech. Rep. DSTO-TR-1384. Defence Science and Technology, Australia.Google Scholar
Groves, N. C., Huang, T. T. & Chang, M. S.1989 Geometric characteristics of DARPA SUBOFF models (DTRC model nos. 5470 and 5471). Tech. Rep. DTRC/SHD-1298-01. David Taylor Research Center, Bethesda.Google Scholar
Jeans, T. L. & Holloway, A. G. L. 2010 Flow-separation lines on axisymmetric bodies with tapered tails. J. Aircraft 47 (6), 2177–2183.10.2514/1.C031141Google Scholar
Jeans, T. L., Watt, G. D., Gerber, A. G., Holloway, A. G. L. & Baker, C. R. 2009 High-resolution Reynolds-averaged Navier–Stokes flow predictions over axisymmetric bodies with tapered tails. AIAA J. 47 (1), 19–32.Google Scholar
Jiang, F., Andersson, H. I., Gallardo, J. P. & Okulov, V. L. 2016 On the peculiar structure of a helical wake vortex behind an inclined prolate spheroid. J. Fluid Mech. 801, 1–12.Google Scholar
Lee, S.-K.2015 Topology model of the flow around a submarine hull form. Tech. Rep. DST-Group-TR-3177. Defence Science and Technology, Australia.Google Scholar
Mackay, M.2003 The standard submarine model: a survey of static hydrodynamic experiments and semiempirical predictions. Tech. Rep. TR-2003-079. Defence Research and Development Canada.Google Scholar
Sarpkaya, T. 1966 Separated flow about lifting bodies and impulsive flow about cylinders. AIAA J. 4 (3), 414–420.Google Scholar
Schindel, L. H. 1969 Effects of vortex separation on the lift distribution on bodies of elliptic cross section. J. Aircraft 6 (6), 537–543.Google Scholar
Tobak, M. & Peake, D. J. 1982 Topology of three-dimensional separated flows. Annu. Rev. Fluid Mech. 14, 61–85.Google Scholar
Van Randwijck, E. F. & Feldman, J. P.2000 Results of experiments with a segmented model to investigate the distribution of the hydrodynamics forces and moments on a streamlined body of revolution at an angle of attack or with a pitching angular velocity. Tech. Rep. NSWCCD-50-TR-2000/008. Naval Surface Warfare Center Carderock Division.Google Scholar
Wang, K. C. 1972 Separation patterns of boundary layer over an inclined body of revolution. AIAA J. 10 (8), 1044–1050.Google Scholar
Wetzel, T. G.1996 Unsteady flow over a 6:1 prolate spheroid. PhD thesis, Department of Aerospace and Ocean Engineering, Virginia Polytechnic Institute and State University, Blacksburg, Virginia.Google Scholar
Wetzel, T. G., Simpson, R. L. & Chesnakas, C. J. 1998 Measurement of three-dimensional crossflow separation. AIAA J. 36 (4), 557–564.Google Scholar