Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Daddi-Moussa-Ider, Abdallah
Löwen, Hartmut
and
Gekle, Stephan
2018.
Creeping motion of a solid particle inside a spherical elastic cavity.
The European Physical Journal E,
Vol. 41,
Issue. 9,
Hoell, Christian
Löwen, Hartmut
Menzel, Andreas M.
and
Daddi-Moussa-Ider, Abdallah
2019.
Creeping motion of a solid particle inside a spherical elastic cavity: II. Asymmetric motion.
The European Physical Journal E,
Vol. 42,
Issue. 7,
Shaik, Vaseem A.
and
Ardekani, Arezoo M.
2019.
Swimming sheet near a plane surfactant-laden interface.
Physical Review E,
Vol. 99,
Issue. 3,
K V S, Chaithanya
and
Thampi, Sumesh P
2020.
Deformation dynamics of an active compound particle in an imposed shear flow—a theoretical study.
Journal of Physics D: Applied Physics,
Vol. 53,
Issue. 31,
p.
314001.
Sprenger, Alexander R.
Shaik, Vaseem A.
Ardekani, Arezoo M.
Lisicki, Maciej
Mathijssen, Arnold J. T. M.
Guzmán-Lastra, Francisca
Löwen, Hartmut
Menzel, Andreas M.
and
Daddi-Moussa-Ider, Abdallah
2020.
Towards an analytical description of active microswimmers in clean and in surfactant-covered drops.
The European Physical Journal E,
Vol. 43,
Issue. 9,
Dewangan, Narendra K.
and
Conrad, Jacinta C.
2020.
Bacterial motility enhances adhesion to oil droplets.
Soft Matter,
Vol. 16,
Issue. 35,
p.
8237.
Kree, R.
and
Zippelius, A.
2021.
Controlled locomotion of a droplet propelled by an encapsulated squirmer.
The European Physical Journal E,
Vol. 44,
Issue. 1,
Pimenta, P. H. N.
and
Oliveira, T. F.
2021.
Study on the rheology of a dilute emulsion of surfactant-covered droplets using the level set and closest point methods.
Physics of Fluids,
Vol. 33,
Issue. 10,
Kree, R.
Rückert, L.
and
Zippelius, A.
2021.
Dynamics of a droplet driven by an internal active device.
Physical Review Fluids,
Vol. 6,
Issue. 3,
Kree, R.
and
Zippelius, A.
2022.
Mobilities of a drop and an encapsulated squirmer.
The European Physical Journal E,
Vol. 45,
Issue. 2,
Ardekani, Arezoo M.
2022.
Motile microorganisms in complex fluids.
Science Talks,
Vol. 3,
Issue. ,
p.
100048.
Della-Giustina, J.
Nganguia, H.
and
Demir, E.
2023.
Squirming with a backward-propelling cage.
Physics of Fluids,
Vol. 35,
Issue. 5,
K. V. S., Chaithanya
Singeetham, Pavan Kumar
and
Thampi, Sumesh P.
2023.
Active compound particles in a quadratic flow: hydrodynamics and morphology.
Soft Matter,
Vol. 19,
Issue. 41,
p.
7963.
Aymen, U.
Palaniappan, D.
Demir, E.
and
Nganguia, H.
2023.
Influence of heterogeneity or shape on the locomotion of a caged squirmer.
Journal of Fluid Mechanics,
Vol. 967,
Issue. ,
Hiruta, Yoshiki
and
Ishimoto, Kenta
2024.
Reciprocal microswimming in fluctuating and confined environments.
Physical Review Research,
Vol. 6,
Issue. 1,
Wang, Yufan
Wang, Yiting
Zhao, Hui
Li, Weifeng
and
Liu, Haifeng
2025.
An experimental investigation on role of turbulence modulation induced by thread structure in coaxial air blast atomization of a surfactant-laden jet.
Chemical Engineering Science,
Vol. 301,
Issue. ,
p.
120678.
Kawakami, Sho
and
Vlahovska, Petia M.
2025.
Migration and deformation of a droplet enclosing an active particle.
Journal of Fluid Mechanics,
Vol. 1007,
Issue. ,
Nganguia, Herve
Adegbuyi, Adedoyin
Uffenheimer, Matthew
and
Pak, On Shun
2025.
Squirming inside a liquid droplet with surface viscosities.
Physical Review Fluids,
Vol. 10,
Issue. 3,
 $(Pe_{s})$. Expanding the variables in
$(Pe_{s})$. Expanding the variables in 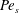 $Pe_{s}$, we solve the Stokes equations for the concentric configuration using Lamb’s general solution, while the dynamic equation for the stream function is solved in the bipolar coordinates for the eccentric configurations. For a two-mode squirmer inside a drop, the surfactant redistribution can either increase or decrease the magnitude of swimmer and drop velocities, depending on the value of the eccentricity. This was explained by analysing the influence of surfactant redistribution on the thrust and drag forces acting on the swimmer and the drop. The far-field representation of a surfactant-covered drop enclosing a pusher swimmer at its centre is a puller; the strength of this far field is reduced due to the surfactant redistribution. The advection of surfactant on the drop surface leads to a time-averaged propulsion of the drop and the time-reversible swimmer that it engulfs, thereby causing them to escape from the constraints of the scallop theorem. We quantified the range of parameters for which an eccentrically stable configuration can be achieved for a two-mode squirmer inside a clean drop. The surfactant redistribution shifts this eccentrically stable position towards the top surface of the drop, although this shift is small.
$Pe_{s}$, we solve the Stokes equations for the concentric configuration using Lamb’s general solution, while the dynamic equation for the stream function is solved in the bipolar coordinates for the eccentric configurations. For a two-mode squirmer inside a drop, the surfactant redistribution can either increase or decrease the magnitude of swimmer and drop velocities, depending on the value of the eccentricity. This was explained by analysing the influence of surfactant redistribution on the thrust and drag forces acting on the swimmer and the drop. The far-field representation of a surfactant-covered drop enclosing a pusher swimmer at its centre is a puller; the strength of this far field is reduced due to the surfactant redistribution. The advection of surfactant on the drop surface leads to a time-averaged propulsion of the drop and the time-reversible swimmer that it engulfs, thereby causing them to escape from the constraints of the scallop theorem. We quantified the range of parameters for which an eccentrically stable configuration can be achieved for a two-mode squirmer inside a clean drop. The surfactant redistribution shifts this eccentrically stable position towards the top surface of the drop, although this shift is small.