Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Fox, Andrew J.
Schneider, James W.
and
Khair, Aditya S.
2021.
Dynamics of a sphere in inertial shear flow between parallel walls.
Journal of Fluid Mechanics,
Vol. 915,
Issue. ,
Ekanayake, Nilanka I.K.
Berry, Joseph D.
and
Harvie, Dalton J.E.
2021.
Lift and drag forces acting on a particle moving in the presence of slip and shear near a wall.
Journal of Fluid Mechanics,
Vol. 915,
Issue. ,
Hammond, Paul S.
2021.
Will we ever wash our hands of lubrication theory?.
Physics of Fluids,
Vol. 33,
Issue. 8,
Chun, Byoungjin
and
Jung, Hyun Wook
2021.
Inertia- and shear-induced inhomogeneities in non-Brownian mono and bidisperse suspensions under wall-bounded linear shear flow.
Physics of Fluids,
Vol. 33,
Issue. 5,
Jing, Lu
Ottino, Julio M.
Lueptow, Richard M.
and
Umbanhowar, Paul B.
2021.
A unified description of gravity- and kinematics-induced segregation forces in dense granular flows.
Journal of Fluid Mechanics,
Vol. 925,
Issue. ,
Shi, Pengyu
Rzehak, Roland
Lucas, Dirk
and
Magnaudet, Jacques
2021.
Drag and lift forces on a rigid sphere immersed in a wall-bounded linear shear flow.
Physical Review Fluids,
Vol. 6,
Issue. 10,
Geonzon, Lester C.
Kobayashi, Motoyoshi
and
Adachi, Yasuhisa
2021.
Effect of shear flow on the hydrodynamic drag force of a spherical particle near a wall evaluated using optical tweezers and microfluidics.
Soft Matter,
Vol. 17,
Issue. 34,
p.
7914.
Qian, Yunzhou
Usher, Shane P.
Scales, Peter J.
Stickland, Anthony D.
and
Alexiadis, Alessio
2022.
Agglomeration Regimes of Particles under a Linear Laminar Flow: A Numerical Study.
Mathematics,
Vol. 10,
Issue. 11,
p.
1931.
Wei, Mingzhu
Duan, Jinlong
Wang, Xu
and
Zhou, Jifu
2023.
Motion of a solid particle in an ore-lifting riser with transverse vibrations.
Physics of Fluids,
Vol. 35,
Issue. 11,
Chen, Gaofeng
and
Jiang, Xikai
2023.
Single-particle dynamics in a low-Reynolds-number fluid under spherical confinement.
Journal of Fluid Mechanics,
Vol. 969,
Issue. ,
Wang, Ping
Lei, Yinghaonan
Zhu, Zhengping
and
Zheng, Xiaojing
2023.
Drag model of finite-sized particle in turbulent wall-bound flow over sediment bed.
Journal of Fluid Mechanics,
Vol. 964,
Issue. ,
de Timary, Guillaume
Cappello, Jean
and
Scheid, Benoit
2023.
Enhanced pinch flow fractionation using inertial streamline crossing.
Microfluidics and Nanofluidics,
Vol. 27,
Issue. 1,
Lauricella, Giuseppe
Naderi, Mohammad Moein
Zhou, Jian
Papautsky, Ian
and
Peng, Zhangli
2024.
Bifurcation of equilibrium positions for ellipsoidal particles in inertial shear flows between two walls.
Journal of Fluid Mechanics,
Vol. 984,
Issue. ,
Lei, Yinghaonan
and
Wang, Ping
2024.
Proceedings of the IUTAM Symposium on Turbulent Structure and Particles-Turbulence Interaction.
Vol. 41,
Issue. ,
p.
265.
Anyaduba, Tochukwu Dubem
and
Rodriguez-Manzano, Jesus
2024.
Offsetting Dense Particle Sedimentation in Microfluidic Systems.
Micromachines,
Vol. 15,
Issue. 9,
p.
1063.
Rastello, Marie
Marié, Jean-Louis
and
Karan Venayagamoorthy, S
2024.
Initiation of motion for an isolated finite size particle in a rotating tank flow.
Fluid Dynamics Research,
Vol. 56,
Issue. 1,
p.
015506.
Gao, Wei
Shi, Pengyu
Parsani, Matteo
and
Costa, Pedro
2024.
On the relevance of lift force modelling in turbulent wall flows with small inertial particles.
Journal of Fluid Mechanics,
Vol. 988,
Issue. ,
Ding, Jiawei
Mei, Dan
Liu, Bowen
Gao, Mingwei
and
Cui, Jiale
2025.
Investigation of the Dynamic Characteristics of PM2.5 Dispersion During the Acceleration of Motor Vehicles in Urban Streets Based on Computational Fluid Dynamics and Dynamic Mode Decomposition.
Atmosphere,
Vol. 16,
Issue. 3,
p.
268.
Cao, Ze
Li, Zhanpeng
Liu, Yakun
Zhang, Di
Tafti, Danesh K.
Wang, Yibo
Yuan, Huiting
and
Hu, Manrong
2025.
Drag, lift, and torque on a near-wall oblate spheroid in linear shear flow.
Physics of Fluids,
Vol. 37,
Issue. 1,
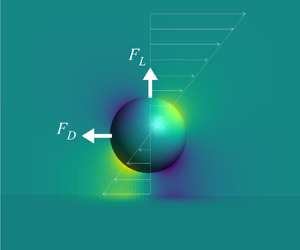
 $10^{-3} \lesssim Re_{\gamma } \lesssim 10^{-1}$). This shear rate range is relevant when dealing with particulate flows within small channels, for example particle migration in microfluidic devices being used or developed for the biotech industry. We demonstrate a strong dependence of both the lift and drag forces on shear rate. Building on previous theoretical
$10^{-3} \lesssim Re_{\gamma } \lesssim 10^{-1}$). This shear rate range is relevant when dealing with particulate flows within small channels, for example particle migration in microfluidic devices being used or developed for the biotech industry. We demonstrate a strong dependence of both the lift and drag forces on shear rate. Building on previous theoretical  $Re_{\gamma } \ll 1$ studies, a wall-shear-based zero-slip lift correlation is proposed that is applicable when the wall lies both within the inner and outer regions of the disturbed flow. Similarly, we validate an improved wall-shear-based zero-slip drag correlation that more accurately captures the drag force when the particle is close to, but not touching, the wall. Application of the new correlations to predict the movement of a force-free particle shows that the examined shear-based lift force is as important as the previously examined slip-based lift force, highlighting the need to accurately account for shear when predicting the near-wall movement of force-free particles.
$Re_{\gamma } \ll 1$ studies, a wall-shear-based zero-slip lift correlation is proposed that is applicable when the wall lies both within the inner and outer regions of the disturbed flow. Similarly, we validate an improved wall-shear-based zero-slip drag correlation that more accurately captures the drag force when the particle is close to, but not touching, the wall. Application of the new correlations to predict the movement of a force-free particle shows that the examined shear-based lift force is as important as the previously examined slip-based lift force, highlighting the need to accurately account for shear when predicting the near-wall movement of force-free particles.


