Hostname: page-component-586b7cd67f-2brh9 Total loading time: 0 Render date: 2024-12-01T04:06:05.477Z Has data issue: false hasContentIssue false
  • English
  • Français

Libration-induced mean flow in a spherical shell

Published online by Cambridge University Press:  08 February 2013

Alban Sauret  and
Stéphane Le Dizès
Alban Sauret
Affiliation:
Institut de Recherche sur les Phénomènes Hors Équilibre, CNRS, and Aix-Marseille University, 49 rue F. Joliot-Curie, F-13013 Marseille, France
Stéphane Le Dizès*
Affiliation:
Institut de Recherche sur les Phénomènes Hors Équilibre, CNRS, and Aix-Marseille University, 49 rue F. Joliot-Curie, F-13013 Marseille, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We investigate the flow in a spherical shell subject to a time harmonic oscillation of its rotation rate, also called longitudinal libration, when the oscillation frequency is larger than twice the mean rotation rate. In this frequency regime, no inertial waves are directly excited by harmonic forcing. We show, however, that, through nonlinear interactions in the Ekman layers, it can generate a strong mean zonal flow in the interior. An analytical theory is developed using a perturbative approach in the limit of small libration amplitude $\varepsilon $ and small Ekman number 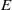$E$. The mean flow is found to be at leading order an azimuthal flow that scales as the square of the libration amplitude and depends only on the cylindrical radius coordinate. The mean flow also exhibits a discontinuity across the cylinder tangent to the inner sphere. We show that this discontinuity can be smoothed through multi-scale Stewartson layers. The mean flow is also found to possess a weak axial flow that scales as 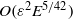$O({\varepsilon }^{2} {E}^{5/ 42} )$ in the Stewartson layers. The analytical solution is compared to axisymmetric numerical simulations, and a good agreement is demonstrated.

JFM classification

Boundary Layers: Boundary Layers Geophysical and Geological Flows: Geophysical and Geological Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 718 , 10 March 2013 , pp. 181 - 209
Copyright
©2013 Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abramowitz, M. & Stegun, I. A. 1965 Handbook of Mathematical Functions. Dover.Google Scholar
Aldridge, K. D. 1967 An experimental study of axisymmetric inertial oscillations of a rotating liquid sphere. PhD thesis, Massachusetts Institute of Technology.Google Scholar
Aldridge, K. D. & Toomre, A. 1969 Axisymmetric inertial oscillations of a fluid in a rotating spherical container. J. Fluid Mech. 37, 307–323.CrossRefGoogle Scholar
Busse, F. H. 1968 Steady fluid flow in a precessing spheroidal shell. J. Fluid Mech. 33, 739–751.CrossRefGoogle Scholar
Busse, F. H. 2010a Mean zonal flows generated by librations of a rotating spherical cavity. J. Fluid Mech. 650, 505–512.CrossRefGoogle Scholar
Busse, F. H. 2010b Zonal flow induced by longitudinal librations of a rotating cylindrical cavity. Physica D 240, 208–211.CrossRefGoogle Scholar
Calkins, M. A., Noir, J., Eldredge, J. & Aurnou, J. M. 2010 Axisymmetric simulations of libration-driven fluid dynamics in a spherical shell geometry. Phys. Fluids 22, 086602.CrossRefGoogle Scholar
Chan, K. H., Liao, X. & Zhang, K. 2011 Simulations of fluid motion in ellipsoidal planetary cores driven by longitudinal libration. Phys. Earth Planet. Inter. 187, 391–403.Google Scholar
Comstock, R. L. & Bills, B. G. 2003 A solar system survey of forced librations in longitude. J. Geophys. Res. 108, 113.Google Scholar
Davis, S. H. 1976 The stability of time-periodic flows. Annu. Rev. Fluid Mech. 8, 57–74.CrossRefGoogle Scholar
Dormy, E. P. & Jault, D. 1998 MHD flow in a slightly differentially rotating spherical shell, with conducting inner core, in a dipolar magnetic field. Earth Planet. Sci. Lett. 160, 15–30.CrossRefGoogle Scholar
Ern, P. & Wesfreid, J. E. 1999 Flow between time-periodically co-rotating cylinders. J. Fluid Mech. 397, 73–98.CrossRefGoogle Scholar
Fischer, D. A., Marcy, G. W., Butler, R. P., Vogt, S. S., Laughlin, G., Henry, G. W., Abouav, D., Peek, K. M. G., Wright, J. T., Johnson, J. A., McCarthy, C. & Isaacson, H. 2008 Five planets orbiting 55 Cancri. Astrophys. J. 675, 790–801.CrossRefGoogle Scholar
Goldreich, P. & Peale, S. 1966 Spin–orbit coupling in the solar system. Astron. J. 397, 73–98.Google Scholar
Hollerbach, R. & Kerswell, R. R. 1995 Oscillatory internal shear layers in rotating and precessing flows. J. Fluid Mech. 298, 327–339.CrossRefGoogle Scholar
Kerswell, R. R. 1995 On the internal shear layers spawned by the critical regions in oscillatory Ekman boundary layers. J. Fluid Mech. 298, 311–325.CrossRefGoogle Scholar
Kida, S. 2011 Steady flow in a rapidly rotating sphere with weak precession. J. Fluid Mech. 680, 150–193.CrossRefGoogle Scholar
Lorenz, R. D., Stiles, B. W., Kirk, R. L., Allison, M. D., Del Marmo, P. P., Iess, L., Lunine, J. I., Ostro, S. J. & Hensley, S. 2008 Titan’s rotation reveals an internal ocean and changing zonal winds. Science 319 (5870), 1649–1651.CrossRefGoogle ScholarPubMed
Margot, J. L., Peale, S. J., Jurgens, R. F., Slade, M. A. & Holin, I. V. 2007 Large amplitude libration of Mercury reveals a molten core. Science 316 (5825), 710–714.CrossRefGoogle ScholarPubMed
Morize, C., Le Bars, M., Le Gal, P. & Tilgner, A. 2010 Experimental determination of zonal winds driven by tides. Phys. Rev. Lett. 104, 214501.CrossRefGoogle ScholarPubMed
Noir, J., Calkins, M. A., Lasbleis, M., Cantwell, J. & Aurnou, J. M. 2010 Experimental study of libration-driven zonal flows in a straight cylinder. Phys. Earth Planet. Inter. 182, 98–106.CrossRefGoogle Scholar
Noir, J., Cébron, D., Le Bars, M., Sauret, A. & Aurnou, J. M. 2012 Experimental study of libration-driven zonal flows in non-axisymmetric containers. Phys. Earth Planet. Inter. 204–205, 1–10.CrossRefGoogle Scholar
Noir, J., Hemmerlin, F., Wicht, J., Baca, S. M. & Aurnou, J. M. 2009 An experimental and numerical study of librationally driven flow in planetary cores and subsurface oceans. Phys. Earth Planet. Inter. 173, 141–152.Google Scholar
Proudman, I. 1956 The almost-rigid rotation of viscous fluid between concentric spheres. J. Fluid Mech. 1, 505–516.CrossRefGoogle Scholar
Rambaux, N., Van Hoolst, T. & Karatekin, Ö. 2011 Librational response of Europa, Ganymede, and Callisto with an ocean for non-Keplerian orbit. Astron. Astrophys. 527, A118.CrossRefGoogle Scholar
Rieutord, M. 1991 Linear theory of rotating fluids using spherical harmonics – II. Time-periodic flows. Geophys. Astrophys. Fluid Dyn. 59, 185–208.CrossRefGoogle Scholar
Sauret, A., Cébron, D., Le Bars, M. & Le Dizès, S. 2012 Fluid flows in a librating cylinder. Phys. Fluids 24, 026603.Google Scholar
Sauret, A., Cébron, D., Morize, C. & Le Bars, M. 2010 Experimental and numerical study of mean zonal flows generated by librations of a rotating spherical cavity. J. Fluid Mech. 662, 260–268.CrossRefGoogle Scholar
Spohn, T. & Schubert, G. 2003 Oceans in the icy Galilean satellites of Jupiter? Icarus 161 (2), 456–467.CrossRefGoogle Scholar
Stewartson, K. 1966 On almost rigid rotations. Part 2. J. Fluid Mech. 26, 131–144.CrossRefGoogle Scholar
Suess, S. T. 1971 Viscous flow in a deformable rotating container. J. Fluid Mech. 45, 189–201.CrossRefGoogle Scholar
Tilgner, A. 1999 Driven inertial oscillations in spherical shells. Phys. Rev. E 59 (2), 1789–1794.CrossRefGoogle Scholar
Tilgner, A. 2007 Zonal wind driven by inertial modes. Phys. Rev. Lett. 99, 194501.CrossRefGoogle ScholarPubMed
Van Hoolst, T., Rambaux, N., Karatekin, Ö., Dehant, V. & Rivoldini, A. 2008 The librations, shape and icy shell of Europa. Icarus 195 (1), 386–399.CrossRefGoogle Scholar
Wang, C. Y. 1970 Cylindrical tank of fluid oscillating about a steady rotation. J. Fluid Mech. 41, 581–592.CrossRefGoogle Scholar
Wieczorek, M. A., Correia, A. C. M., Le Feuvre, M., Laskar, J. & Rambaux, N. 2012 Mercury’s spin–orbit resonance explained by initial retrograde and subsequent synchronous rotation. Nat. Geosci. 5, 18–21.CrossRefGoogle Scholar
Zhang, K., Chan, K. H. & Liao, X. 2011 On fluid motion in librating ellipsoids with moderate equatorial eccentricity. J. Fluid Mech. 673, 468–479.CrossRefGoogle Scholar