Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Xu, Hao
Wei, Yingjie
Wang, Cong
and
Lu, Jiaxing
2019.
On wake vortex encounter of axial-symmetric projectiles launched successively underwater.
Ocean Engineering,
Vol. 189,
Issue. ,
p.
106382.
Posa, Antonio
and
Balaras, Elias
2020.
A numerical investigation about the effects of Reynolds number on the flow around an appended axisymmetric body of revolution.
Journal of Fluid Mechanics,
Vol. 884,
Issue. ,
Manovski, Peter
Jones, Malcolm B
Henbest, Simon M
Xue, Yunpeng
Giacobello, Matteo
and
de Silva, Charitha
2020.
Boundary layer measurements over a body of revolution using long-distance particle image velocimetry.
International Journal of Heat and Fluid Flow,
Vol. 83,
Issue. ,
p.
108591.
Broglia, Riccardo
Posa, Antonio
and
Bettle, Mark C.
2020.
Analysis of vortices shed by a notional submarine model in steady drift and pitch advancement.
Ocean Engineering,
Vol. 218,
Issue. ,
p.
108236.
Zhou, Di
Wang, Kan
and
Wang, Meng
2020.
Large-Eddy Simulation of an Axisymmetric Boundary Layer on a Body of Revolution.
Xu, H
and
Wei, Y J
2020.
Numerical study on hydroballistic and flow field characteristics of projectiles ejected from a vertical launch system underwater.
Journal of Physics: Conference Series,
Vol. 1507,
Issue. 8,
p.
082034.
Ortiz-Tarin, J.L.
Nidhan, S.
and
Sarkar, S.
2021.
High-Reynolds-number wake of a slender body.
Journal of Fluid Mechanics,
Vol. 918,
Issue. ,
Qu, Yi
Wu, Qin
Zhao, Xin
Huang, Biao
Fu, Xiaoying
and
Wang, Guoyu
2021.
Numerical investigation of flow structures around the DARPA SUBOFF model.
Ocean Engineering,
Vol. 239,
Issue. ,
p.
109866.
Morse, Nicholas
and
Mahesh, Krishnan
2021.
Large-eddy simulation and streamline coordinate analysis of flow over an axisymmetric hull.
Journal of Fluid Mechanics,
Vol. 926,
Issue. ,
Liu, Jingcheng
Chu, Xuesen
and
Zhang, Yongming
2021.
Numerical investigation of natural transitions of bow boundary layers over underwater axisymmetric bodies.
Physics of Fluids,
Vol. 33,
Issue. 7,
Szoke, Máté
Nurani Hari, Nandita
Devenport, William J.
Glegg, Stewart A.
and
Teschner, Tom-Robin
2021.
Flow Field Analysis Around Pressure Shielding Structures.
Balantrapu, N. Agastya
Hickling, Christopher
Alexander, W. Nathan
and
Devenport, William
2021.
The structure of a highly decelerated axisymmetric turbulent boundary layer.
Journal of Fluid Mechanics,
Vol. 929,
Issue. ,
Liu, Yi
Zhou, Zhiteng
Zhu, Lixing
and
Wang, Shizhao
2021.
Numerical investigation of flows around an axisymmetric body of revolution by using Reynolds-stress model based hybrid Reynolds-averaged Navier–Stokes/large eddy simulation.
Physics of Fluids,
Vol. 33,
Issue. 8,
Rocca, Andrea
Cianferra, Marta
Broglia, Riccardo
and
Armenio, Vincenzo
2022.
Computational hydroacoustic analysis of the BB2 submarine using the advective Ffowcs Williams and Hawkings equation with Wall-Modeled LES.
Applied Ocean Research,
Vol. 129,
Issue. ,
p.
103360.
Huang, Fenglai
Meng, Qingjie
Cao, Liushuai
and
Wan, Decheng
2022.
Wakes and free surface signatures of a generic submarine in the homogeneous and linearly stratified fluid.
Ocean Engineering,
Vol. 250,
Issue. ,
p.
111062.
Tian, Guizhong
Shi, Jin
Hu, Yushen
Zhou, Honggen
and
Feng, Xiaoming
2022.
Numerical-Experimental Study on the Influence of the Biomimetic Spine-Covered Protrusions (BSCPs) Structure on the Base Pressure and Near-Wake Flow of Underwater Vehicles.
Arabian Journal for Science and Engineering,
Vol. 47,
Issue. 6,
p.
6821.
Gao, Shan
Shi, Yao
Pan, Guang
and
Quan, Xiaobo
2022.
The transient vortex structure in the wake of an axial-symmetric projectile launched underwater.
Physics of Fluids,
Vol. 34,
Issue. 6,
Jiang, Yichen
Li, Yongkun
Wu, Chongjian
Qing, Wang
and
Zhang, Guiyong
2022.
Assessment of RANS and DES turbulence models for the underwater vehicle wake flow field and propeller excitation force.
Journal of Marine Science and Technology,
Vol. 27,
Issue. 1,
p.
226.
Zhou, Zhideng
Li, Zhaobin
He, Guowei
and
Yang, Xiaolei
2022.
Towards multi-fidelity simulation of flows around an underwater vehicle with appendages and propeller.
Theoretical and Applied Mechanics Letters,
Vol. 12,
Issue. 1,
p.
100318.
Zhou, Zhi-teng
Xu, Zhao-yue
Wang, Shi-zhao
and
He, Guo-wei
2022.
Wall-modeled large-eddy simulation of noise generated by turbulence around an appended axisymmetric body of revolution.
Journal of Hydrodynamics,
Vol. 34,
Issue. 4,
p.
533.
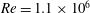 $Re=1.1\times 10^{6}$, based on the free-stream velocity and the length of the body. The geometry used in the present work is an idealized submarine hull (DARPA SUBOFF without appendages) at zero angle of pitch and yaw. The computational domain is chosen to avoid confinement effects and capture the wake up to fifteen diameters downstream of the body. The unstructured computational grid is designed to capture the fine near-wall flow structures as well as the wake evolution. LES results show good agreement with the available experimental data. The axisymmetric turbulent boundary layer has higher skin friction and higher radial decay of turbulence away from the wall, compared to a planar turbulent boundary layer under similar conditions. The mean streamwise velocity exhibits self-similarity, but the turbulent intensities are not self-similar over the length of the simulated wake, consistent with previous studies reported in the literature. The axisymmetric wake shifts from high-
$Re=1.1\times 10^{6}$, based on the free-stream velocity and the length of the body. The geometry used in the present work is an idealized submarine hull (DARPA SUBOFF without appendages) at zero angle of pitch and yaw. The computational domain is chosen to avoid confinement effects and capture the wake up to fifteen diameters downstream of the body. The unstructured computational grid is designed to capture the fine near-wall flow structures as well as the wake evolution. LES results show good agreement with the available experimental data. The axisymmetric turbulent boundary layer has higher skin friction and higher radial decay of turbulence away from the wall, compared to a planar turbulent boundary layer under similar conditions. The mean streamwise velocity exhibits self-similarity, but the turbulent intensities are not self-similar over the length of the simulated wake, consistent with previous studies reported in the literature. The axisymmetric wake shifts from high-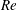 $Re$ to low-
$Re$ to low-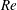 $Re$ equilibrium self-similar solutions, which were only observed for axisymmetric wakes of bluff bodies in the past.
$Re$ equilibrium self-similar solutions, which were only observed for axisymmetric wakes of bluff bodies in the past.

