Hostname: page-component-586b7cd67f-dlnhk Total loading time: 0 Render date: 2024-11-28T09:08:13.941Z Has data issue: false hasContentIssue false
  • English
  • Français

Laminar and transitional liquid metal duct flow near a magnetic point dipole

Published online by Cambridge University Press:  28 October 2013

Saskia Tympel ,
Thomas Boeck  and
Jörg Schumacher
Saskia Tympel*
Affiliation:
Institut für Thermo- und Fluiddynamik, Technische Universität Ilmenau, Postfach 100565, D-98684 Ilmenau, Germany
Thomas Boeck
Affiliation:
Institut für Thermo- und Fluiddynamik, Technische Universität Ilmenau, Postfach 100565, D-98684 Ilmenau, Germany
Jörg Schumacher
Affiliation:
Institut für Thermo- und Fluiddynamik, Technische Universität Ilmenau, Postfach 100565, D-98684 Ilmenau, Germany
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The flow transformation and the generation of vortex structures by a strong magnetic dipole field in a liquid metal duct flow is studied by means of three-dimensional direct numerical simulations. The dipole is considered as the paradigm for a magnetic obstacle which will deviate the streamlines due to Lorentz forces acting on the fluid elements. The duct is of square cross-section. The dipole is located above the top wall and is centred in spanwise direction. Our model uses the quasistatic approximation which is applicable in the limit of small magnetic Reynolds numbers. The analysis covers the stationary flow regime at small hydrodynamic Reynolds numbers 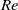$\mathit{Re}$ as well as the transitional time-dependent regime at higher values which may generate a turbulent flow in the wake of the magnetic obstacle. We present a systematic study of these two basic flow regimes and their dependence on 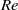$\mathit{Re}$ and on the Hartmann number 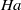$\mathit{Ha}$, a measure of the strength of the magnetic dipole field. Furthermore, three orientations of the dipole are compared: streamwise-, spanwise- and wall-normal-oriented dipole axes. The most efficient generation of turbulence at a fixed distance above the duct follows for the spanwise orientation, which is caused by a certain configuration of Hartmann layers and reversed flow at the top plate. The enstrophy in the turbulent wake grows linearly with 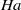$\mathit{Ha}$ which is connected with a dominance of the wall-normal derivative of the streamwise velocity.

JFM classification

MHD and Electrohydrodynamics: MHD and Electrohydrodynamics Instability: Transition to turbulence Vortex Flows: Vortex shedding
Type
Papers
Information
Journal of Fluid Mechanics , Volume 735 , 25 November 2013 , pp. 553 - 586
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Adams, J. C., Swarztrauber, P. & Sweet, R. 1999 Efficient Fortran subprograms for the solution of separable elliptic partial differential equations. http://www.cisl.ucar.edu/css/software/fishpack/.Google Scholar
Airiau, C. & Castets, M. 2004 On the amplification of small disturbances in a channel flow with normal magnetic field. Phys. Fluids 16 (8), 082991.Google Scholar
Alboussiére, T. 2001 Quasi characteristic MHD flows. C. R. Acad. Sci. Paris. Ser. IIB – Mechanics 329 (10), 767–773.Google Scholar
Alboussiére, T., Garandet, J. P. & Moreau, R. 1996 Asymptotic analysis and symmetry in MHD convection. Phys. Fluids 8 (8), 082215.CrossRefGoogle Scholar
Albrecht, T., Grundmann, R., Mutschke, G. & Gerbeth, G. 2006 On the stability of the boundary layer subject to a wall-parallel Lorentz force. Phys. Fluids 18 (9), 098103.CrossRefGoogle Scholar
Andreev, O., Kolesnikov, Y. & Thess, A. 2006 Experimental study of liquid metal channel flow under the influence of a non-uniform magnetic field. Phys. Fluids 18 (6), 065108.Google Scholar
Andreev, O., Kolesnikov, Y. & Thess, A. 2009 Application of the ultrasonic velocity profile method to the mapping of liquid metal flows under the influence of a non-uniform magnetic field. Exp. Fluids 46, 77–83.Google Scholar
Barkley, D., Blackburn, H. M. & Sherwin, S. J. 2008 Direct optimal growth analysis for time steppers. Intl J. Numer. Meth. Fluids 57, 1435–1458.Google Scholar
Biskamp, D. 1993 Nonlinear Magnetohydrodynamics. Cambridge University Press.Google Scholar
Boeck, T., Krasnov, D. & Zienicke, E. 2007 Numerical study of turbulent magnetohydrodynamic channel flow. J. Fluid Mech. 572, 179–188.CrossRefGoogle Scholar
Chaudhary, R., Vanka, S. P. & Thomas, B. G. 2010 Direct numerical simulations of magnetic fields on turbulent flow in a square duct. Phys. Fluids 22 (7), 075102.CrossRefGoogle Scholar
Cuevas, S., Smolentsev, S. & Abdou, M. A. 2006a On the flow past a magnetic obstacle. J. Fluid Mech. 553, 227–252.Google Scholar
Cuevas, S., Smolentsev, S. & Abdou, M. A. 2006b Vorticity generation in creeping flow past a magnetic obstacle. Phys. Rev. E 74 (5), 1–10.CrossRefGoogle Scholar
Davidson, P. A. 1999 Magnetohydrodynamics in material processing. Annu. Rev. Fluid Mech. 31, 273–300.CrossRefGoogle Scholar
Davidson, P. A. 2004 Turbulence: An Introduction for Scientists and Engineers. Oxford University Press.Google Scholar
Davidson, P. A. (Ed.) 2006 An Introduction to Magnetohydrodynamics. Cambridge University Press.Google Scholar
Dousset, V. & Pothérat, A. 2008 Numerical simulations of a cylinder wake under a strong axial magnetic field. Phys. Fluids 20 (1), 017104.Google Scholar
Gavrilakis, S. 1992 Numerical simulation of low-Reynolds-number turbulent flow through a straight square duct. J. Fluid Mech. 244, 101–129.Google Scholar
Gerard-Varet, D. 2002 Amplification of small perturbations in a Hartmann layer. Phys. Fluids 14 (4), 1458–1467.CrossRefGoogle Scholar
Giannetti, F. & Luchini, P. 2007 Structural sensitivity of the instability of the cylinder wake. J. Fluid Mech. 581, 167–197.Google Scholar
Hartmann, J. 1937 Hg-dynamics I. Theory of the laminar flow of an electrically conductive liquid in a homogeneous magnetic field. K. Dan. Vidensk. Selsk. Mat. Fys. Medd. 15 (6), 1–28.Google Scholar
Hartmann, J. & Lazarus, F. 1937 Hg-dynamics II. Experimental investigations on the flow of mercury in a homogeneous magnetic field. K. Dan. Vidensk. Selsk. Mat. Fys. Medd. 15 (7), 1–45.Google Scholar
Heinicke, C. 2013 Spatially resolved measurements in a liquid metal OW with Lorentz force velocimetry. Exp. Fluids 54 (6) 1560, 1–8.Google Scholar
Heinicke, C., Tympel, S., Pulugundla, G., Rahneberg, I., Boeck, T. & Thess, A. 2012 Interaction of a small permanent magnet with a liquid metal duct flow. J. Appl. Phys. 112 (12), 124914.Google Scholar
Huser, A. & Biringen, S. 1993 Direct numerical simulation of turbulent flow in a square duct. J. Fluid Mech. 257, 65–95.Google Scholar
Jackson, J. D. 1998 Classical Electrodynamics, 3rd edn. Wiley.Google Scholar
Jeong, J. & Hussain, F. 1995 On the identification of a vortex. J. Fluid Mech. 285, 69–94.Google Scholar
Jeong, J., Hussain, F., Schoppa, W. & Kim, J. 1997 Coherent structures near the wall in a turbulent channel flow. J. Fluid Mech. 332, 185–214.Google Scholar
Kenjeres, S. 2012 Energy spectra and turbulence generation in the wake of magnetic obstacle. Phys. Fluids 24 (11), 115111.Google Scholar
Knaepen, B. & Moreau, R. 2008 Magnetohydrodynamic turbulence at low magnetic Reynolds number. Annu. Rev. Fluid Mech. 40, 25–45.Google Scholar
Kobayashi, H. 2008 Large eddy simulation of magnetohydrodynamic turbulent duct flows. Phys. Fluids 20 (1), 015102.CrossRefGoogle Scholar
Krasnov, D., Thess, A., Boeck, T., Zhao, Y. & Zikanov, O. 2013 Patterned turbulence in liquid metal flow: computational reconstruction of the Hartmann experiment. Phys. Rev. Lett. 110 (8), 084501.Google Scholar
Krasnov, D., Zikanov, O. & Boeck, T. 2011 Comparative study of finite difference approaches in simulation of magnetohydrodynamic turbulence at low magnetic Reynolds number. Comput. Fluids 50 (1), 46–59.Google Scholar
Krasnov, D., Zikanov, O. & Boeck, T. 2012 Numerical study of magnetohydrodynamic duct flow at high Reynolds and Hartmann numbers. J. Fluid Mech. 704, 421–446.Google Scholar
Krasnov, D., Zikanov, O., Rossi, M. & Boeck, T. 2010 Optimal linear growth in magnetohydrodynamic duct flow. J. Fluid Mech. 653, 273–299.Google Scholar
Krasnov, D. S., Zienicke, E., Zikanov, O., Boeck, T. & Thess, A. 2004 Numerical study of the instability of the Hartmann layer. J. Fluid Mech. 504 (2004), 183–211.Google Scholar
Kulikovskii, A. G. 1968 Slow steady flow of a conducting liquid at large Hartmann numbers. Izv. Akad. Nauk SSSR Mekh. Zhidk. Gaza 3 (2), 3–10.Google Scholar
Mistrangelo, C. 2011 Topological analysis of separation phenomena in liquid metal flow in sudden expansions. Part 2. Magnetohydrodynamic flow. J. Fluid Mech. 674, 132–162.CrossRefGoogle Scholar
Moreau, R. J. 1990 Magnetohydrodynamics. Kluwer.CrossRefGoogle Scholar
Morinishi, Y., Lund, T. S., Vasilyev, O. V. & Moin, P. 1998 Fully conservative higher-order finite difference scheme for incompressible flow. J. Comput. Phys. 143 (1), 90–124.Google Scholar
Müller, U. & Bühler, L. 2001 Magnetohydrodynamics in Channels and Containers. Springer.CrossRefGoogle Scholar
Ni, M.-J., Munipalli, R., Morley, N. B., Huang, P. & Abdou, M. A. 2007 A current density conservative scheme for incompressible MHD flows at low magnetic Reynolds number. Part I. On a rectangular collocated grid system. J. Comput. Phys. 227, 174–204.Google Scholar
Niu, K. 1989 Nuclear Fusion. Cambridge University Press.Google Scholar
Nordström, J., Nordin, N. & Henningson, D. 1999 The fringe region technique and the Fourier method used in the direct numerical simulation of spatially evolving viscous flows. SIAM J. Sci. Comput. 20 (4), 1365–1393.Google Scholar
Pozrikidis, C. (Ed.) 1997 Introduction to Theoretical and Computational Fluid Dynamics. Oxford University Press.Google Scholar
Priede, J., Buchenau, D. & Gerbeth, G. 2011 Single-magnet rotary flowmeter for liquid metals. J. Appl. Phys. 110 (3), 034512.Google Scholar
Rüdiger, G. & Hollerbach, R. 2004 The Magnetic Universe: Geophysical and Astrophysical Dynamo Theory. Wiley-VCH.CrossRefGoogle Scholar
Shatrov, V. & Gerbeth, G. 2010 Marginal turbulent magnetohydrodynamic flow in a square duct. Phys. Fluids 8 (5), 084101.Google Scholar
Shercliff, J. A. (Ed.) 1962 The Theory of Electromagnetic Flow Measurement. Cambridge University Press.Google Scholar
Shercliff, J. A. (Ed.) 1965 A Textbook of Magnetohydrodynamics. Pergamon.Google Scholar
Simens, M. P., Jiménez, J., Hoyas, S. & Mizuno, Y. 2009 A high-resolution code for turbulent boundary layers. J. Comput. Phys. 228, 4218–4231.Google Scholar
Stefani, F., Gundrum, T. & Gerbeth, G. 2004 Contactless inductive flow tomography. Phys. Rev. E 70 (5), 056306.CrossRefGoogle ScholarPubMed
Thess, A., Votyakov, E., Knaepen, B. & Zikanov, O. 2007 Theory of the Lorentz force flowmeter. New J. Phys. 9 (8) 299, 1–27.CrossRefGoogle Scholar
Thess, A., Votyakov, E. & Kolesnikov, Y. 2006 Lorentz force velocimetry. Phys. Rev. Lett. 96 (16), 164501.Google Scholar
Tympel, S. 2013 Magnetohydrodynamic duct flow in the presence of a magnetic dipole. PhD thesis, Ilmenau University of Tehcnology, Germany, http://www.db-thueringen.de/servlets/DocumentServlet?id=22327.Google Scholar
Tympel, S., Krasnov, D., Boeck, T. & Schumacher, J. 2012 Distortion of liquid metal flow in a square duct due to the influence of a magnetic point dipole. Proc. Appl. Maths Mech. 12 (1), 567–568.Google Scholar
Uhlmann, M. & Nagata, M. 2006 Linear stability of flow in an internally heated rectangular duct. J. Fluid Mech. 551, 387–404.Google Scholar
Vantieghem, S., Albets-Chico, X. & Knaepen, B. 2009 The velocity profile of laminar MHD flows in circular conducting pipes. Theor. Comput. Fluid Dyn. 23 (6), 525–533.Google Scholar
Votyakov, E., Kolesnikov, Y., Andreev, O., Zienicke, E. & Thess, A. 2007 Structure of the wake of a magnetic obstacle. Phys. Rev. Lett. 98 (14), 144504.Google Scholar
Votyakov, E., Zienicke, E. & Kolesnikov, Y. 2008 Constrained flow around a magnetic obstacle. J. Fluid Mech. 610, 131–156.CrossRefGoogle Scholar
Williamson, C. K. H. 1996 Vortex dynamics in the cylinder wake. Annu. Rev. Fluid Mech. 28, 477–539.Google Scholar
Zhao, Y. & Zikanov, O. 2012 Instabilities and turbulence in magnetohydrodynamic flow in a toroidal duct prior to transition in Hartmann layers. J. Fluid Mech. 692, 288–316.CrossRefGoogle Scholar