Hostname: page-component-78c5997874-8bhkd Total loading time: 0 Render date: 2024-11-17T14:15:54.136Z Has data issue: false hasContentIssue false
  • English
  • Français

Laboratory studies of Lagrangian transport by breaking surface waves

Published online by Cambridge University Press:  01 August 2019

Luc Lenain Open the ORCID record for Luc Lenain [Opens in a new window] ,
Nick Pizzo Open the ORCID record for Nick Pizzo [Opens in a new window]  and
W. Kendall Melville
Luc Lenain*
Affiliation:
Scripps Institution of Oceanography, University of California San Diego, La Jolla, CA 92037, USA
Nick Pizzo
Affiliation:
Scripps Institution of Oceanography, University of California San Diego, La Jolla, CA 92037, USA
W. Kendall Melville
Affiliation:
Scripps Institution of Oceanography, University of California San Diego, La Jolla, CA 92037, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

While it has long been recognized that Lagrangian drift at the ocean surface plays a critical role in the kinematics and dynamics of upper ocean processes, only recently has the contribution of wave breaking to this drift begun to be investigated through direct numerical simulations (Deike et al., J. Fluid Mech., vol. 829, 2017, pp. 364–391; Pizzo et al., J. Phys. Oceanogr., vol. 49(4), 2019, pp. 983–992). In this work, laboratory measurements of the surface Lagrangian transport due to focusing deep-water non-breaking and breaking waves are presented. It is found that wave breaking greatly enhances mass transport, compared to non-breaking focusing wave packets. These results are in agreement with the direct numerical simulations of Deike et al. (J. Fluid Mech., vol. 829, 2017, pp. 364–391), and the increased transport due to breaking agrees with their scaling argument. 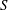In particular, the transport at the surface scales with $S$, the linear prediction of the maximum slope at focusing, while the surface transport due to non-breaking waves scales with 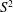$S^{2}$, in agreement with the classical Stokes prediction.

JFM classification

Geophysical and Geological Flows: Air/sea interactions Waves/Free-surface Flows: Surface gravity waves Waves/Free-surface Flows: Wave breaking
Type
JFM Rapids
Information
Journal of Fluid Mechanics , Volume 876 , 10 October 2019 , R1
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Banner, M. L. & Peirson, W. L. 2007 Wave breaking onset and strength for two-dimensional deep-water wave groups. J. Fluid Mech. 585 (1), 93–115.Google Scholar
Batchelor, G. K. 1967 An Introduction to Fluid Dynamics. Cambridge University Press.Google Scholar
van den Bremer, T. S. & Taylor, P. H. 2016 Lagrangian transport for two-dimensional deep-water surface gravity wave groups. Proc. R. Soc. Lond. A 472 (2192), 20160159.Google Scholar
Cavaleri, L., Fox-Kemper, B. & Hemer, M. 2012 Wind waves in the coupled climate system. Bull. Am. Meteorol. Soc. 93 (11), 1651–1661.Google Scholar
Craik, A. D. & Leibovich, S. 1976 A rational model for Langmuir circulations. J. Fluid Mech. 73 (03), 401–426.Google Scholar
Deike, L., Pizzo, N. E. & Melville, W. K. 2017 Lagrangian transport by breaking surface waves. J. Fluid Mech. 829, 364–391.Google Scholar
Deike, L., Popinet, S. & Melville, W. K. 2015 Capillary effects on wave breaking. J. Fluid Mech. 769, 541–569.Google Scholar
Drazen, D. A. & Melville, W. K. 2009 Turbulence and mixing in unsteady breaking surface waves. J. Fluid Mech. 628, 85–119.Google Scholar
Drazen, D. A., Melville, W. K. & Lenain, L. 2008 Inertial scaling of dissipation in unsteady breaking waves. J. Fluid Mech. 611, 307–332.Google Scholar
Grue, J. & Jensen, A. 2012 Orbital velocity and breaking in steep random gravity waves. J. Geophys. Res.: Oceans 117, C07013.Google Scholar
Grue, J. & Kolaas, J. 2017 Experimental particle paths and drift velocity in steep waves at finite water depth. J. Fluid Mech. 810, R1.Google Scholar
Hornung, H. G., Willert, C. & Turner, S. 1995 The flow field downstream of a hydraulic jump. J. Fluid Mech. 287, 299–316.Google Scholar
Kenyon, K. E. 1969 Stokes drift for random gravity waves. J. Geophys. Res. 74 (28), 6991–6994.Google Scholar
Leibovich, S. 1983 The form and dynamics of Langmuir circulations. Annu. Rev. Fluid Mech. 15 (1), 391–427.Google Scholar
Longuet-Higgins, M. S. 1974 Breaking waves in deep or shallow water. In Proceedings of the 10th Conference on Naval Hydrodynamics (Cambridge, MA), pp. 597–605. Office of Naval Research.Google Scholar
Longuet-Higgins, M. S. 1998 Vorticity and curvature at a free surface. J. Fluid Mech. 356, 149–153.Google Scholar
Melling, A. 1997 Tracer particles and seeding for particle image velocimetry. Meas. Sci. Technol. 8 (12), 1406.Google Scholar
Melville, W. K. 1996 The role of surface wave breaking in air-sea interaction. Annu. Rev. Fluid Mech. 28, 279–321.Google Scholar
Melville, W. K., Veron, F. & White, C. J. 2002 The velocity field under breaking waves: coherent structure and turbulence. J. Fluid Mech. 454, 203–233.Google Scholar
Phillips, O. M. 1985 Spectral and statistical properties of the equilibrium range in wind-generated gravity waves. J. Fluid Mech. 156 (1), 505–531.Google Scholar
Pizzo, N. E. 2017 Surfing surface gravity waves. J. Fluid Mech. 823, 316–328.Google Scholar
Pizzo, N. E. & Melville, W. K. 2013 Vortex generation by deep-water breaking waves. J. Fluid Mech. 734, 198–218.Google Scholar
Pizzo, N. E. & Melville, W. K. 2016 Wave modulation: the geometry, kinematics, and dynamics of surface-wave packets. J. Fluid Mech. 803, 275–291.Google Scholar
Pizzo, N. E., Melville, W. K. & Deike, L. 2016 Current generation by deep-water wave breaking. J. Fluid Mech. 803, 292–312.Google Scholar
Pizzo, N. E., Melville, W. K. & Deike, L. 2019 Lagrangian transport by non-breaking and breaking deep-water waves at the ocean surface. J. Phys. Oceanogr. 49 (4), 983–992.Google Scholar
Prasad, A. K. 2000 Particle image velocimetry. Curr. Sci. 79 (1), 51–60.Google Scholar
Rapp, R. J. & Melville, W. K. 1990 Laboratory measurements of deep-water breaking waves. Phil. Trans. R. Soc. Lond. A 735–800.Google Scholar
Romero, L., Melville, W. K. & Kleiss, J. M. 2012 Spectral energy dissipation due to surface wave breaking. J. Phys. Oceanogr. 42 (9), 1421–1444.Google Scholar
Sullivan, P. P., McWilliams, J. C. & Melville, W. K. 2007 Surface gravity wave effects in the oceanic boundary layer: large-eddy simulation with vortex force and stochastic breakers. J. Fluid Mech. 593, 405–452.Google Scholar
Sutherland, P. & Melville, W. K. 2013 Field measurements and scaling of ocean surface wave-breaking statistics. Geophys. Res. Lett. 40 (12), 3074–3079.Google Scholar
Sutherland, P. & Melville, W. K. 2015 Field measurements of surface and near-surface turbulence in the presence of breaking waves. J. Phys. Oceanogr. 45 (4), 943–965.Google Scholar
Tian, Z., Perlin, M. & Choi, W. 2010 Energy dissipation in two-dimensional unsteady plunging breakers and an eddy viscosity model. J. Fluid Mech. 655, 217–257.Google Scholar