Article contents
Kármán–Howarth solutions of homogeneous isotropic turbulence
Published online by Cambridge University Press: 07 December 2021
Abstract
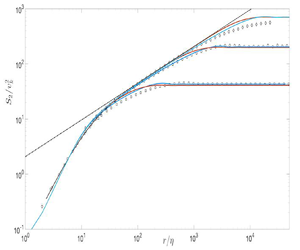
The Kármán–Howarth equation (KHEq) is solved using a closure model to obtain solutions of the second-order moment of the velocity increment,  $S_2$, in homogeneous isotropic turbulence (HIT). The results are in good agreement with experimental data for decaying turbulence and are also consistent with calculations based on the three-dimensional energy spectrum for decaying HIT. They differ, however, from those for forced HIT, the difference occurring mainly at large scales. This difference is attributed to the fact that the forcing generates large-scale motions which are not compatible with the KHEq. As the Reynolds number increases, the impact of forcing on the small scales decreases, thus allowing the KHEq and spectrally based solutions to agree well in the range of scales unaffected by forcing. Finally, the results show that the two-thirds law is compatible with the KHEq solutions as the Reynolds number increases to very large, if not infinite, values.
$S_2$, in homogeneous isotropic turbulence (HIT). The results are in good agreement with experimental data for decaying turbulence and are also consistent with calculations based on the three-dimensional energy spectrum for decaying HIT. They differ, however, from those for forced HIT, the difference occurring mainly at large scales. This difference is attributed to the fact that the forcing generates large-scale motions which are not compatible with the KHEq. As the Reynolds number increases, the impact of forcing on the small scales decreases, thus allowing the KHEq and spectrally based solutions to agree well in the range of scales unaffected by forcing. Finally, the results show that the two-thirds law is compatible with the KHEq solutions as the Reynolds number increases to very large, if not infinite, values.
JFM classification
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press
References
REFERENCES
- 3
- Cited by



